A source S of acoustic wave of the frequency v0=1700Hz and a receiver R are located at the same point. At the instant t=0, the source start from rest to move away from the receiver with a constant acceleration ω. The velocity of sound in air is v=340ms.
Q. If ω=10ms2, the apparent frequency that will be recorded by the stationary receiver at t=10s will be
1700Hz
1.35Hz
850Hz
1.27Hz
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the motion of the source
The source S starts from rest and moves away from the receiver R with a constant acceleration ω=10ms². We need to find the position of the source after t=10seconds.
Hint: Use the kinematic equation for uniformly accelerated motion:
\( d = ut + \frac{1}{2} a t^2 \)
where \( u = 0 \) (initial velocity), \( a = \omega \), and \( t = 10 \, \text{s} \).
Step 2: Calculate the distance traveled by the source
Using the kinematic equation:
\( d = 0 \cdot 10 + \frac{1}{2} \cdot 10 \cdot (10)^2 \)
\( d = \frac{1}{2} \cdot 10 \cdot 100 = 500 \, \text{m} \)
Hint: Remember that the distance traveled is directly proportional to the square of the time when starting from rest.
Step 3: Determine the velocity of the source at t = 10 seconds
The velocity of the source at time t can be calculated using:
\( v = u + at \)
\( v = 0 + 10 \cdot 10 = 100 \, \text{m/s} \)
Hint: The velocity increases linearly with time under constant acceleration.
Step 4: Calculate the time taken for the sound to reach the receiver
Since the source is 500 m away from the receiver at t=10seconds, we need to find out how long it takes for the sound to travel this distance. The speed of sound in air is given as v=340ms.
Using the formula:
\( t_{sound} = \frac{d}{v} = \frac{500}{340} \approx 1.47 \, \text{s} \)
Hint: The time taken for sound to travel a distance is calculated using the formula \( \text{time} = \frac{\text{distance}}{\text{speed}} \).
Step 5: Determine the effective time when the sound was emitted
The sound that reaches the receiver at t=10seconds was emitted at:
\( t_{emit} = 10 - t_{sound} \approx 10 - 1.47 \approx 8.53 \, \text{s} \)
Hint: The sound emitted must account for the time it takes to travel to the receiver.
Step 6: Calculate the velocity of the source at the time of emission
Now, we need to find the velocity of the source at t=8.53seconds:
\( v = u + at = 0 + 10 \cdot 8.53 \approx 85.3 \, \text{m/s} \)
Hint: Use the same kinematic equation as before to find the velocity at a specific time.
Step 7: Apply the Doppler Effect formula
Since the source is moving away from the receiver, we use the Doppler Effect formula:
\( f' = \frac{v}{v + v_s} f_0 \)
Where:
- \( f' \) = apparent frequency
- \( v \) = speed of sound = 340 m/s
- \( v_s \) = speed of source = 85.3 m/s
- \( f_0 \) = original frequency = 1700 Hz
Substituting the values:
\( f' = \frac{340}{340 + 85.3} \cdot 1700 \)
Step 8: Calculate the apparent frequency
Calculating the denominator:
\( 340 + 85.3 = 425.3 \)
Now substituting into the formula:
\( f' = \frac{340}{425.3} \cdot 1700 \approx 0.798 \cdot 1700 \approx 1350 \, \text{Hz} \)
Hint: When calculating the apparent frequency, ensure to multiply the fraction by the original frequency to find the final result.
Final Answer
The apparent frequency recorded by the stationary receiver at \( t = 10 \, \text{s} \) will be approximately 1350 Hz.
The source S starts from rest and moves away from the receiver R with a constant acceleration ω=10ms². We need to find the position of the source after t=10seconds.
\( d = ut + \frac{1}{2} a t^2 \)
where \( u = 0 \) (initial velocity), \( a = \omega \), and \( t = 10 \, \text{s} \).
Using the kinematic equation:
\( d = 0 \cdot 10 + \frac{1}{2} \cdot 10 \cdot (10)^2 \)
\( d = \frac{1}{2} \cdot 10 \cdot 100 = 500 \, \text{m} \)
The velocity of the source at time t can be calculated using:
\( v = u + at \)
\( v = 0 + 10 \cdot 10 = 100 \, \text{m/s} \)
Since the source is 500 m away from the receiver at t=10seconds, we need to find out how long it takes for the sound to travel this distance. The speed of sound in air is given as v=340ms.
\( t_{sound} = \frac{d}{v} = \frac{500}{340} \approx 1.47 \, \text{s} \)
The sound that reaches the receiver at t=10seconds was emitted at:
\( t_{emit} = 10 - t_{sound} \approx 10 - 1.47 \approx 8.53 \, \text{s} \)
Now, we need to find the velocity of the source at t=8.53seconds:
\( v = u + at = 0 + 10 \cdot 8.53 \approx 85.3 \, \text{m/s} \)
Since the source is moving away from the receiver, we use the Doppler Effect formula:
\( f' = \frac{v}{v + v_s} f_0 \)
Where:
- \( f' \) = apparent frequency
- \( v \) = speed of sound = 340 m/s
- \( v_s \) = speed of source = 85.3 m/s
- \( f_0 \) = original frequency = 1700 Hz
\( f' = \frac{340}{340 + 85.3} \cdot 1700 \)
Calculating the denominator:
\( 340 + 85.3 = 425.3 \)
Now substituting into the formula:
\( f' = \frac{340}{425.3} \cdot 1700 \approx 0.798 \cdot 1700 \approx 1350 \, \text{Hz} \)
The apparent frequency recorded by the stationary receiver at \( t = 10 \, \text{s} \) will be approximately 1350 Hz.
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Assertion-Reasoning
Explore 24 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICS ENGLISHChapter:RIGID BODY DYNAMICS 2Exercise:Interger
Explore 2 Videos
- SUPERPOSITION AND STANDING WAVESBook:CENGAGE PHYSICS ENGLISHChapter:SUPERPOSITION AND STANDING WAVESExercise:Comprehension Type
Explore 5 Videos
Similar Questions
A source S of acoustic wave of the frequency v0=1700Hz and a receiver R are located at the same point. At the instant t=0, the source start from rest to move away from the receiver with a constant acceleration ω. The velocity of sound in air is v=340ms.
If ω=10ms2 for 10s and then ω=0 for t>10s, the apparent frequency recorded by the receiver at t=15s
View SolutionA source S of acoustic wave of the frequency v0=1700Hz and a receiver R are located at the same point. At the instant t=0, the source start from rest to move away from the receiver with a constant acceleration ω. The velocity of sound in air is v=340ms.
If ω=10ms2 for 10s and then ω=0 for t>10s, the apparent frequency recorded by the receiver at t=15s
View SolutionA source of sonic oscillations with frequency n=1700Hz and a receiver are located on the same normal to a wall. Both the source and receiver are stationary, and the wall recedes from the source with velocity u=6.0ms. Find the beat frequency registered by the receiver. The velocity of sound is v=340ms.
View SolutionA source of sonic oscillations with frequency n=1700Hz and a receiver are located on the same normal to a wall. Both the source and receiver are stationary, and the wall recedes from the source with velocity u=6.0ms. Find the beat frequency registered by the receiver. The velocity of sound is v=340ms.
View SolutionA source of sound emitting a note of frequency 200 Hz moves towards an observer with a velocity v equal to the velocity of sound. If the observer also moves away from the source with the same velocity v, the apparent frequency heard by the observer is
View SolutionA listener is at rest and a police siren is moving away from the listerner at 60m/s. what frequency does the listerner hear given that the velocity of sound in air is 340m/s and frequency os siren is 500Hz?
View SolutionA source of sound of frequency 300 Hz and a receiver are located along the same line normal to the wall as shown in the figure. Both the source and the receiver are stationary and the wall receeds from the source with velocity 20ms−1.If the beat frequency registered by the receiver is 240x Hz then x is :
(Assume Vsound=300 m/s).

View SolutionA stationary sound sound 's' of frequency 334 Hz and a stationary ovserver 'O' are placed near a reflecting suface moving away from the source with velocity 2 m/s as shown in the figure. If the velocity of the sound waves is air is v= 330 m/s the apparent frequency of the echo is
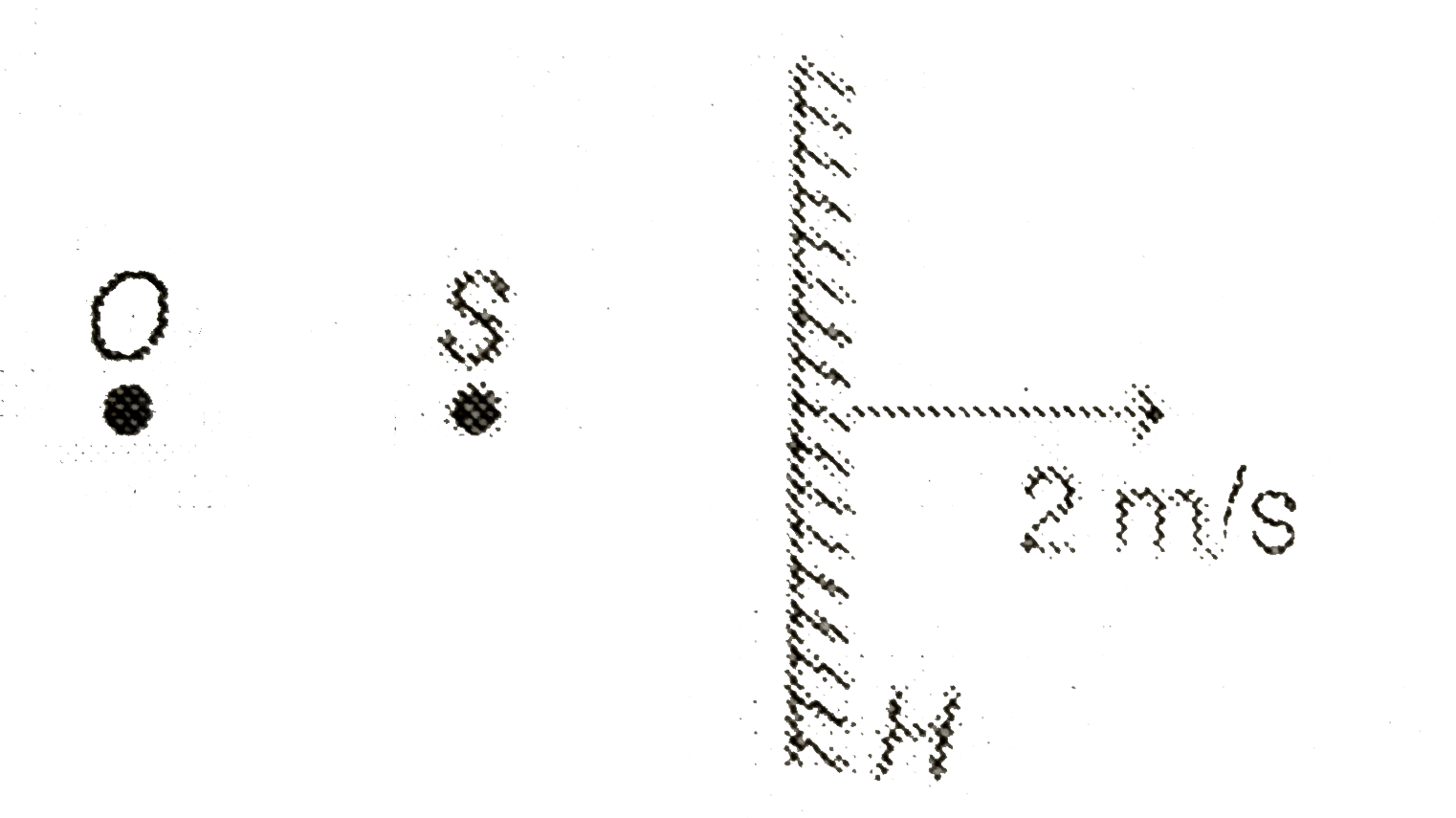
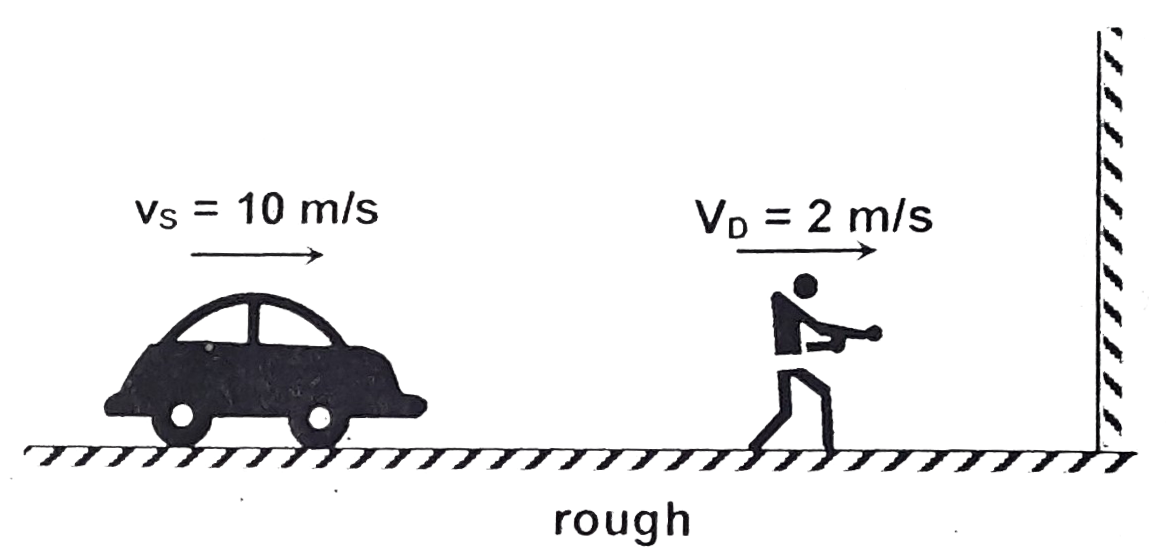
View SolutionA car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
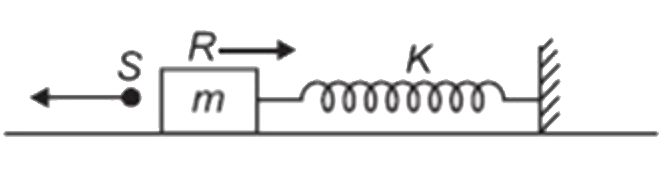
View SolutionA sound source S, emitting a sound of frequency 400 Hz and a receiver R of mass m are at the same point. R is performing SHM with the help of a spring of force constant K. At a time t = 0, R is at the mean position and moving towards the right, as shown. At the same time, the source starts moving away from R with some acceleration a. The frequency registered by the receiver at a time t = 10 s is 250 Hz. What is the time (in seconds) at which the corresponding registered frequency of 250 Hz was emitted by the source? [Given that mK=25π2 and amplitude of oscillation = 100πm,Vsound = 320 m s^(-1)]
View Solution