A stationary source emits sound of frequency
υ=1200Hz. If a wind blows at the speed of 0.1c deduce (i) the percentage change in the wavelength and (ii) the change in the frequency for a stationary observer on the wind side of the source. What happens when there is no wind, but the observer moves at speed 0.1c towards the source?
To solve the problem step by step, we will analyze the effects of wind on sound waves emitted from a stationary source and the effects of an observer moving towards the source.
Given Data:
- Frequency of the sound source, f=1200Hz
- Speed of wind, w=0.1c (where c is the speed of sound)
Step 1: Determine the speed of sound with wind
When wind blows, the effective speed of sound increases. The new speed of sound V′ can be calculated as:
V′=c+w=c+0.1c=1.1c
Step 2: Calculate the original wavelength
The wavelength λ of the sound can be calculated using the formula:
λ=cf
Step 3: Calculate the new wavelength with wind
The new wavelength λ′ when the wind is blowing can be calculated as:
λ′=V′f=1.1cf
Step 4: Calculate the change in wavelength
The change in wavelength Δλ is given by:
Δλ=λ′−λ=1.1cf−cf=0.1cf
Step 5: Calculate the percentage change in wavelength
The percentage change in wavelength can be calculated using the formula:
Percentage Change=(Δλλ×100)=(0.1c/fc/f×100)=10%
Step 6: Change in frequency for a stationary observer
Since the observer is stationary and the source is also stationary, the frequency observed does not change due to the wind. Thus, the change in frequency Δf is:
Δf=0Hz
Step 7: Analyze the case when there is no wind, but the observer moves towards the source
When the observer moves towards the source at speed 0.1c, the apparent frequency f′ can be calculated using the Doppler effect formula:
f′=f(c+voc)
where vo=0.1c.
Step 8: Calculate the apparent frequency
Substituting the values, we get:
f′=1200(c+0.1cc)=1200(1+0.1)=1200×1.1=1320Hz
Step 9: Calculate the change in frequency
The change in frequency when the observer moves towards the source is:
Δf=f′−f=1320−1200=120Hz
Step 10: Calculate the percentage change in frequency
The percentage change in frequency can be calculated as:
Percentage Change=(Δff×100)=(1201200×100)=10%
Final Answers:
1. Percentage change in wavelength: 10%
2. Change in frequency for a stationary observer on the wind side: 0 Hz
3. Change in frequency when the observer moves towards the source: 120 Hz (10% increase)
- Frequency of the sound source, f=1200Hz
- Speed of wind, w=0.1c (where c is the speed of sound)
When wind blows, the effective speed of sound increases. The new speed of sound V′ can be calculated as:
V′=c+w=c+0.1c=1.1c
The wavelength λ of the sound can be calculated using the formula:
λ=cf
The new wavelength λ′ when the wind is blowing can be calculated as:
λ′=V′f=1.1cf
The change in wavelength Δλ is given by:
Δλ=λ′−λ=1.1cf−cf=0.1cf
The percentage change in wavelength can be calculated using the formula:
Percentage Change=(Δλλ×100)=(0.1c/fc/f×100)=10%
Since the observer is stationary and the source is also stationary, the frequency observed does not change due to the wind. Thus, the change in frequency Δf is:
Δf=0Hz
When the observer moves towards the source at speed 0.1c, the apparent frequency f′ can be calculated using the Doppler effect formula:
f′=f(c+voc)
where vo=0.1c.
Substituting the values, we get:
f′=1200(c+0.1cc)=1200(1+0.1)=1200×1.1=1320Hz
The change in frequency when the observer moves towards the source is:
Δf=f′−f=1320−1200=120Hz
The percentage change in frequency can be calculated as:
Percentage Change=(Δff×100)=(1201200×100)=10%
1. Percentage change in wavelength: 10%
2. Change in frequency for a stationary observer on the wind side: 0 Hz
3. Change in frequency when the observer moves towards the source: 120 Hz (10% increase)
Topper's Solved these Questions
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Subjective
56 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Single Correct
252 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Exercise 6.1
56 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Comprehension Type
5 Videos
Similar Questions
A stationary source emits sound of frequency 1200 Hz. If would blows at the speed of 0.1 v. deduce
(a) The change in the frequency for a stationary observer on the wind side of the source
(b) Report the calculations for the case when there is no wind but the observer moves at 0.1 v speed towards the source (Given : velocity of sound = v)
View SolutionShow that when the speed of the source and the observer are small compared to the speed of sound in the medium, the change in frequency becomes independent of the fact whether the source is moving or the observer.
View Solution
Knowledge Check
A source of sound producing wavelength 50 cm is moving away from a stationary observer with (15)th speed of sound. Then what is the wavelength of sound received by the observer?
A55 cmB40 cmC60 cmD70 cmAn observer moves towards a stationary source of sound. The percentage change in the apparent frequency is
AzeroB0.05C0.1D0.2A sound source is moving towards a stationary observer with 1/10 of the speed of sound. The ratio of apparent to real frequency is
A109B(109)2C(1110)2D1110
when a source of sound of frequency f crosses stationary observer with a speed vs(<< speed of sound v) , the apparent change in frequency Δf is given by
View SolutionWhen source of sound moves towards a stationary observer, the wavelength of sound received by him
View Solution(a) Does the change in frequency due to Doppler effect depend on the distance between source (b)Does the change in frequency due to Doppler effect on the fact that source is moving towards the observer or observer is moving towards the source ?
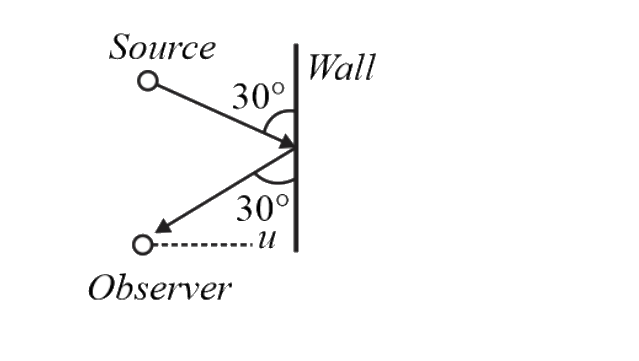
View SolutionA stationary source (see figure) emits sound waves of frequency f towards a stationary wall. If an observer moving with speed u in a direction perpendicular to the wall measures a frequency f'=118f at the instant shown, then u is related to the speed of sound Vs as
View SolutionAn observer starts moving with unifrom acceleration a towards a stationary sound soure of frequency fo. As the observer approaches the source ,the apparent frequency f heard by the observer varies with time t as
View Solution
