A train approachign a railway crossing at a speed of $120 k m h^{- 1}$ sounds a short whistle at frequency 640 Hz when it is 300 m away from the crossing. The speed of sound in air is $340 m s^{- 1}$ . What will be the frequency heard by a person standing on a road perpendicular to the track through the crossing at a distance of 400 m from the crossing ?

Video Solution

Text Solution

Verified by Experts

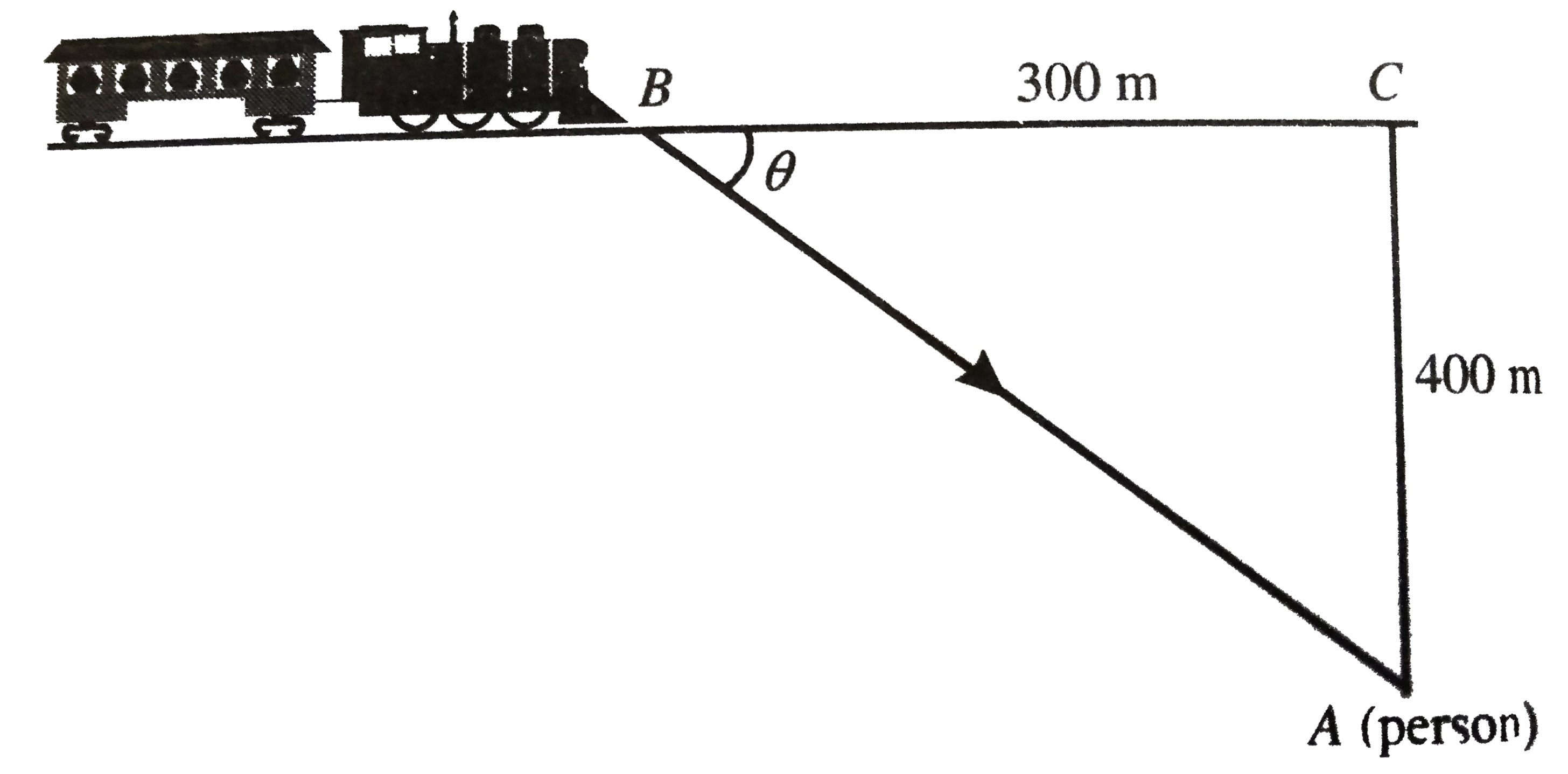
The observer A is at rest with respect to the air and the source is travelling at a velocity of 120 km/s, i.e. $(\frac{100}{3}) \frac{m}{s}$ . As is clear from the figure, the person receives the sound of the whistle in direction BA making an angle $θ$ with the trach where $cos θ = \frac{300}{500} = \frac{3}{5}$ . The component of the velocity of the source (i.e., of the train) along the direction AB is $(\frac{100}{3}) \times (\frac{3}{5}) \frac{m}{s} = 20 \frac{m}{s}$ . As the source is approaching the person with this component, the frequency heard by the observer is $n' = \frac{v}{v - u cos θ} n = \frac{340}{340 - 20} \times 640 H z = 680 H z$

Updated on:21/07/2023

A train approaching a railway platform with a speed of 20 m $s^{- 1}$ starts blowing the whistle. Speed of sound in air is 340 $m s^{- 1}$ . If the frequency of the emitted sound from the whistle is 640 Hz, the frequency of sound as heard by person standing on the platform
A600 Hz
B640 Hz
C680 Hz
D720 Hz