A solenoid of length 0.6 m has a radius of 2 cm and is made up of 600 turns If it carries a current of 4 A, then the magnitude of the magnetic field inside the solenoid is
the solenoid is shown in Fig
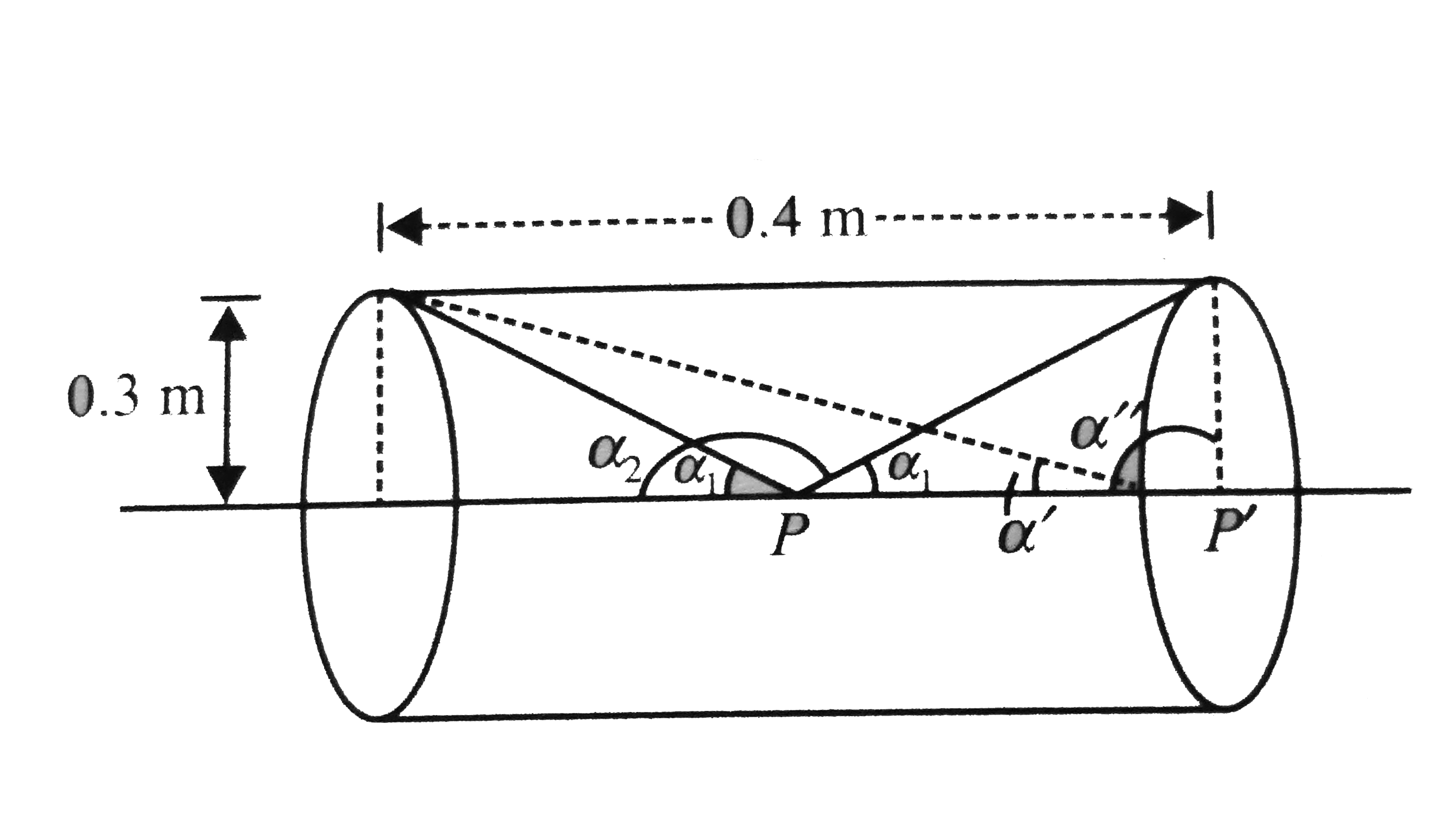 The magnetic field at the middle point P on the axis of a current
The magnetic field at the middle point P on the axis of a current
carrying solenoide is given by
B=μ0ni2(cosα1−cosα2)
where n=number of turns/meter and i=current in the solenoid
Here n=10000.4=2500 per metre and i=5.0×10−3A
cosα2=cos(180−α1)=−cosα1
∴B=μ0ni22cosα1
cosα1=0.2√(0.2)2+(0.3)2=0.2√0.13=0.555
∴B=(4π×10−7)×2500×5.0×10−3×0.555
=2.775π×10−6T
At the end of the solenoid
B=μ0ni2[cosα'−cosα'']
cosα'=0.4√{(0.3)2+(0.4)2}=0.40.5=0.8
cosα''=cos90∘=0
∴B=(4π×10−7)(2500)(5.0×10−3)2×0.8=2π×10−6T
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Solved Example
20 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.1
35 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type
14 Videos
Similar Questions
A solenoid of length 0⋅5m has a radius of 1cm and is made up of 500 turns. It carries a current of 5A. What is the magnitude of the magnetic field inside the solenoid?
View SolutionA solenoid has length 0.4m, radius 1 cm and 400 turns of wire. If a current fo 5 A is passed through this solenoid, then what is the magnetic field inside the solenoid?
View Solution
Knowledge Check
A 90 cm long solenoid has six layers of windings of 450 turns each. If the diameter of solenoid is 2.2 cm and current carried is 6 A, then the magnitude of magnetic field inside the solenoid, near its centre is
A50πGB60πGC72πGD80πG
A thin solenoid of length 0.4m and having 500 turns of wire carries a current 1A,then find the magnetic field on the axis inside the solenoid.
View SolutionThe average radius of an air cored made toroid is 0.1 m and it has 500 turns. If it carries 0.5 ampere current, then the magnetic field inside it is :
View SolutionA solenoid of 2.5 m length and 2.0 cm diameter possesses 10 turns per cm. A current of 0.5 A is flowing through it . The magnetic induction at axis inside the solenoid is
View SolutionA solenoid of length 10 cm and radius 1 cm contains 200 turns and carries a current of 10 A. Find the magnetic field at a point on the axis at a distance of 10cm from the centre.
View SolutionA long solenoid of length L has a mean diameter D. It has n layers of windings of N turns each. If it carries a current ‘i’ the magnetic field at its centre will be
View SolutionA solenoid of length 8cm has 500 turns /m in it. If radius of coil is 3cm and if it is carrying a current of 2A, find the magnetic induction at a point 4cm from the end on the axis of the solenoid
View SolutionA solenoid of 2m long & 3cm diameter has 5 layers of winding of 500 turns per metre length in each layer & carries a current of 5A. Find intensity of magnetic field at the centre of the solenoid.
View Solution
