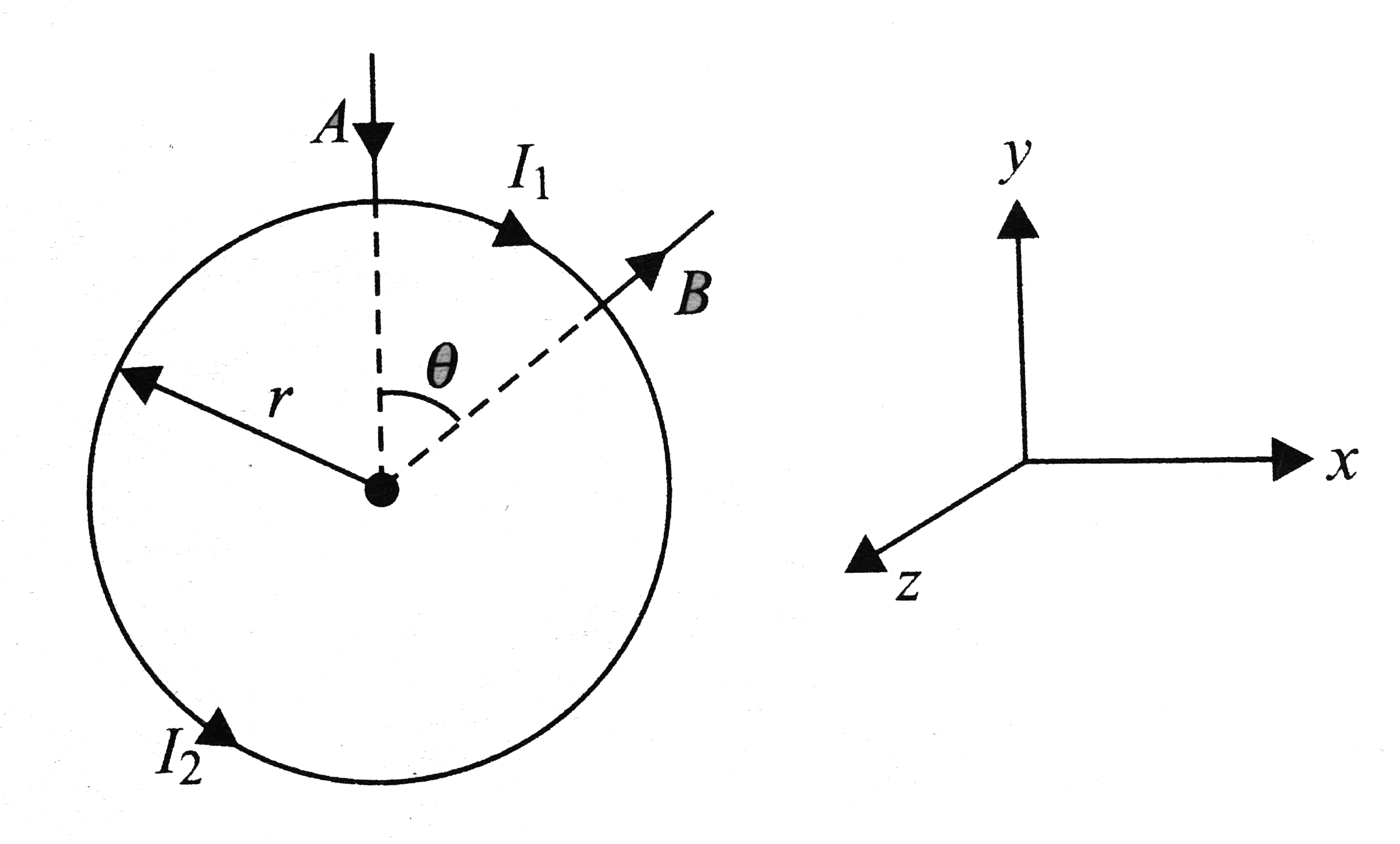
A battery is connected between two points A and B on the circumference of a uniform conducting ring of radius r and resistance R. One of the arc AB of the ring subtends an angle θ at the centre. The value of the magnetic field produced at the centre due to the current in the ring is
Magnetic field at the centre of an arc is given by
B=μ0I2R×θ2π
Megnetic field due to a smaller arc, →B1=μ0I12r×θ2π(−ˆk)
Megnetic field due to a larger arc, →B2=μ0I12r×2π−θ2π(+ˆk)
Resultant magnetic field =[−μ0I1θ4πr+μ0I(2π−θ)4πr](ˆk)...(i)
Two arcs form a parallel combination of resistors.
Here l1R1=i1R2⇒I1I2=R2R1....(ii)
Here R1andR2 are the resistances of smaller and bigger arcs,
respectively.
But R2R1=l2l1=(2π−θ)rθr=2π−θθ.....(iii)
From Eqs. (ii) and (iii), we get
I1θ=I2(2π−θ).....(iv)
Hence, from Eqs. (i) and (iv), the magnetic field at the centre
of ring will be zero.
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Solved Example
20 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.1
35 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Comprehension Type
14 Videos
Similar Questions
A battery is connected between two points AandB on the circumference of a uniform conducting ring of radius r and resistance R . One of the arcs AB of the ring subtends an angle θ at the centre . The value of the magnetic induction at the centre due to the current in the ring is
View SolutionAn arc of a circle of raduis R subtends an angle π2 at the centre. It carriers a current i. The magnetic field at the centre will be
View SolutionA current - carrying wire of certain length is bent to form an arc that substends an angle θ at the centre. Let B be the magnetic field at the centre due to this wire. The correct graph between B and θ is
View SolutionFind the centre of mass of a uniform semicircular ring of radius R and mass M.
View SolutionFor a uniform ring of mass M and radius R at its centre
View SolutionA thin conducting ring of radius R is given a charge +Q, Fig. The electric field at the center O of the ring due to the charge on the part AKB of the ring is E. The electric field at the center due to the charge on part ACDB of the ring is

View SolutionA conducting ring of radius r and resistance R rolls on a horizintal surface with constant velocity v. The magnetic field B is uniform and is normal to the plane of the loop. Choose the correct option.
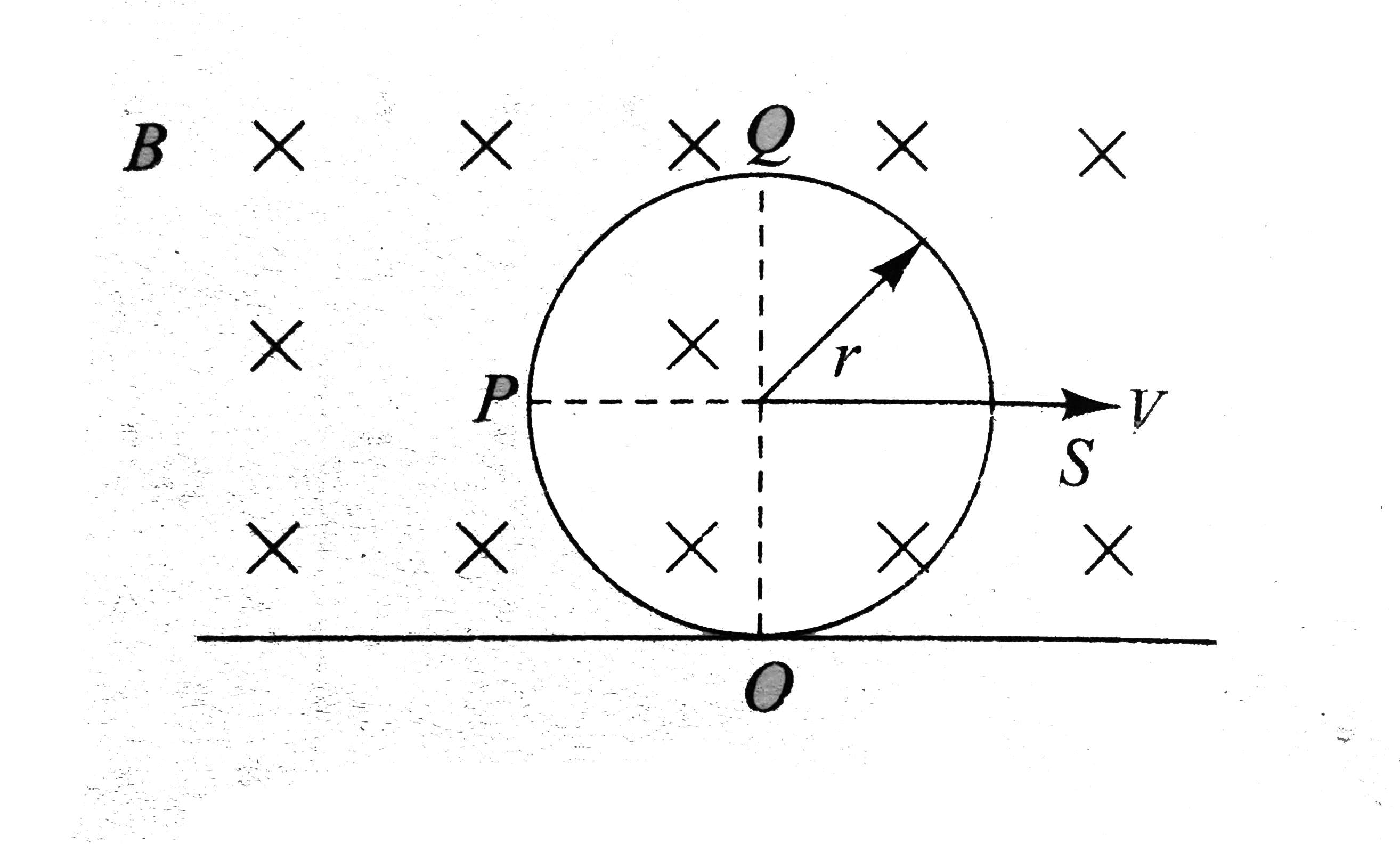
View SolutionA conducting ring of radius r and resistance R is placed in region of uniform time varying magnetic field B which is perpendicular to the plane of the ring. It the magnetic field is changing at a rate α, then the current induced in the ring is
View SolutionFind potential at a point 'P' at a distance 'x' on the axis away from centre of a uniform ring of mass M and radius R .
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
View Solution
