The mean life time of a radionuclide, if the activity decrease by 4% for every 1h, would b e(product is non-radioactive, i.e., stable )
25h
1.042h
2h
30h
The correct Answer is:a
To find the mean lifetime of a radionuclide given that its activity decreases by 4% every hour, we can follow these steps:
Step 1: Understand the decay rate
The activity of a radionuclide decreases by 4% per hour. This means that after one hour, the remaining activity is 96% of the original activity.
Step 2: Express the decay mathematically
If N0 is the initial activity, after one hour, the activity N can be expressed as:
N=N0×(1−0.04)=N0×0.96
Step 3: Relate activity to decay constant
The relationship between the decay constant λ and the activity is given by:
dNdt=−λN
Given that the activity decreases by 4% in one hour (3600 seconds), we can express this as:
dNdt=−0.04N0/3600
Step 4: Set up the equation
From the decay equation, we can equate the two expressions for dNdt:
−λN=−0.04N03600
This simplifies to:
λ=0.043600
Step 5: Calculate the decay constant
Calculating λ:
λ=0.043600=1.11×10−5s−1
Step 6: Find the mean lifetime
The mean lifetime τ of a radionuclide is given by:
τ=1λ
Substituting the value of λ:
τ=11.11×10−5≈90000s
Step 7: Convert seconds to hours
To convert seconds into hours, we divide by the number of seconds in an hour (3600 seconds):
τ (in hours)=900003600≈25hours
Final Answer
The mean lifetime of the radionuclide is approximately 25 hours.
---
The activity of a radionuclide decreases by 4% per hour. This means that after one hour, the remaining activity is 96% of the original activity.
If N0 is the initial activity, after one hour, the activity N can be expressed as:
N=N0×(1−0.04)=N0×0.96
The relationship between the decay constant λ and the activity is given by:
dNdt=−λN
Given that the activity decreases by 4% in one hour (3600 seconds), we can express this as:
dNdt=−0.04N0/3600
From the decay equation, we can equate the two expressions for dNdt:
−λN=−0.04N03600
This simplifies to:
λ=0.043600
Calculating λ:
λ=0.043600=1.11×10−5s−1
The mean lifetime τ of a radionuclide is given by:
τ=1λ
Substituting the value of λ:
τ=11.11×10−5≈90000s
To convert seconds into hours, we divide by the number of seconds in an hour (3600 seconds):
τ (in hours)=900003600≈25hours
The mean lifetime of the radionuclide is approximately 25 hours.
Topper's Solved these Questions
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:Linked Comprehension
Explore 29 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:Integer
Explore 6 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:Subjective
Explore 35 Videos
- MISCELLANEOUS VOLUME 5Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 5Exercise:Integer
Explore 12 Videos
- PHOTOELECTRIC EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:PHOTOELECTRIC EFFECTExercise:Integer Type
Explore 4 Videos
Similar Questions
Find the decay constant of .55Co radio nuclide if its activity is known to decrease 4% per hour. The decay product is non-radioactive.
View SolutionDefine the activity of a radio nuclide. Write its S.I. unit. Give a plot of the activity of a radioactive species versus time.
How long will a radioactive isotope, whose half life is T years, take for its activity to reduce to 1/8th of its initial value ?
View SolutionA radioactive with decay constant λ is being produced in a nuclear ractor at a rate q0 per second, where q0 is a positive constant and t is the time. During each decay, E0 energy is released. The production of radionuclide starts at timet=0.
Average power developed in time t due to the decay of the radionuclide is
View SolutionA radioactive with decay constant λ is being produced in a nuclear ractor at a rate q0 per second, where q0 is a positive constant and t is the time. During each decay, E0 energy is released. The production of radionuclide starts at time t=0.
Instantaneous power developed at time t due to the decay of the radionuclide is .
View SolutionMany unstable nuclie can decay spontaneously to a nucleus of lower mass but differnet combination of nucleons. The process of spontaneous emission of radiation is called radioactivity substance.
Radioactive decay is a statistical process. Radiaactivity is independent of all external conditions. The number of decays per unit time or decay rate is called activity. Activity exponentially decrease with time. Mean lifetime is always greater than half-life time.
n number of α-particels per second are being emitted by B atoms of a radioactive element. The half-life of element will be
View SolutionThe activity of a nucleus is inversely proportional to its half of average life. Thus, shorter the half life of an element, greater is its radioactivity, i.e., greater the number of atomsd disintegrating per second. The relation between half life and average life is t1/2=0.693λ=τ×0.693
or τ=1.44t1/2
The half life of a radioactive element is 10 years. What percentage of it will decay in 100 years?
View SolutionRadioactive decay follows first-order kinetic. The mean life and half-life of nuclear decay process are τ=1/λ and t1/2=0.693/λ. Therefore are a number of radioactive elements in nature, their abundance is directly proportional to half life. The amount remaining after n half lives of radioactive elements can be calculated using the relation:
N=N0(12)n
Amount of radioactive elements (activity) decreases with passage of time as
View SolutionThe activity of a nucleus is inversely proportional to its half of average life. Thus, shorter the half life of an element, greater is its radioactivity, i.e., greater the number of atomsd disintegrating per second. The relation between half life and average life is t1/2=0.693λ=τ×0.693
or τ=1.44t1/2
The half-life periods of four isotopes are given 1 = 6.7 years, II = 8000 years, III = 5760 years, IV=2.35×105 years. Which of these is most stable?
View SolutionA colliison between reactant molecules must occur with a certain minimum energy before it is effective in yielding Product molecules. This minimum energy is called activation energy Ea Large the value of activation energy, smaller the value of rate constant k. Larger is the value of activation energy, greater is the effect of temperature rise on rate constant k.
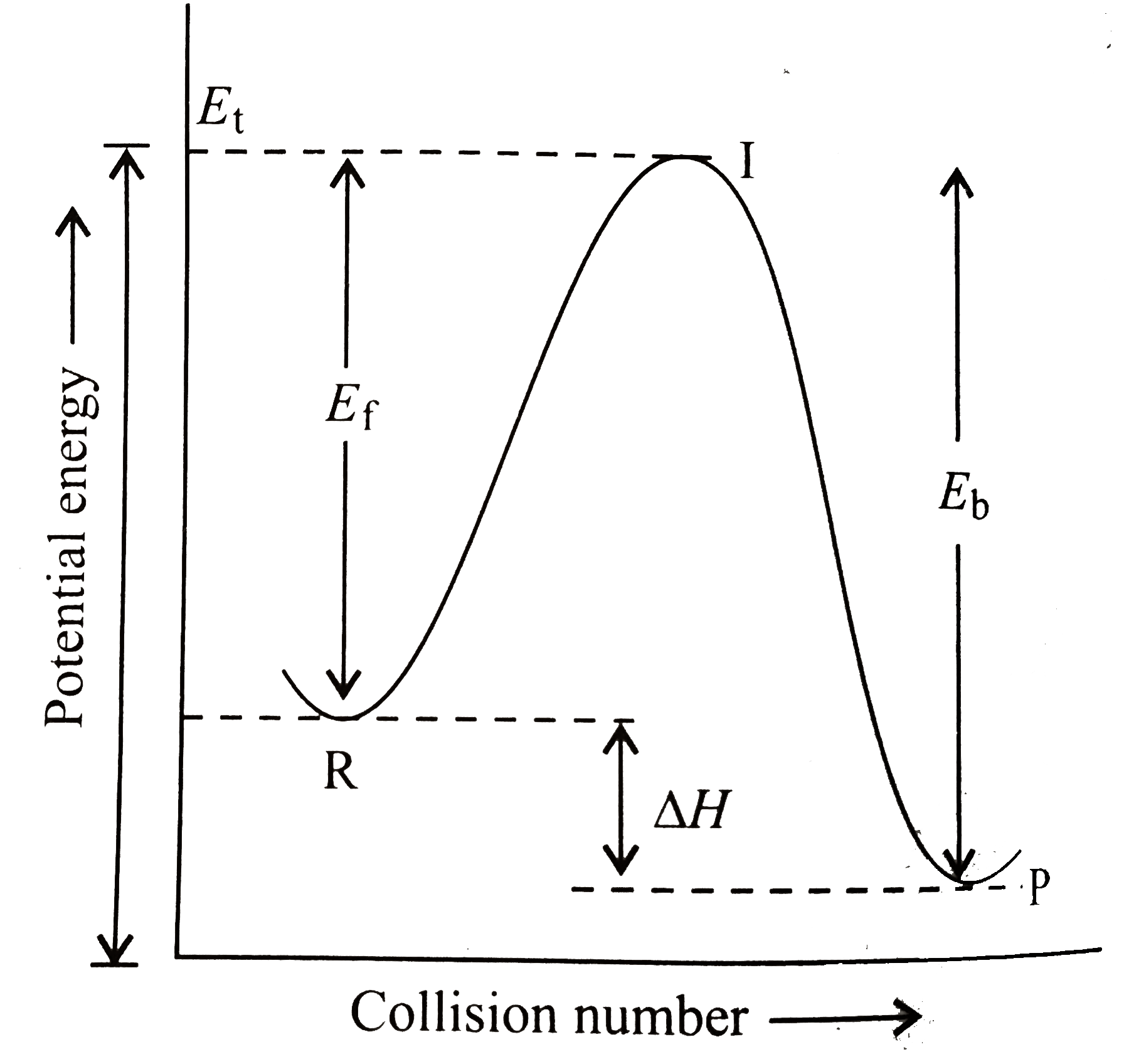
Ef= Activation energy of forward reaction
Eb= Activation energy of backward reaction
ΔH=Ef−Eb
Ef= threshold energy
The activation eneries for forward and backward reactions in a chemical reaction are 30.5 and 45.4kJmol−1 respectively. The reaction is
View SolutionThe activity of a nucleus is inversely proportional to its half of average life. Thus, shorter the half life of an element, greater is its radioactivity, i.e., greater the number of atomsd disintegrating per second. The relation between half life and average life is t1/2=0.693λ=τ×0.693
or τ=1.44t1/2
Mark the incorrect relation.
View Solution