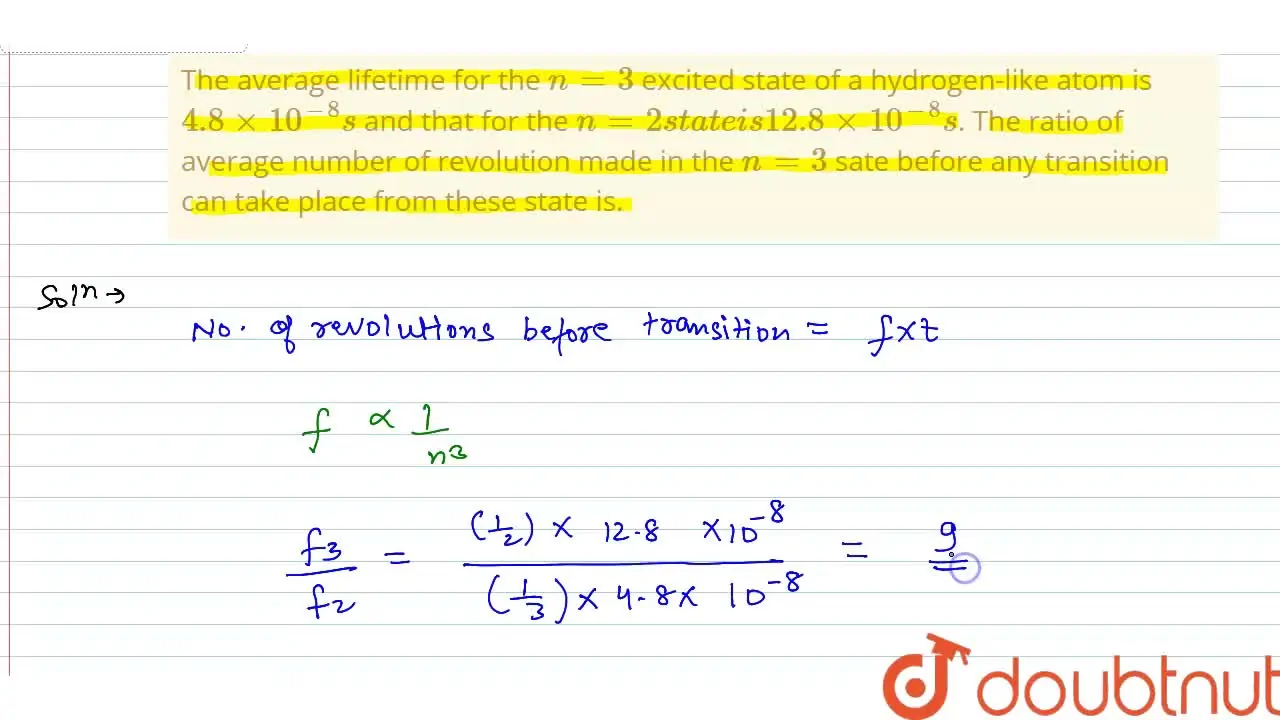
The average lifetime for the n=3 excited state of a hydrogen-like atom is 4.8×10−8s and that for the n=2stateis12.8×10−8s. The ratio of average number of revolution made in the n=3 sate before any transition can take place from these state is.
The correct Answer is:9
Numbersof revolution before transition = frequency xx times
Also, frequency∝1n3
So required ratio=(1/2)12.8×10−8(1/3)4.8×10−8=9
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Fill In The Blanks8videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Single correct answer type22videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Linked Comprehension62videos
ATOMIC PHYSICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct10videos
ALTERNATING CURRENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer5videos
CAPACITOR AND CAPACITANCE
Similar Questions
Avarage lifetime of a hydrogen atom excited to n=2 state 10−6s find the number of revolutions made by the electron on the avarage before it jump to the ground state
View SolutionIf the average life time of an excited state of hydrogen is of the order of 10−8s, estimate how many rotations an electron makes when it is in the state n=2 and before it suffers a transition to staten=1(Bohrradiusa0=5.3×10−11m)?
View SolutionThe speed of light in air is 3×108 m/s and the speed of light in glass is 2×108 m/s. What is the refractive index of glass?
View SolutionIf the average life time of an excited state of hydrogen is of the order of 10−8s, estimate how many whits an alectron makes when it is in the state n=2 and before it suffers a transition to staten=1(Bohrrediusa0=5.3×10−11m)?
View SolutionIf the average life time of an excited state of hydrogen is of the order of 10−8s, estimate how many whits an alectron makes when it is in the state n=2 and before it suffers a transition to staten=1(Bohrrediusa0=5.3×10−11m)?
View SolutionThe time period of revolution of electron in its ground state orbit in a hydrogen atom is 1.60×10−16s. The time period of revolution of the electron in its second excited state in a He+ ion is:
View SolutionThe time period of revolution of electron in its ground state orbit in a hydrogen atom is 1.60×10−16s. The time period of revolution of the electron in its second excited state in a He+ ion is:
View SolutionThe time period of revolution of an electron in its ground state orbit in a hydrogen atom is 1.6×10−16 s. The frequency of the revoltuion in ( s−1). of the electron in its second exited state is
View SolutionThe time period of revolution of an electron in its ground state orbit in a hydrogen atom is 1.6×10−16 s. The frequency of the revoltuion in ( s−1). of the electron in its second exited state is
View SolutionA hydrogen-like atom emits radiation of frequency 2.7×1015 Hz when if makes a transition from n = 2 to n = 1. The frequency emitted in a transition from n = 3 to n = 1 will be
View Solution