Question

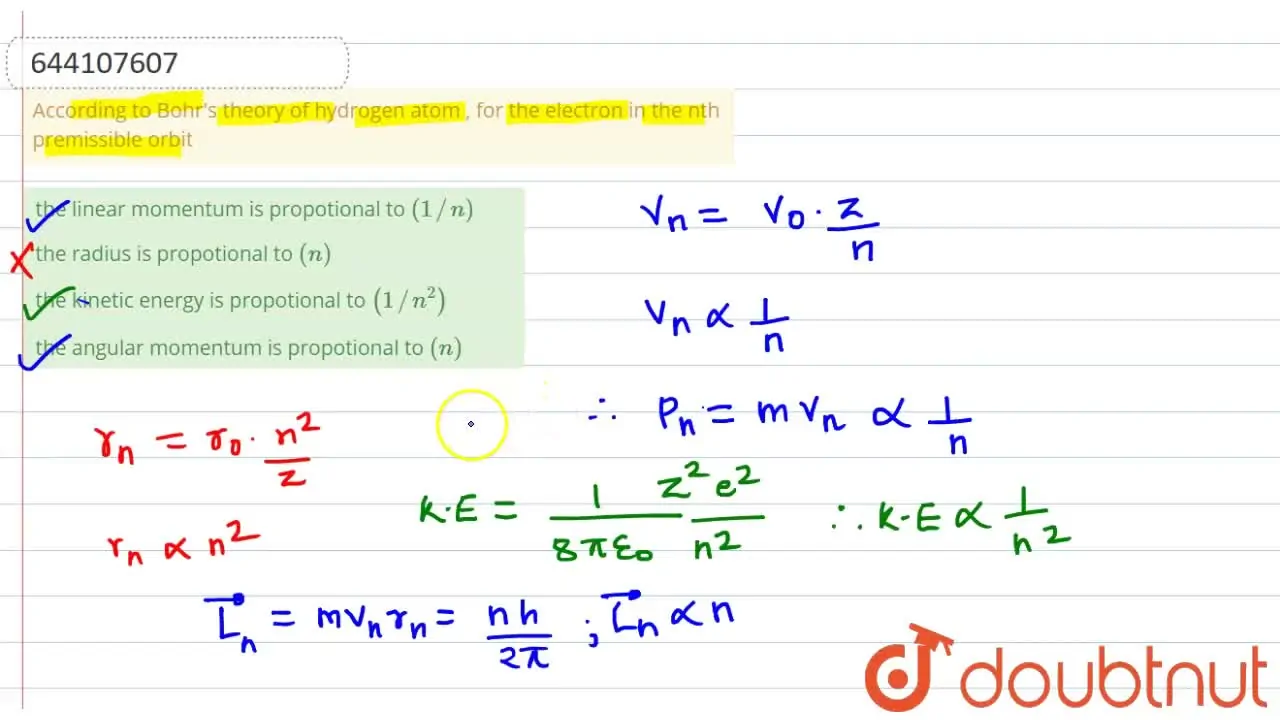
According to Bohr's theory of hydrogen atom , for the electron in the nth premissible orbit

Options

A. the linear momentum is propotional to `(1//n)`
B. the radius is propotional to `(n)`
C. the kinetic energy is propotional to `(1//n^(2))`
D. the angular momentum is propotional to `(n)`

Answer

A::C::D

Explanation

To solve the question regarding the properties of an electron in the nth permissible orbit of a hydrogen atom according to Bohr's theory, we will analyze each of the given options step by step.

Step 1: Analyze Linear Momentum
According to Bohr's theory, the linear momentum \( p \) of the electron in the nth orbit is given by:
\( p \propto \frac{1}{n} \)
This is because the velocity \( v \) of the electron in the nth orbit is inversely proportional to \( n \), and since momentum \( p = mv \) (where \( m \) is the mass of the electron), we conclude that:
\( p \propto \frac{1}{n} \)
Thus, Option A is correct.

Step 2: Analyze Radius of the Orbit
The radius \( R_n \) of the nth orbit is given by:
\( R_n = R_0 \frac{n^2}{Z} \)
where \( R_0 \) is the ground state radius and \( Z \) is the atomic number. From this equation, we see that:
\( R_n \propto n^2 \)
Therefore, Option B is incorrect.

Step 3: Analyze Kinetic Energy
The kinetic energy \( KE \) of the electron in the nth orbit can be expressed as:
\( KE = \frac{1}{8 \pi \epsilon_0} \frac{Z^2 e^2}{n^2} \)
From this, we can see that:
\( KE \propto \frac{1}{n^2} \)
Thus, Option C is correct.

Step 4: Analyze Angular Momentum
The angular momentum \( L \) of the electron in the nth orbit is given by:
\( L = m v_n R_n \)
According to Bohr's quantization condition, angular momentum is also given by:
\( L = \frac{nh}{2\pi} \)
This shows that:
\( L \propto n \)
Thus, Option D is correct.

Conclusion
The correct options based on the analysis are:
- Option A: Correct
- Option B: Incorrect
- Option C: Correct
- Option D: Correct

Final Answer
The correct options are A, C, and D.

---