In the above question, if the scientist continues taking data at higher photon energies he will find the next major ''dip'' in the intensity graph at what photon energy ?
19E0
89E0
3E0
9E0
The correct Answer is:B
To find the next major dip in the intensity graph at higher photon energies, we can follow these steps:
1. Understanding the Initial Dip:
- The first major dip in the intensity graph occurs due to the transition of electrons in a hydrogen atom from the ground state (n=1) to the first excited state (n=2). The corresponding photon energy for this transition is approximately 1.6 eV.
2. Identifying the Next Transition:
- After the transition from n=1 to n=2, the next major dip will occur when the electron transitions from n=1 to n=3. This is because the electrons in the hydrogen atom can only occupy discrete energy levels.
3. Calculating the Energy Levels:
- The energy levels of the hydrogen atom are given by the formula:
En=−E0n2
where E0 is the energy of the ground state (n=1) and n is the principal quantum number.
4. Finding the Energy for n=1 and n=3:
- For n=1 (ground state):
E1=−E0
- For n=3 (first excited state):
E3=−E032=−E09
5. Calculating the Energy Difference:
- The energy difference (ΔE) between the two states (n=1 and n=3) is:
ΔE=E3−E1=(−E09)−(−E0)=−E09+E0
- Simplifying this gives:
ΔE=E0(1−19)=E0(89)
6. Conclusion:
- Therefore, the photon energy corresponding to the next major dip in the intensity graph (from n=1 to n=3) is:
Ephoton=8E09
Final Answer:
The next major dip in the intensity graph will occur at a photon energy of 8E09.
- The first major dip in the intensity graph occurs due to the transition of electrons in a hydrogen atom from the ground state (n=1) to the first excited state (n=2). The corresponding photon energy for this transition is approximately 1.6 eV.
- After the transition from n=1 to n=2, the next major dip will occur when the electron transitions from n=1 to n=3. This is because the electrons in the hydrogen atom can only occupy discrete energy levels.
- The energy levels of the hydrogen atom are given by the formula:
En=−E0n2
where E0 is the energy of the ground state (n=1) and n is the principal quantum number.
- For n=1 (ground state):
E1=−E0
- For n=3 (first excited state):
E3=−E032=−E09
- The energy difference (ΔE) between the two states (n=1 and n=3) is:
ΔE=E3−E1=(−E09)−(−E0)=−E09+E0
- Simplifying this gives:
ΔE=E0(1−19)=E0(89)
- Therefore, the photon energy corresponding to the next major dip in the intensity graph (from n=1 to n=3) is:
Ephoton=8E09
The next major dip in the intensity graph will occur at a photon energy of 8E09.
Topper's Solved these Questions
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Multiple Correct
13 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Linked Comprehension
62 Videos
- ATOMIC PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ATOMIC PHYSICSEXERCISE - Subject
17 Videos
- ALTERNATING CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ALTERNATING CURRENTEXERCISE - Single Correct
10 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
Similar Questions
Knowledge Check
In the question number 48, the energy of photon in eV at the red of the visible spectrum is
A6.63B3.62C7.61D1.64
Find frequency of a photon of energy 3.3 eV?
View SolutionConsider a hydrogen-like ionized atom with atomic number with a single electron. In the emission spectrum of this atom, the photon emitted in the 2 to 1 transition has energy 74.8 higher than the photon emitted in the 3 to 2 transition. The ionization energy of the hydrogen atom is 13.6. The value of Z is __________.
View SolutionThe radiant energy is emitted or absorbed continuously in the form of photons.
View SolutionA hydrogen atom in ground state obsebe a photon of ultraviolet radition of wavelength 50nm Assuming that the entire photon energy is taken up by the electron with what kinetic energy will the up by the electron with what kinetic energy will the electron be ejected?
View SolutionAn atom absorbs a photon of wavelength 500nm and emits another photon of wavelength 700nm. Find the net energy absorbed by the atom in the process.
View SolutionThe Bamber series for the hydrogen atom corresponds to electron that terminal in the state of quantum number n=2.
a Find the longest wavelength photon emitted and determine its energy.
b Find the shortest wavelength photon emitted in the balmer series.
View SolutionIn order to determine the value of E0, a scienctist shines photons (''light particles '')of various energies at a cloud of atomic hydrogen. Most of the hydrogen atom occupy transition through that cloud : see figure is a graph of part of the scientist's data, showing the idensity of the transmitted light as a function of the photon energy. A hydrogen atom's electron is likely to obsorb a photon only if the photon gives the electron enough energy to knock it into a higher shell.
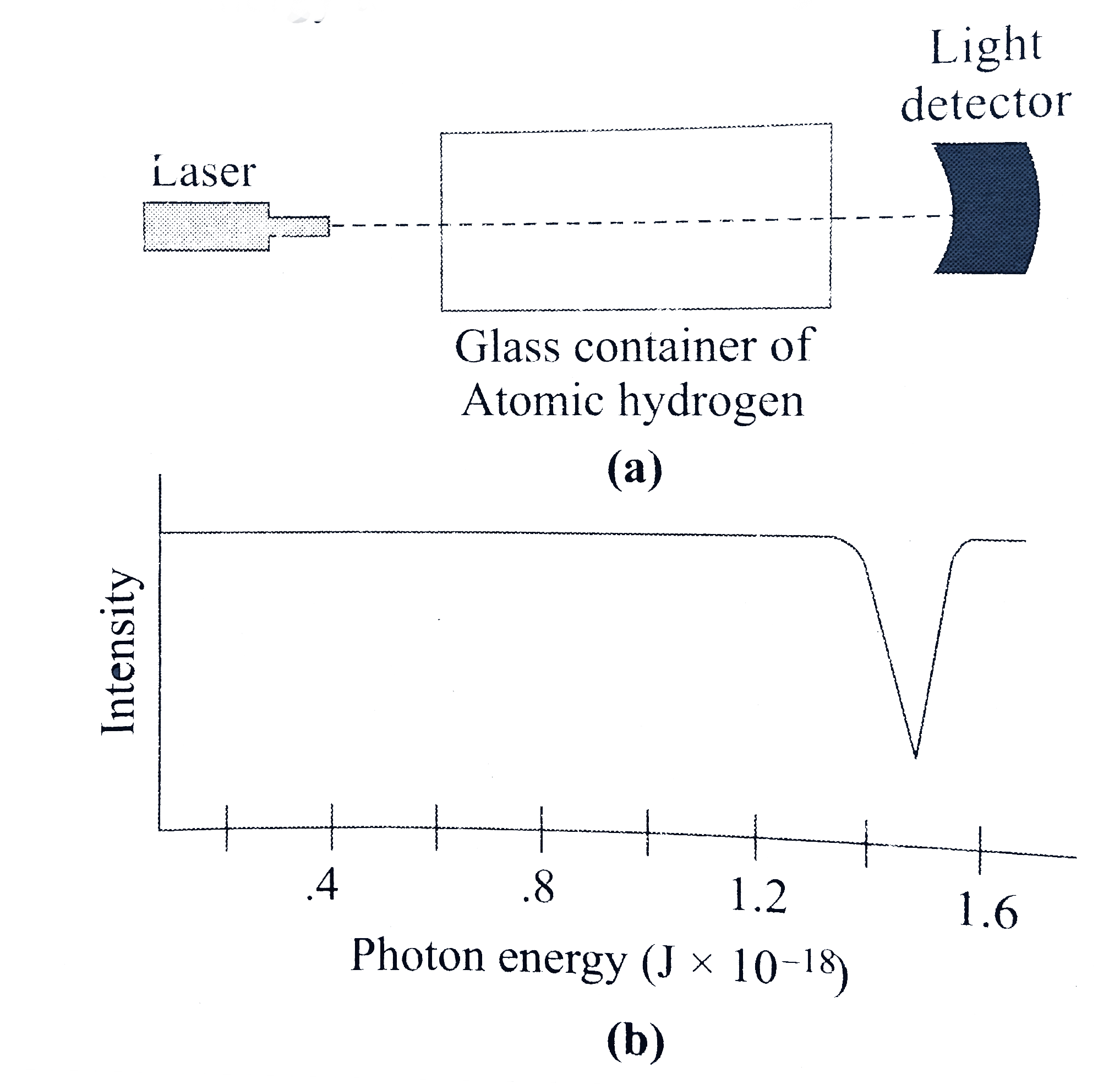
According to this experiment , what is the approximate value of E0?
View Solution
