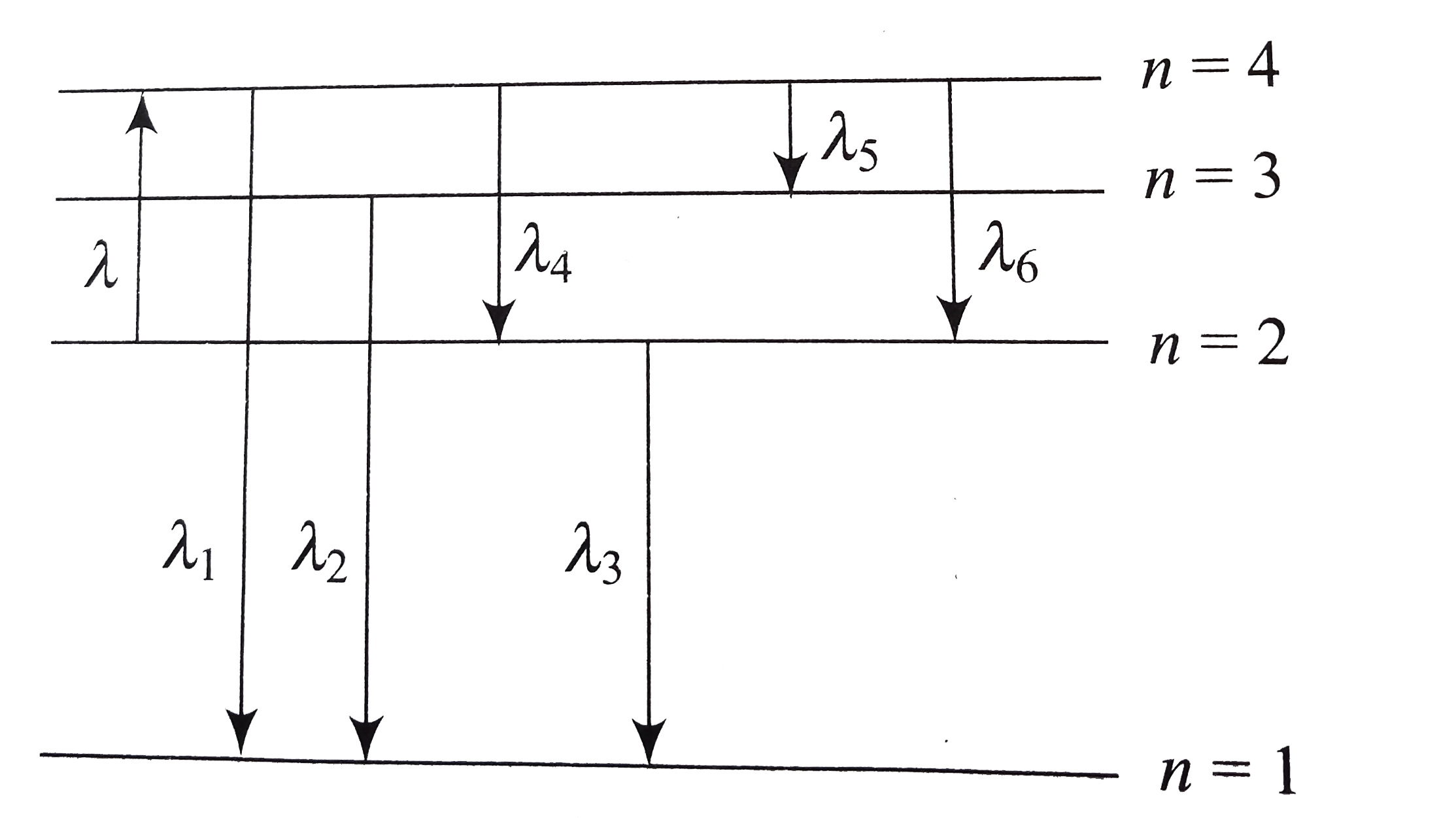
A gas of hydrogen like ions is prepared in such a way that ions are only in the ground state and the first excited state. A monochromatic light of wavelength 1216 Å is absorbed by the ions. The ions are lifted to higher excited states and emit radiations of six wavelengths. Find the principal quantum number of the final excited state.
The energy state of hydrogen-like atom is given by
En=−Bn2
where B=Z2Rhc.
The emission of six wavelength implies that the higher excited state corresponds to quantum number n=4 . As some wavelength are lower and some wavelength are higher , it implies that the initial excited state is n=2 . Thus the principal quantum number of excited states are n=,n=3,n=4.
∴hcλ=B(122−142)⇒hcλ=3B16
∴B=1.6×6.62×10−34×3×1083×(1216×10−10)
=8.71×10−18J
If Z is atimic number of necleus, then B=Z2Rhc
∴Z2=BRhc=8.17×10−18J13.6×1.6×10−19=4
∴Z=2
The maximum wavelength of emitted redition corresponds to transition n=4→n=3 and is given by
hcλmax=B(132−142)
⇒λmax=144hc7B=144×6.62×10−34×3×1087×8.71×10−18
=4.69×10−7m
The minimum wavelength of emitted rediction corresponds to transition n=4→n=1 and is given by
hcλmax=B(112−142)
⇒λmax=16hc15B=16×6.62×10−34×3×10815×8.71×10−18
=0.2×10−7m
Topper's Solved these Questions
- ATOMIC PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:ATOMIC PHYSICSExercise:Exercise 4.1
Explore 15 Videos
- ATOMIC PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:ATOMIC PHYSICSExercise:Exercise 4.2
Explore 12 Videos
- ATOMIC PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:ATOMIC PHYSICSExercise:ddp.4.3
Explore 15 Videos
- ALTERNATING CURRENTBook:CENGAGE PHYSICS ENGLISHChapter:ALTERNATING CURRENTExercise:Single Correct
Explore 10 Videos
- CAPACITOR AND CAPACITANCEBook:CENGAGE PHYSICS ENGLISHChapter:CAPACITOR AND CAPACITANCEExercise:Integer
Explore 5 Videos
Similar Questions
A gas of hydrogen - like ion is perpendicular in such a way that ions are only in the ground state and the first excite state. A monochromatic light of wavelength 1216Å is absorved by the ions. The ions are lifted to higher excited state and emit emit radiation of six wavelength , some higher and some lower than the incident wavelength. Find the principal quantum number of the excited state identify the nuclear charge on the ions . Calculate the values of the maximum and minimum wavelengths.
View SolutionA gas of hydrogen like ions is prepared in such a way that ions are only in the ground state and the first excited state. A monochromatic light of wavelength 1216 Å is absorbed by the ions. The ions are lifted to higher excited states and emit radiations of six wavelengths. Find the principal quantum number of the final excited state.
View Solution
Knowledge Check
Hydrogen atom from excited state comes to the ground state by emitting a photon of wavelength λ. If R is the Rydberg constant, then the principal quatum number n of the excited state is
A√λRλR−1B√λλR−1C√λR2λR−1D√λRλ−1
When a hydrogen atom is excited from ground state to first excited state, then
View SolutionA gaseous excited hydrogen-like species with nuclear charge Z can emit radiations of six different photon energies.
The principal quantum number of the excited state is :
View SolutionAn excited state of H atom emits a photon of wavelength λ and returns in the ground state. The principal quantum number of excited state is given by:
View SolutionA He+ ion is in its first excited state. Its ionization energy is
View SolutionA gas of hydrogen like ions is prepared in a particular excited state A. if emit photons having wavelength equal to the wavelength of the first line of the lyman series together with photons of five other wavelength identify the gas and find the principal quantum number of the state A
View SolutionIf He+ ion is in its first excited state then its ionization energy is
View SolutionHydrogen atom in its ground state is excited by means of monochromatic radiation of wavelength 975A∘. How many different lines are possible in the resulting spectrum? Calculate the longest wavelength amongst them.
View Solution
