A sodium metal piece is illuminated with light of wavelength 0.3 μm. The work function of sodium is 2.46 eV. For this situation ,mark out the correct statement (s).
The maximum kinetic energy of the ejected photoelectrons is 1.68 eV
The cut-off wavelength for sodium is 505 nm
The minimum photon energy of incident light for photoelectric effect to take place is 2.46 eV
All of the above
The correct Answer is:D
To solve the problem, we will follow these steps:
Step 1: Calculate the Energy of Incident Light
We start by calculating the energy of the incident light using the formula:
E=hcλ
Where:
- h (Planck's constant) = 6.626×10−34J s
- c (speed of light) = 3.00×108m/s
- λ (wavelength) = 0.3μm=0.3×10−6m
Substituting the values:
E=(6.626×10−34J s)(3.00×108m/s)0.3×10−6m
Calculating the energy:
E=1.9878×10−250.3×10−6=6.626×10−19J
Now, convert this energy from joules to electron volts (1 eV = 1.6×10−19J):
E=6.626×10−191.6×10−19≈4.14eV
Step 2: Calculate the Maximum Kinetic Energy of Ejected Electrons
Using Einstein's photoelectric equation:
K.E.=E−ϕ
Where ϕ (work function of sodium) = 2.46 eV.
Substituting the values:
K.E.=4.14eV−2.46eV=1.68eV
Step 3: Calculate the Cutoff Wavelength
The cutoff wavelength λ0 can be calculated using the work function:
ϕ=hcλ0
Rearranging gives:
λ0=hcϕ
Substituting the values:
λ0=(6.626×10−34J s)(3.00×108m/s)2.46×1.6×10−19J
Calculating:
λ0=1.9878×10−253.936×10−19≈5.05×10−7m=505nm
Step 4: Determine the Minimum Photon Energy
The minimum photon energy required for the photoelectric effect to occur is equal to the work function:
Emin=ϕ=2.46eV
Conclusion
Based on the calculations:
1. The maximum kinetic energy of ejected electrons is 1.68eV (Correct).
2. The cutoff wavelength of sodium is 505nm (Correct).
3. The minimum photon energy for the photoelectric effect is 2.46eV (Correct).
Thus, all statements are correct.
We start by calculating the energy of the incident light using the formula:
E=hcλ
Where:
- h (Planck's constant) = 6.626×10−34J s
- c (speed of light) = 3.00×108m/s
- λ (wavelength) = 0.3μm=0.3×10−6m
E=(6.626×10−34J s)(3.00×108m/s)0.3×10−6m
E=1.9878×10−250.3×10−6=6.626×10−19J
E=6.626×10−191.6×10−19≈4.14eV
Using Einstein's photoelectric equation:
K.E.=E−ϕ
Where ϕ (work function of sodium) = 2.46 eV.
K.E.=4.14eV−2.46eV=1.68eV
The cutoff wavelength λ0 can be calculated using the work function:
ϕ=hcλ0
Rearranging gives:
λ0=hcϕ
λ0=(6.626×10−34J s)(3.00×108m/s)2.46×1.6×10−19J
λ0=1.9878×10−253.936×10−19≈5.05×10−7m=505nm
The minimum photon energy required for the photoelectric effect to occur is equal to the work function:
Emin=ϕ=2.46eV
Based on the calculations:
1. The maximum kinetic energy of ejected electrons is 1.68eV (Correct).
2. The cutoff wavelength of sodium is 505nm (Correct).
3. The minimum photon energy for the photoelectric effect is 2.46eV (Correct).
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Multiple Correct
10 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Linked Comprehension
44 Videos
- PHOTOELECTRIC EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PHOTOELECTRIC EFFECTEXERCISE - Subjective
16 Videos
- NUCLEAR PHYSICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NUCLEAR PHYSICSEXERCISE - ddp.5.5
14 Videos
- RAY OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RAY OPTICSEXERCISE - DPP 1.6
12 Videos
Similar Questions
For the situation shown in the figure below mark out the correct statement (s) -
 .
.
View SolutionA point source of light is taken away from the experimental setup of photoelectric effect. For this situation, mark out the correct statement (s).
View Solution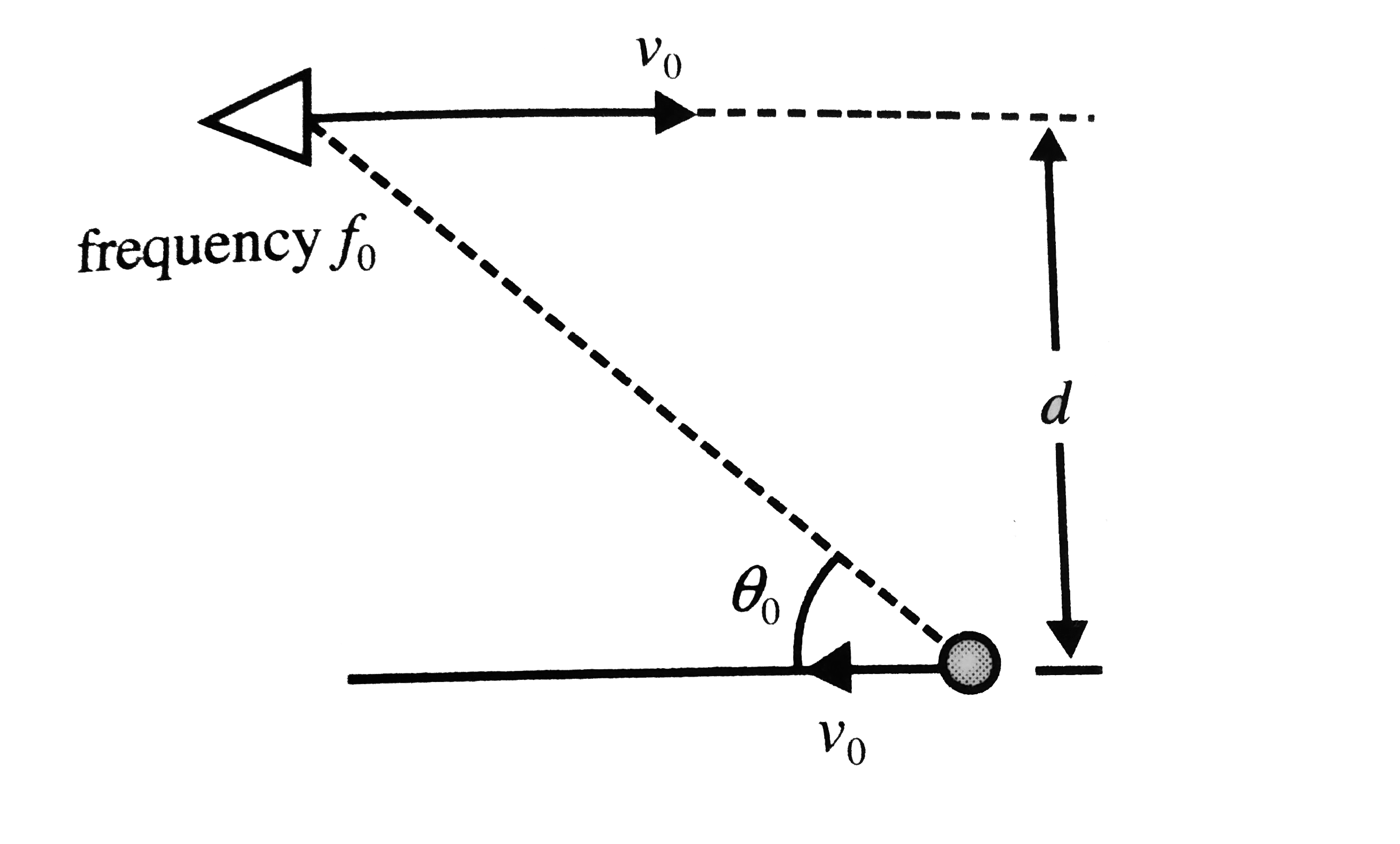
A source of sound and detector are moving as shown in Fig. at t=0. Take velocity of sound wave to be v. For this situation mark out the correct statement(s).
View Solution
A source of sound and detector are moving as shown in Fig. at t=0. Take velocity of sound wave to be v. For this situation mark out the correct statement(s).
View SolutionAn open vessel containing liquid is moving with constant acceleration a on a levelled horizontal surface. For this situation mark out the correct statement(s).
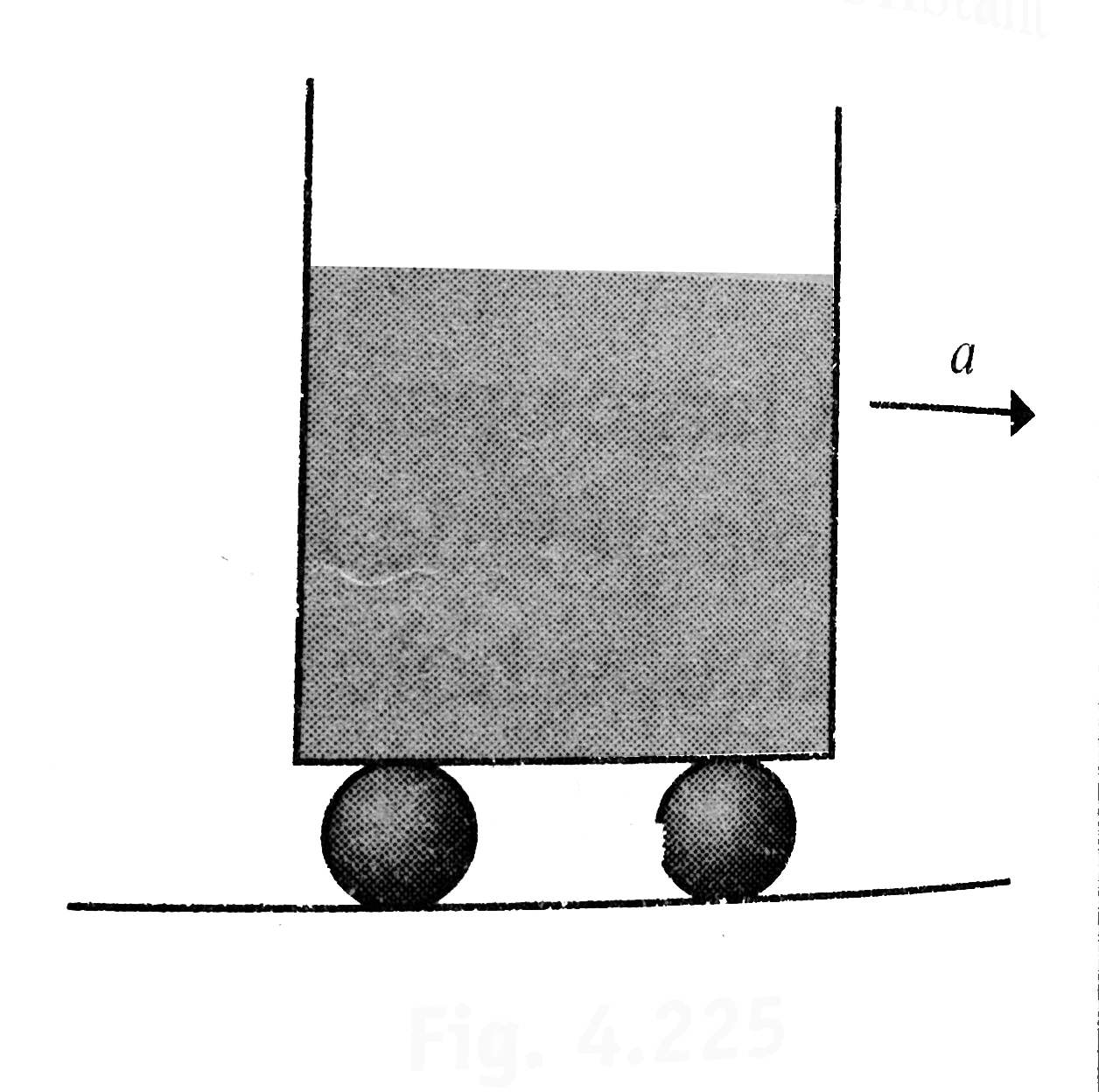
View SolutionThe work function of a metal is 4.2eV , its threshold wavelength will be
View SolutionWhen a metallic surface is illuminated with monochromatic light of wavelength λ, the stopping potential is 5V0. When the same surface is illuminated with light of wavelength 3λ, the stopping potential is V0. Then the work function of the metallic surface is:
View SolutionWhen a metalic surface is illuminated with light of wavelength λ, the stopping potential is V. the same surface is illuminated by light of wavelength 2λ the stopping potential is V3 The thershold waelength for the surface is
View Solution(a) Calculate KE of photoelectron emiited from sodium surface when light of wavelength 400nm is incident on it (Work function of sodium =2.28eV).
(b) Calculate maximum wavelength (threshold wavelength) that can cause emission of photoelectron from sodim surface.
View SolutionWhen a metallic surface is illuminated with monochromatic light of wavelength λ, the stopping potential is 5V0. When the same surface is illuminated with the light of wavelength 3λ, the stopping potential is V0. Then, the work function of the metallic surface is
View Solution
