A biconvex lens has radii of curvature 20 cm and 40cm. The refractive index of the material of the lens is 1.5. An object is placed 40cm in front of the lens. Calculate the psition of the image.
To solve the problem, we will follow these steps:
Step 1: Identify the given values
- Radius of curvature R1=20cm (for the first surface)
- Radius of curvature R2=−40cm (for the second surface, negative because it is on the opposite side)
- Refractive index μ=1.5
- Object distance u=−40cm (negative as per the sign convention)
Step 2: Use the lens maker's formula to find the focal length f
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
Substituting the values:
1f=(1.5−1)(120−1−40)
1f=0.5(120+140)
Step 3: Calculate 120+140
To add these fractions, find a common denominator:
120=240
So,
120+140=240+140=340
Step 4: Substitute back to find 1f
Now substituting back:
1f=0.5(340)=380
Step 5: Calculate the focal length f
Taking the reciprocal:
f=803cm≈26.67cm
Step 6: Use the lens formula to find the image distance v
The lens formula is given by:
1v=1f+1u
Substituting the values:
1v=380+1−40
Step 7: Convert 1−40 to have a common denominator
1−40=−280
So,
1v=380−280=180
Step 8: Calculate v
Taking the reciprocal:
v=80cm
Final Result
The position of the image is 80cm on the opposite side of the lens.
---
- Radius of curvature R1=20cm (for the first surface)
- Radius of curvature R2=−40cm (for the second surface, negative because it is on the opposite side)
- Refractive index μ=1.5
- Object distance u=−40cm (negative as per the sign convention)
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
1f=(1.5−1)(120−1−40)
1f=0.5(120+140)
To add these fractions, find a common denominator:
120=240
So,
120+140=240+140=340
Now substituting back:
1f=0.5(340)=380
Taking the reciprocal:
f=803cm≈26.67cm
The lens formula is given by:
1v=1f+1u
1v=380+1−40
1−40=−280
So,
1v=380−280=180
Taking the reciprocal:
v=80cm
The position of the image is 80cm on the opposite side of the lens.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Exercise1.7
Explore 21 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Subjective
Explore 26 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Exercise1.5
Explore 22 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSExercise:dpp 3.3
Explore 15 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICS ENGLISHChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 27 Videos
Similar Questions
A bicovex lens has radii of cuvature 20 cm each. If the refractive index of the material of the lens is 1.5, what is its focal length?
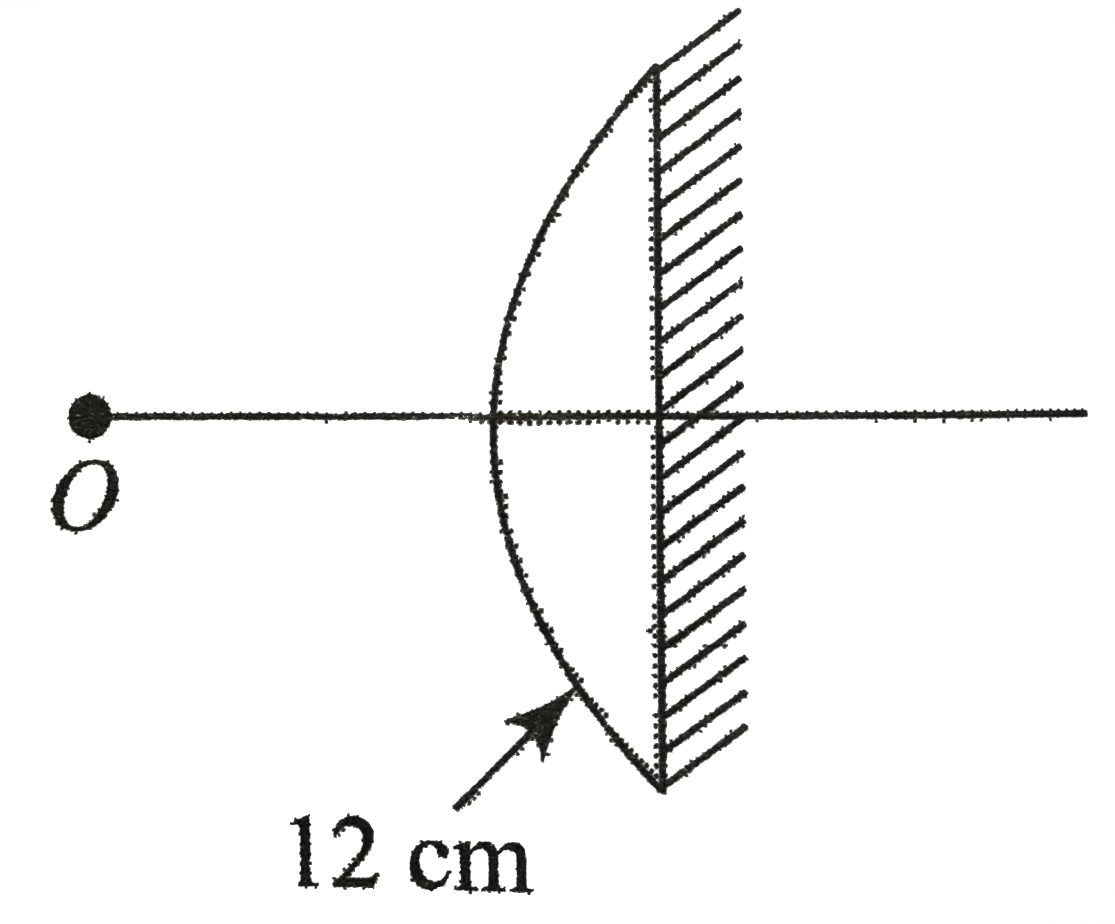
View SolutionA plano-convex lens is silvered on its plane side. The radius of curvature of the other face is 12cm and the refractive index of the material of the lens is 1.5. An object is placed 24 cm in front of the silvered lens. Where will the image be formed?
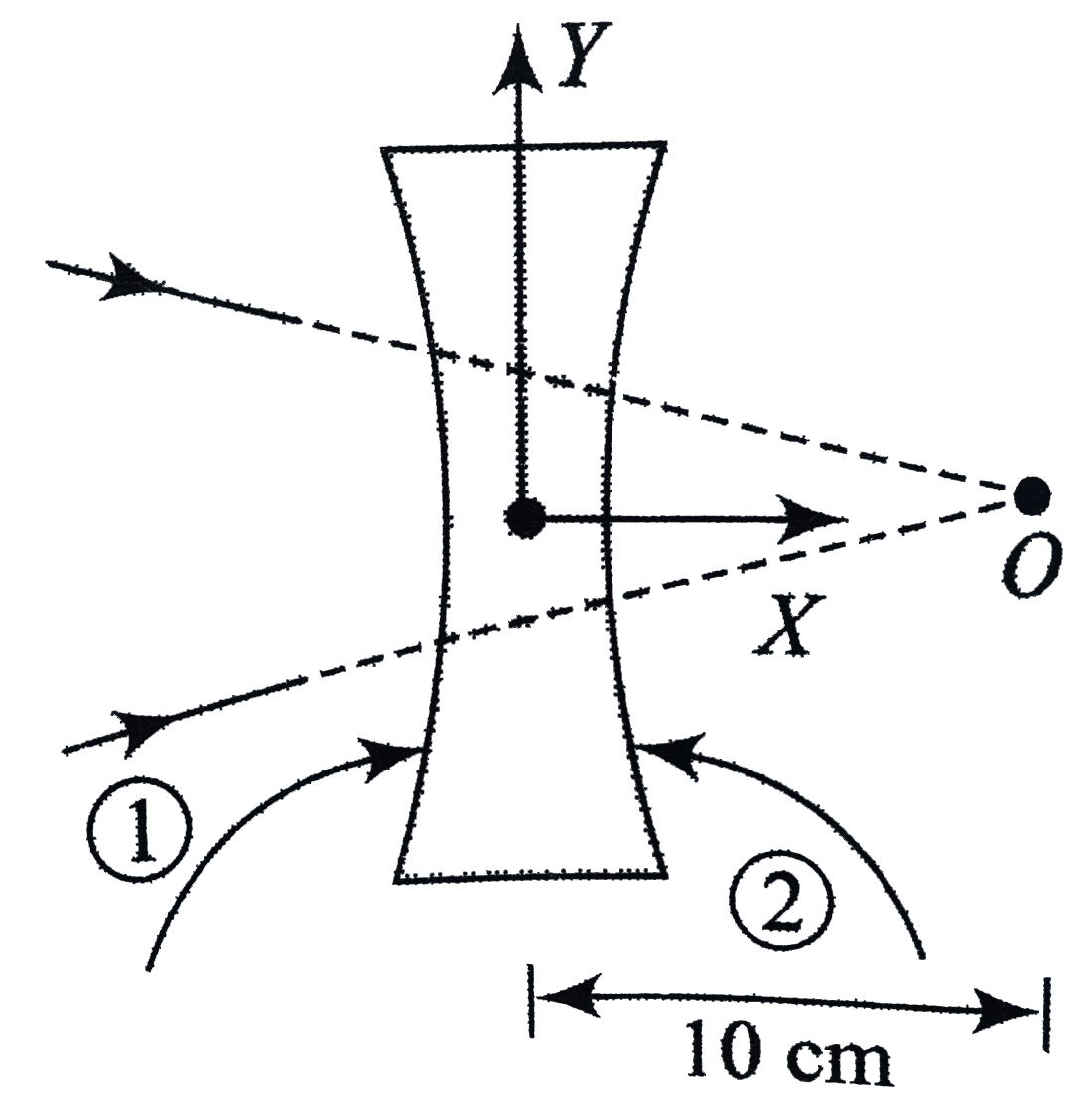
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
View SolutionA convex lens of focal length 20cm is placed co-axially with a convex mirror of radius of curvature 20cm. The two are kept 15cm apart from each other. A point object is placed 60cm in front of the convex lens. Find the position of the image formed by the combination.
View SolutionA diverging meniscus lens of radii of curvatures 25 cm and 50 cm has a refractive index 1.5. Its focal length is (in cm)
View SolutionA glass slab of thickness 2cm and refractive index 2 is placed in contact with a biconvex lens of focal length 20cm. The refractive index of the material of the lens 1.5 . The far side of the lens is silvered. Where should an object be placed in front of the slab so that it images on to itself?
View SolutionThe radii of curvature of the two surfaces of a lens are 20 cm and 30 cm and the refractive index of the material of the lens is 1.5. If the lens is concave -convex, then the focal length of the lens is
View SolutionThe radii of curvature of the two surfaces of a lens are 20 cm and 30 cm and the refractive index of the material of the lens is 1.5. If the lens is concave -convex, then the focal length of the lens is
View SolutionA convex lens of focal length 20cm is placed coaxilly with a convex mirror of radius of curvature 20 cm. The two are kept 15 cm apart. A point object is placed 40 cm in front of the convex lens. Find the position of the image formed by this combination. Draw the ray diagram showing the image formation.
View SolutionA biconvex lens (μ=1.5) has radius of curvature 20cm (both).
find its focal length.

View Solution