Cells A and B and a galvanometer G are connected to a side wire OS by two sliding contacts C and D as shows in Fig. 6.17. The slide wire is 100cm long and has a resistance of 12Ω. With OD=75cm, the galvanometer gives no deflections when OC is 50cm. If D is moved to touch the end of wire S, the value of OC for which the galvanometer shows no deflection is 62.5cm. The emf of cell B is 1.0V. Calculate
(i) the potential difference across O and D when D is at 75cm mark from O
(ii) the potential difference across OS when D touches S
(iii) internal resistance of cell A
(iv) the emf of cell A
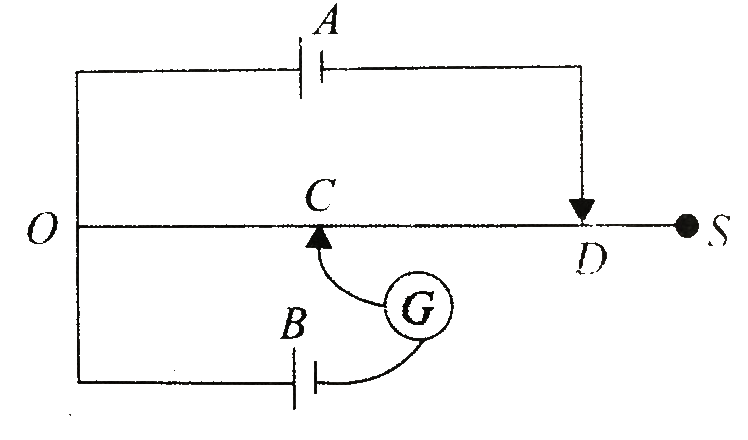
Resistance of wire OD is
12100×75=9Ω
Let E and r be the emf and internal resistance of cell E, respectively.
(i) Since 1V is balanced across 50cm, so potential gradient of wire is 1/50Vcm−1. Therefore, voltage drop across wire OD of length 67cm is (1/50)×75=1.5V.
(ii) Now potential gradient of wire is 1/62.5Vcm−1 Therefore, voltage drop across wire OS of length 100cm is (1/62.5)×100=1.6V.
(iii) For first case
(E9+r)×9=1.5 (i)
For second case
(E12+r)×12=1.6 (ii)
(iv) On solving Eqs. (i) and (ii), we get r=3Ω and E=2V.
Topper's Solved these Questions
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Solved Examples
4 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - Exercise 6.1
15 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - compression type
7 Videos
Similar Questions
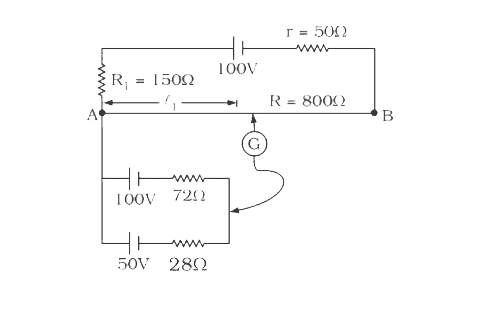
In the circuit shown, the length of AB is 100 cm. The lengthj1 for which the galvanometer shows zero deflection is __________ cm.
View SolutionFor the potentiometer arrangement shown in the figure, length of wire AB is 100 cm and its resistance is 9Ω. Find the length AC for which the galvanometer G will show zero deflection.
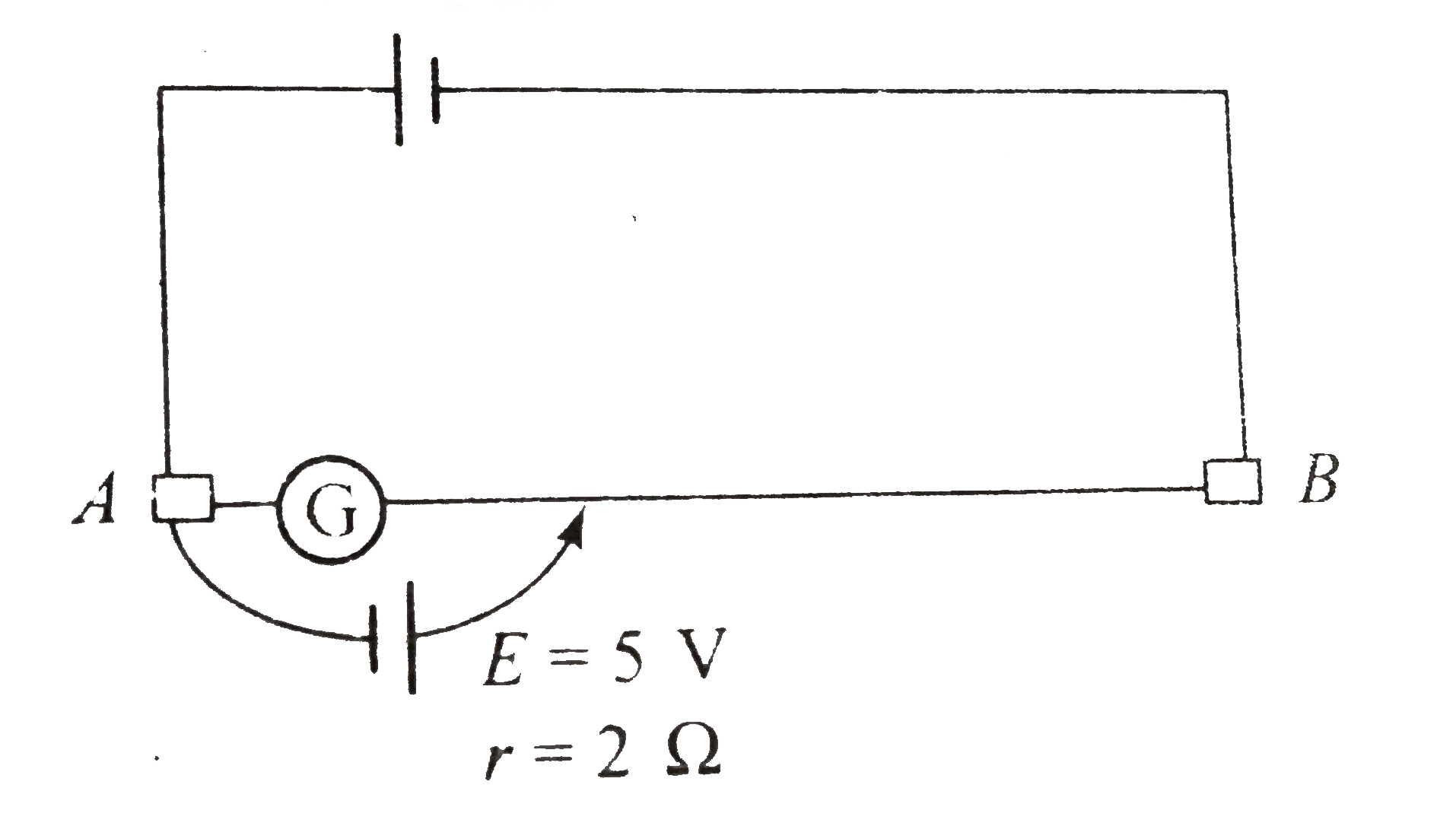
View SolutionIn the potentiometer circuit shown the galvanometer shows no deflection for AD = 35 cm. The resistance of wire AB is 18 Ω and its length is 50 cm . Calculate the emf E of the cell
View SolutionThe potentiometer wire AB is 600cm long. At what distance (in cm) from A should the jockey J touch the wire to get zero deflection in the galvanometer?
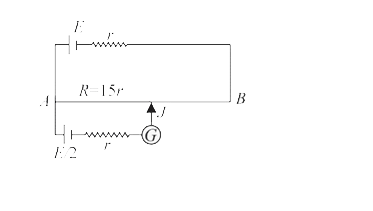
View SolutionThe potentiometer wire AB is 600 cm long . At what distance (in cm) from A should the jockey J touch the wire to get zero deflection in the galvanometer?
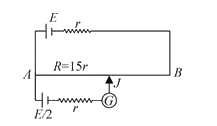
View SolutionThe potentiometer wire AB shown in figure is 40cm long. Where the free end of the galvanometer should be connected on AB so that the galvanometer may show zero deflection?
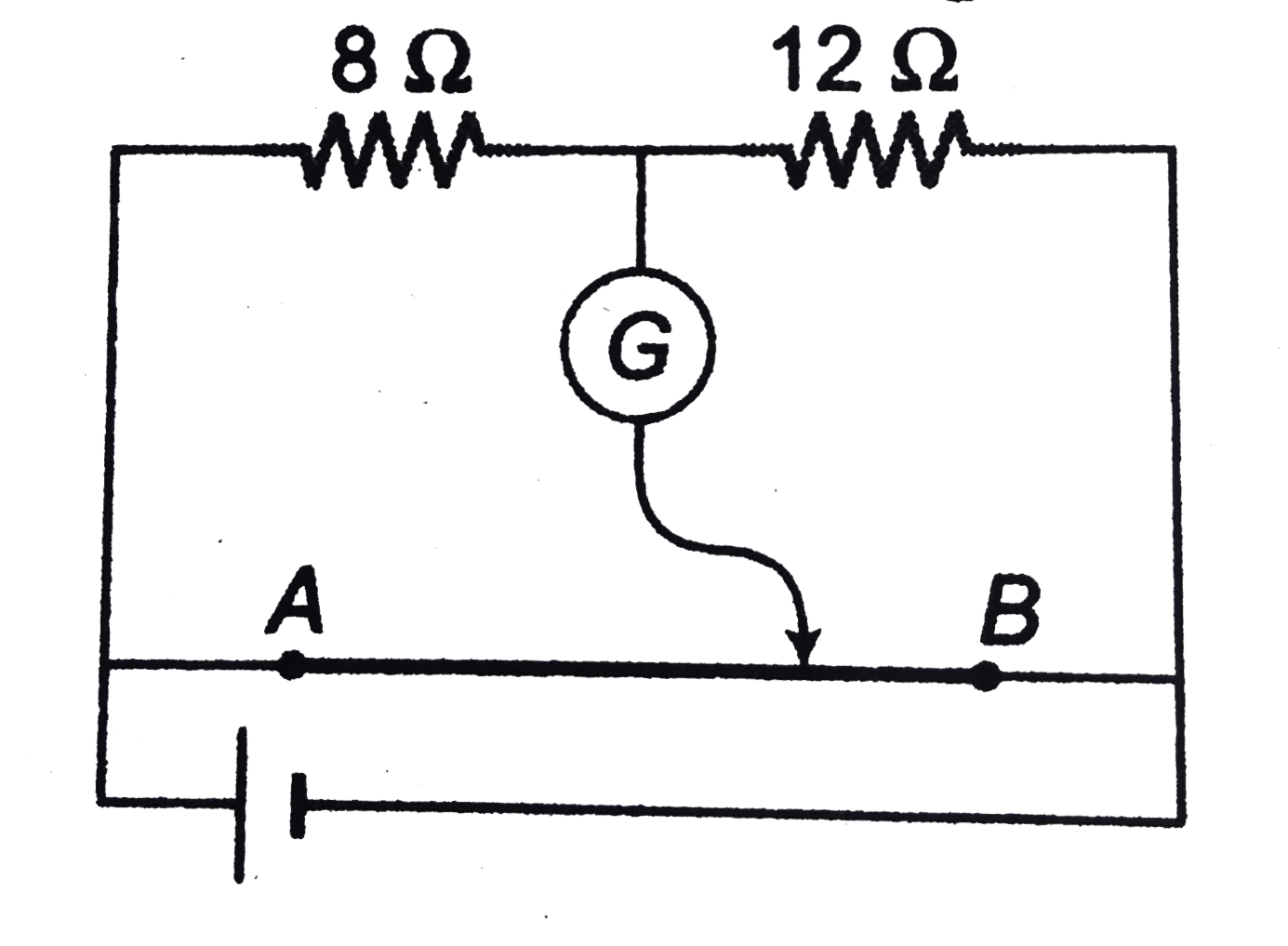
View SolutionIn the adjacent circuit a resisttance R is used. Initially with 'wire AB' not in the circuit, the galavanometer shhows a deflection of d divisions. Now, the wire AB is conneted parallel to the galvanometer and the galvanometer shows a deflection defiction nearly d/2 divisions. Therefore
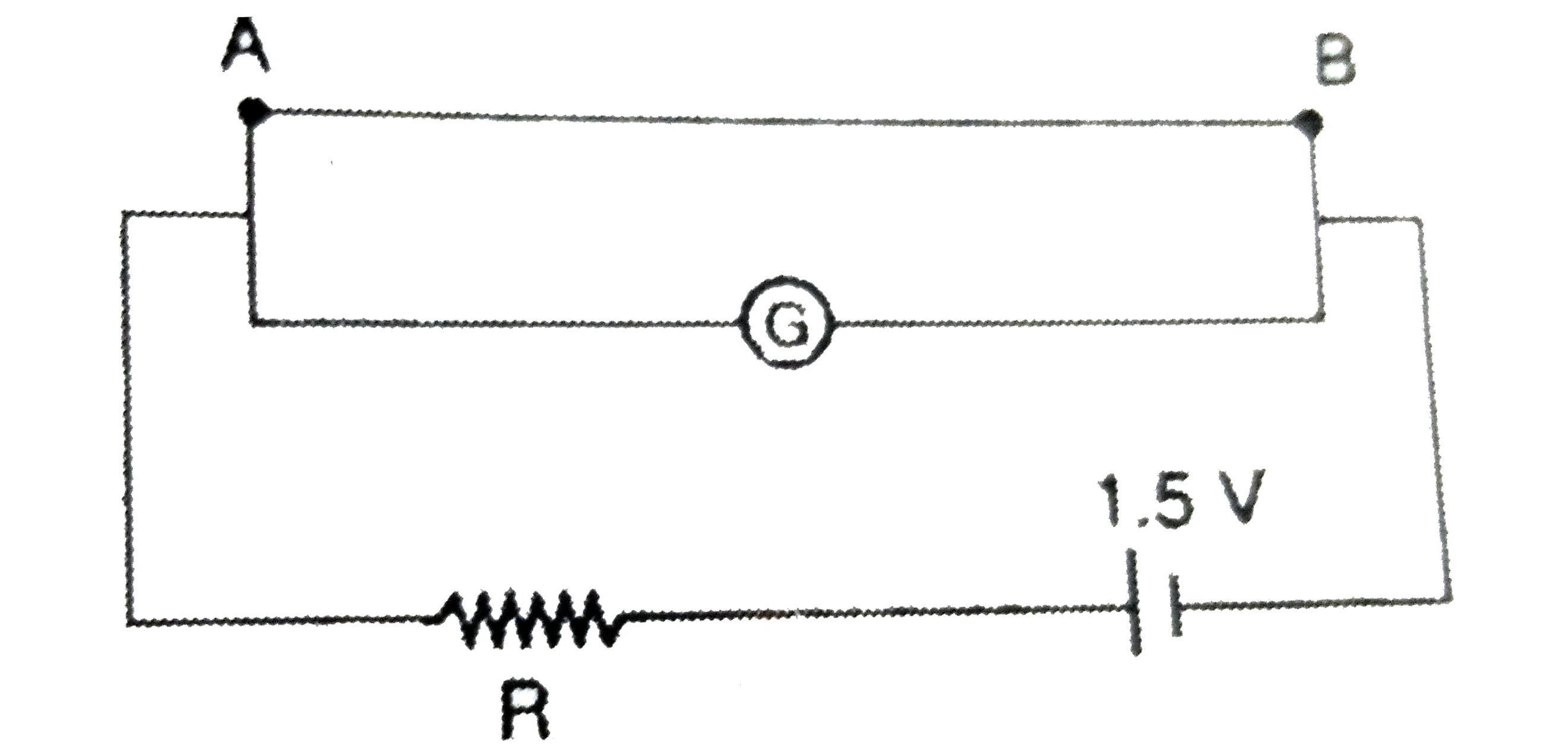
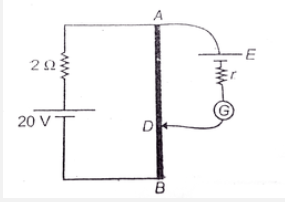
View SolutionAB is a wire of uniform resistance. The galvanometer G shows no deflection when the length AC=20cm and CB=80cm. The resistance R is equal to.
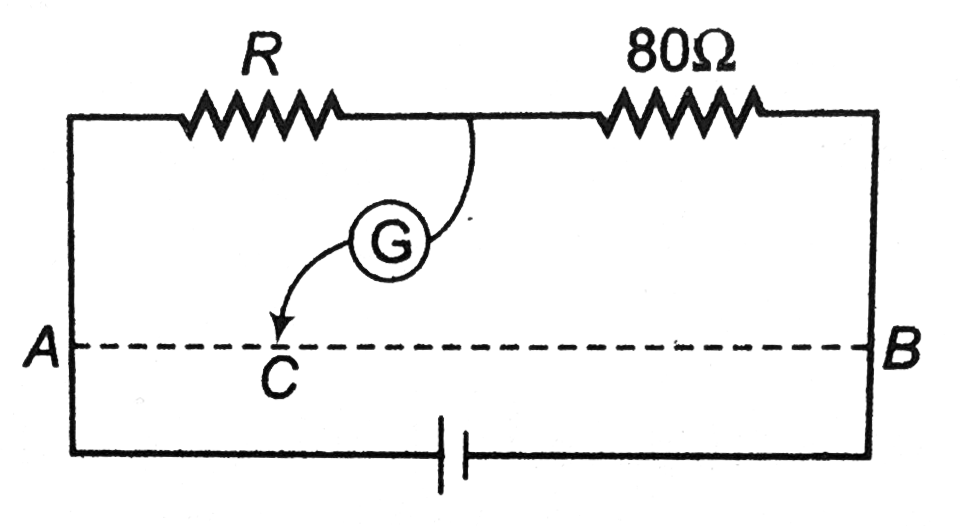 .
.
View SolutionAB is a wire of uniform resistance. The galvanometer G shows no deflection when the length AC=20cm and CB=80cm. The resistance R is equal to.
 .
.
View SolutionIn the circuit, given figure below the length of the wire AB = 400 cm. Where should the jockey J be placed, so that the galvanometer shows a zero deflection?
View Solution
