Find the flux of the electric field through each of the five surfaces of the inclined plane as shown in figure. What is the total flux through the entire closed surface?

Video Solution
Text Solution
Note that flux through the face ABF, CDE, and BCEF is zero. Area vector of face ABF points in the positive z- directions area vector of CDF points in the negative z-direction, and area vector of BCEF points in the negative y- direction. In all the three cases, field E is normal to area vector.
Flux through face ABCD
Magnitude of area vector of face ABCD = ab
ϕE=→E⋅→A=E(ab)cos(90∘−θ)=Eabsinθ
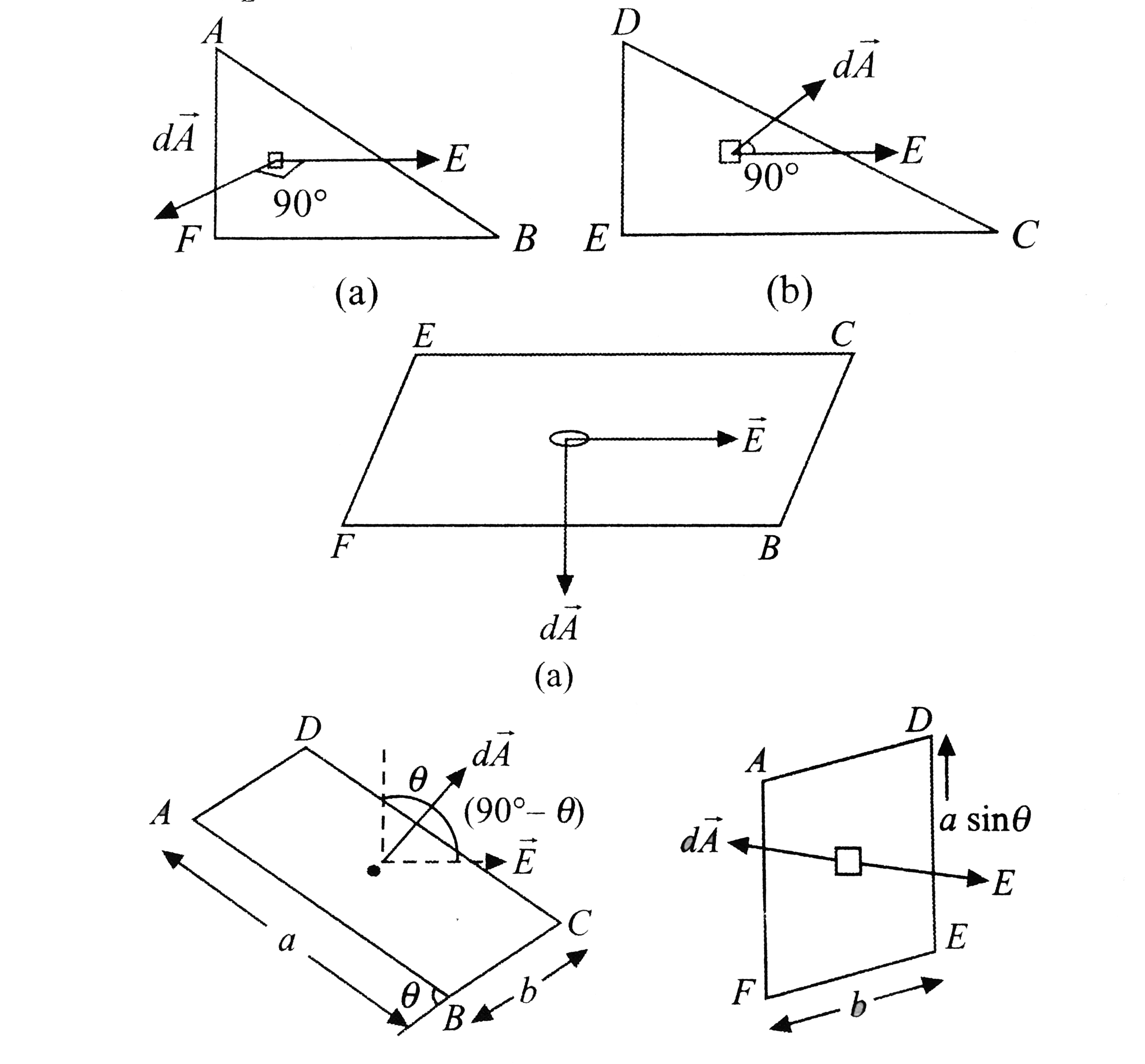
Flux through face ADEF :
Magnitude of area vector of face
ADFE = (asinθ)b=absinθ
ϕE=Ecos180∘'(absinθ)=−Eabsinθ
Thus, we obtain
(ϕE)ABF=0,(ϕE)CDE=0,(ϕE)BCEF=0
(ϕE)ABCD=+Eabsinθand(ϕE)ADEF=−E∣∣∣∫∣∣∣hη.
Flux is a scalar quantity, therefore the total flux is algebraic
sum of flux through each surface.
ϕtotal=(ϕE)ABF+(ϕE)CDE+(ϕE)BCEF
+(ϕE)ABCD+(ϕE)ADEF
=0+0+0+E|∈|θ−Eabsinθ=0 .
|
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Example
7 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - Exercise 2.1
16 Videos
- ELECTRIC CURRENT AND CIRCUITBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger
8 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC POTENTIALEXERCISE - DPP 3.5
15 Videos
Similar Questions
If the flux of the electric field through a closed surface is zero,
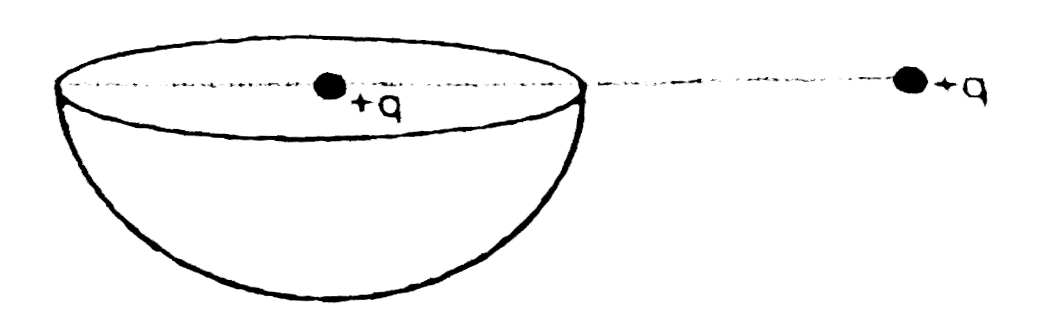
View SolutionFind flux through the hemispherical surface
View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

View SolutionThe electric flux through the surface

View SolutionFind the flux due to the electric field through the curved surface (R is radius of curvature)

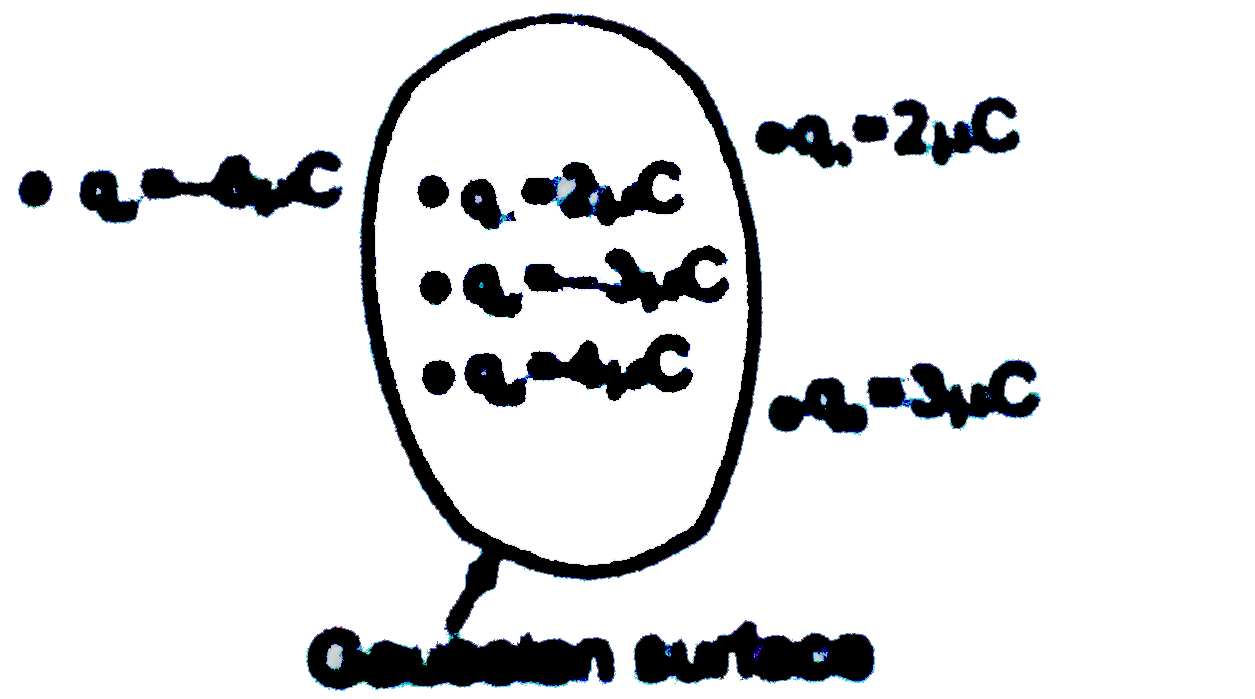
View SolutionFind out flux through the given Gaussian surface.
View SolutionFind the flux of point charge q through the square surface ABCD as shown
View SolutionThe flux of electric field through the circular region of radius r as shown in the figure is
_PHY_E05_291_Q01.png)
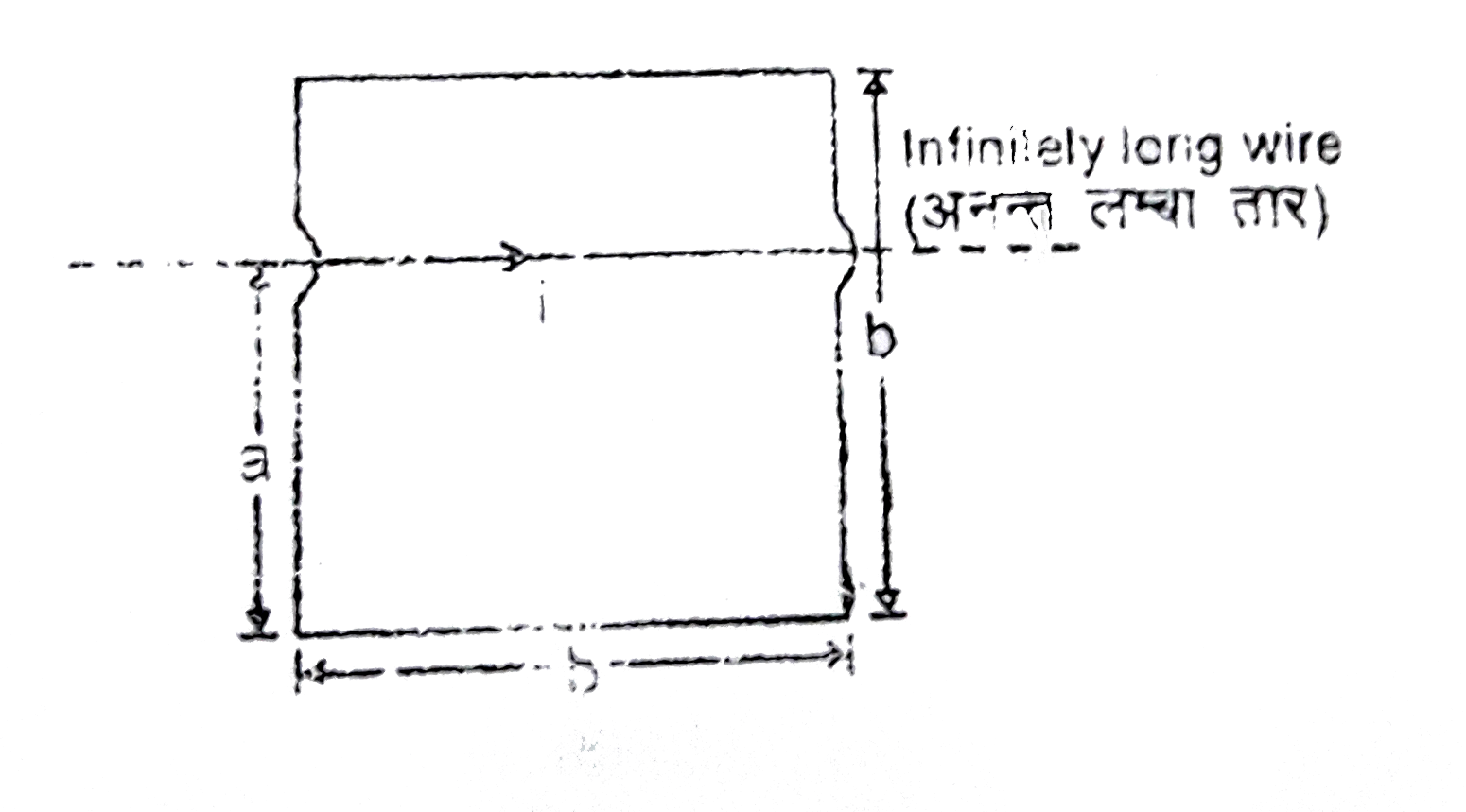
View SolutionFor the situation shown in the figure, flux through the square loop is:
View Solution
