Three charges +q, +q and -2q are placed at the vertices of an equilareral triagle. What is the dipole moment of the system?
To find the dipole moment of the system consisting of three charges +q, +q, and -2q placed at the vertices of an equilateral triangle, we can follow these steps:
Step 1: Understand the Configuration
We have three charges:
- Charge A: -2q (at vertex A)
- Charge B: +q (at vertex B)
- Charge C: +q (at vertex C)
The charges are placed at the vertices of an equilateral triangle.
Step 2: Identify the Dipole Moments
In this configuration, we can consider the pairs of charges that can form dipoles:
1. The dipole formed by the charges at B and A (+q and -2q).
2. The dipole formed by the charges at C and A (+q and -2q).
Step 3: Calculate the Dipole Moment for Each Pair
The dipole moment →p is given by the formula:
→p=q⋅→d
where q is the charge and →d is the displacement vector from the negative charge to the positive charge.
For the dipole formed by +q at B and -2q at A:
- The distance between A and B is the side length of the triangle, which we denote as L.
- The dipole moment →pAB is:
→pAB=q⋅L^rAB
where ^rAB is the unit vector pointing from A to B.
For the dipole formed by +q at C and -2q at A:
- Similarly, the dipole moment →pAC is:
→pAC=q⋅L^rAC
where ^rAC is the unit vector pointing from A to C.
Step 4: Calculate the Resultant Dipole Moment
Since both dipoles are in the same plane and at an angle of 60 degrees to each other (due to the equilateral triangle), we can use the formula for the resultant of two vectors:
|→pnet|=√|→pAB|2+|→pAC|2+2|→pAB||→pAC|cos(60∘)
Substituting the values:
|→pnet|=√(qL)2+(qL)2+2(qL)(qL)⋅12
=√(qL)2+(qL)2+(qL)2=√3(qL)2=√3qL
Step 5: Direction of the Dipole Moment
The direction of the dipole moment is from the negative charge (-2q) towards the positive charges (+q). Given the configuration, the resultant dipole moment will point in the direction opposite to the negative charge.
Final Answer
The dipole moment of the system is:
→pnet=√3qL^r
where ^r is the unit vector pointing from the negative charge to the positive charges.
We have three charges:
- Charge A: -2q (at vertex A)
- Charge B: +q (at vertex B)
- Charge C: +q (at vertex C)
In this configuration, we can consider the pairs of charges that can form dipoles:
1. The dipole formed by the charges at B and A (+q and -2q).
2. The dipole formed by the charges at C and A (+q and -2q).
The dipole moment →p is given by the formula:
→p=q⋅→d
where q is the charge and →d is the displacement vector from the negative charge to the positive charge.
- The distance between A and B is the side length of the triangle, which we denote as L.
- The dipole moment →pAB is:
→pAB=q⋅L^rAB
where ^rAB is the unit vector pointing from A to B.
- Similarly, the dipole moment →pAC is:
→pAC=q⋅L^rAC
where ^rAC is the unit vector pointing from A to C.
Since both dipoles are in the same plane and at an angle of 60 degrees to each other (due to the equilateral triangle), we can use the formula for the resultant of two vectors:
|→pnet|=√|→pAB|2+|→pAC|2+2|→pAB||→pAC|cos(60∘)
|→pnet|=√(qL)2+(qL)2+2(qL)(qL)⋅12
=√(qL)2+(qL)2+(qL)2=√3(qL)2=√3qL
The direction of the dipole moment is from the negative charge (-2q) towards the positive charges (+q). Given the configuration, the resultant dipole moment will point in the direction opposite to the negative charge.
The dipole moment of the system is:
→pnet=√3qL^r
where ^r is the unit vector pointing from the negative charge to the positive charges.
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Solved examples
10 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Exercises
58 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- ELECTRIC CURRENT & CIRCUITSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Kirchhoff s law and simple circuits
15 Videos
Similar Questions
Three charges 2q,−q,and−q are located at the vertices of an equilateral triangle. At the center of the triangle,
View SolutionThree charges Q,(+q) and (+q) are placed at the vertices of an equilateral triangle of side l as shown in the figure. It the net electrostatic energy of the system is zero, then Q is equal to

View Solution
Knowledge Check
Consider the charges q,q and -q placed at the vertices of an equilateral triangle of each side l. What is the force on each charge ?
Aq24√2πε0l2B−q24πε0l2Cq24πε0l2Dzero
Three charges Q,(+q) and (+q) are placed at the vertices of an equilateral triangle of side l as shown in the figure. It the net electrostatic energy of the system is zero, then Q is equal to

View SolutionThree positive charges of equal magnitude q are placed at the vertics of and equilatral triangle of side l. How can the system of charges be palced in equilibrium?
View Solution(a) Three point charges q,-4q and 2q are placed at the vertices of an equilateral triangle ABC of side 'l' as shown in the figure. Obtain the expression for the magnitude of the resultant electric force acting on the charge q.
(b) Find out the amount of the work 4one to separate the charges at infinite distance.

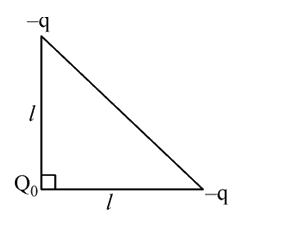
View SolutionThree charges Q0,−q and −q are placed at the vertices of an isosceles right angle triangle as in the figure. The net electrostatic potential energy is zero if Q 0 is equal to :
View SolutionThree equal charges Q are placed at the three vertices of an equilateral triangle. What should be the va,ue of a charge, that when placed at the centroid, reduces the interaction energy of the system to zero ?
View SolutionThree equal charges Q are placed at the three vertices of an equilateral triangle. What should be the va,ue of a charge, that when placed at the centroid, reduces the interaction energy of the system to zero ?
View SolutionThree charges q1=1μC,q2=2μC and q3=3μC are placed on the vertices of an equilateral triangle of side 1.0 m. Find the net electric force acting on charge q1
View Solution
