A box of mass 8kg placed on a rough inclined plane of inclination θ its downward motion can be prevented by applying an upward pull F and it can be made to slide upward appliying a force 2F .The coefficient of friction between the box and the inclined plane is
(tanθ)/3
3tanθ
(tanθ)/2
2tanθ
The correct Answer is:a
To solve the problem, we need to analyze the forces acting on the box on the inclined plane and set up equations based on the information provided.
1. Identify the Forces Acting on the Box:
- The weight of the box (W) is given by W=mg=8kg×9.8m/s2=80N.
- The weight can be resolved into two components:
- Parallel to the incline: W∥=mgsinθ=80sinθ
- Perpendicular to the incline: W_{\perpendicular} = mg \cos \theta = 80 \cos \theta
2. Normal Force (N):
- The normal force acting on the box is equal to the perpendicular component of the weight:
N=mgcosθ=80cosθ
3. Frictional Force:
- The limiting frictional force (F_f) can be expressed as:
Ff=μN=μ(80cosθ)
4. First Condition (Preventing Downward Motion):
- When the upward force F is applied to prevent downward motion, we have:
F=mgsinθ−Ff
- Substituting the expression for friction:
F=80sinθ−μ(80cosθ)
- Rearranging gives:
F=80sinθ−80μcosθ
- Dividing through by 80:
F80=sinθ−μcosθ
- This is our Equation (1).
5. Second Condition (Sliding Upward):
- When the force 2F is applied to make the box slide upward, we have:
2F=mgsinθ+Ff
- Substituting for the frictional force:
2F=80sinθ+μ(80cosθ)
- Rearranging gives:
2F=80sinθ+80μcosθ
- Dividing through by 80:
2F80=sinθ+μcosθ
- This is our Equation (2).
6. Equating the Two Conditions:
- From Equation (1):
F80=sinθ−μcosθ
- From Equation (2):
2F80=sinθ+μcosθ
- Multiply Equation (1) by 2:
2F80=2sinθ−2μcosθ
- Now equate this to Equation (2):
2sinθ−2μcosθ=sinθ+μcosθ
7. Solving for the Coefficient of Friction (μ):
- Rearranging gives:
2sinθ−sinθ=μcosθ+2μcosθ
sinθ=3μcosθ
- Therefore:
μ=sinθ3cosθ=13tanθ
8. Final Result:
- The coefficient of friction μ is:
μ=13tanθ
- The weight of the box (W) is given by W=mg=8kg×9.8m/s2=80N.
- The weight can be resolved into two components:
- Parallel to the incline: W∥=mgsinθ=80sinθ
- Perpendicular to the incline: W_{\perpendicular} = mg \cos \theta = 80 \cos \theta
- The normal force acting on the box is equal to the perpendicular component of the weight:
N=mgcosθ=80cosθ
- The limiting frictional force (F_f) can be expressed as:
Ff=μN=μ(80cosθ)
- When the upward force F is applied to prevent downward motion, we have:
F=mgsinθ−Ff
- Substituting the expression for friction:
F=80sinθ−μ(80cosθ)
- Rearranging gives:
F=80sinθ−80μcosθ
- Dividing through by 80:
F80=sinθ−μcosθ
- This is our Equation (1).
- When the force 2F is applied to make the box slide upward, we have:
2F=mgsinθ+Ff
- Substituting for the frictional force:
2F=80sinθ+μ(80cosθ)
- Rearranging gives:
2F=80sinθ+80μcosθ
- Dividing through by 80:
2F80=sinθ+μcosθ
- This is our Equation (2).
- From Equation (1):
F80=sinθ−μcosθ
- From Equation (2):
2F80=sinθ+μcosθ
- Multiply Equation (1) by 2:
2F80=2sinθ−2μcosθ
- Now equate this to Equation (2):
2sinθ−2μcosθ=sinθ+μcosθ
- Rearranging gives:
2sinθ−sinθ=μcosθ+2μcosθ
sinθ=3μcosθ
- Therefore:
μ=sinθ3cosθ=13tanθ
- The coefficient of friction μ is:
μ=13tanθ
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct
2 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Linked Comprehension
52 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Subjective
21 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A box of mass 8kg placed on a rough inclined plane of inclened θ its downward motion can be prevented by applying an upward pull F and it can be made to slide upward appliying a force 2F .The coefficient of friction between the box and the inclined plane is
View SolutionA box of mass 4 Kg is placed on a rough inclined plane of inclination 60∘. Its downward motion can be prevented by applying an upward pull F. And it can be made to slide upwards by applying a force 3F. The coefficient of friction between the box and inclined plane is
View SolutionA box of mass 8kg placed on a rough inclined plane of inclination θ its downward motion can be prevented by applying an upward pull F and it can be made to slide upward appliying a force 2F .The coefficient of friction between the box and the inclined plane is
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass 5 kg is placed on a rough inclined plane. The inclination of the plane is gradually increased till the block just begins to slide down. The inclination of the plane is than 3 in 5. The coefficient of friction between the block and the plane is (Take, g=10m/s2)
View SolutionA block of mass 5 kg is at rest on a rough inclined surface. If angle of inclination of plane is 60∘, then force applied by it on block is
View SolutionA block of mass 4kg rests on an an inclined plane. The inclination of the plane is gradually increased. It is found that when the inclination is 3 in 5 (sin=θ35) the block just begins to slide down the plane. The coefficient of friction between the block and the plane is.
View SolutionA block of mass 10 kg is placed on rough inclined plane of inclination angle 37∘ .Coefficient of friction between block & plane is 0⋅8, find minimum magnitude of F so that block remains at rest .

View SolutionAn object of mass 10kg is to be kept at rest on an inclined plane making an angle of 37∘to the horizontal by applying a force F along the plane upwards as shown in figure . The coefficient of static fiction between the object and the plane is 0.2 Find the magnitude of force F
[Take g=10ms−2]
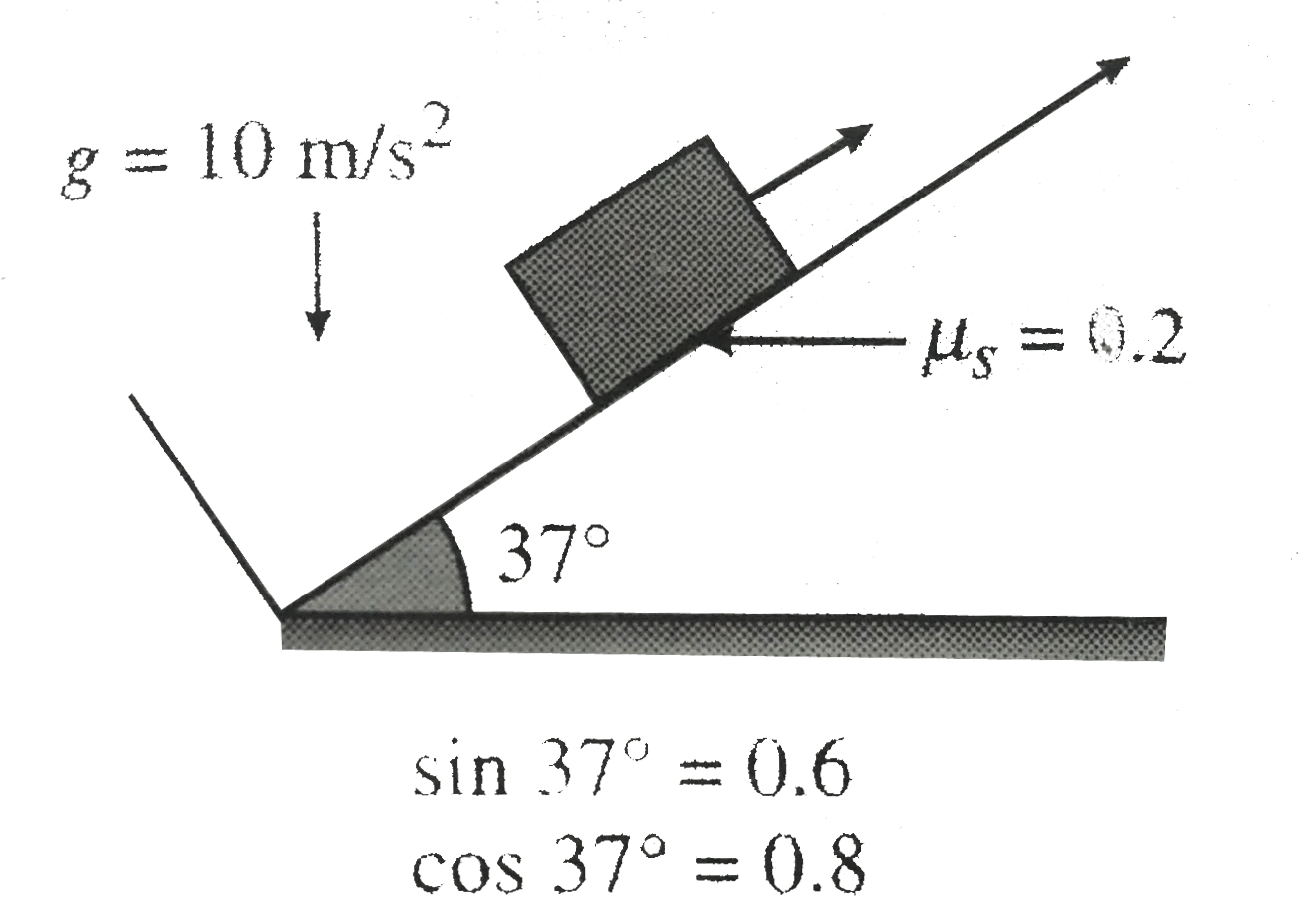
View Solution
