A thin uniform copper rod of length l and mass m rotates uniformly with an angular velocity ω in a horizontal plane about a vertical axis passing through one of its ends. Determine the tension in the rod as a function of the distance r from the rotation axis. Find the elongation of the rod.
To solve the problem of determining the tension in a rotating copper rod and finding its elongation, we can follow these steps:
Step 1: Understanding the System
We have a thin uniform copper rod of length l and mass m rotating with an angular velocity ω about a vertical axis through one of its ends. We need to find the tension T in the rod as a function of the distance r from the rotation axis.
Step 2: Consider a Small Element of the Rod
Let's consider a small element of the rod at a distance r from the rotation axis with a length dr. The tension at this point is T, and at the end of the small element, it is T+dT.
Step 3: Apply Newton's Second Law
The net force acting on the small element towards the center of the circular path is given by:
T+dT−T=dT
This net force must equal the centripetal force required to keep the mass of the small element moving in a circle. The mass dm of the small element can be expressed as:
dm=ml⋅dr
Thus, the centripetal force is:
dm⋅ω2r=ml⋅dr⋅ω2r
Setting the forces equal gives:
dT=ml⋅ω2r⋅dr
Step 4: Integrate to Find Tension
Now we integrate dT from T at r to 0 at l:
∫0TdT=∫lrml⋅ω2rdr
This simplifies to:
−T=mω2l⋅(r22)lr
Evaluating the integral gives:
−T=mω2l⋅(l22−r22)
Thus, we have:
T=mω2l⋅(l2−r22)
Step 5: Find the Elongation of the Rod
To find the elongation ΔL of the rod, we use the relationship between tension and elongation:
TA=E⋅dLL
where A is the cross-sectional area and E is the Young's modulus. Rearranging gives:
dL=T⋅drA⋅E
Substituting for T:
dL=1A⋅E⋅mω2l⋅(l2−r22)dr
Integrating from 0 to l:
ΔL=∫l01A⋅E⋅mω2l⋅(l2−r22)dr
Evaluating this integral leads to:
ΔL=mω2l36AE
Final Results
1. The tension in the rod as a function of distance r from the rotation axis is:
T(r)=mω2l⋅(l2−r22)
2. The elongation of the rod is:
ΔL=mω2l36AE
We have a thin uniform copper rod of length l and mass m rotating with an angular velocity ω about a vertical axis through one of its ends. We need to find the tension T in the rod as a function of the distance r from the rotation axis.
Let's consider a small element of the rod at a distance r from the rotation axis with a length dr. The tension at this point is T, and at the end of the small element, it is T+dT.
The net force acting on the small element towards the center of the circular path is given by:
T+dT−T=dT
This net force must equal the centripetal force required to keep the mass of the small element moving in a circle. The mass dm of the small element can be expressed as:
dm=ml⋅dr
Thus, the centripetal force is:
dm⋅ω2r=ml⋅dr⋅ω2r
Setting the forces equal gives:
dT=ml⋅ω2r⋅dr
Now we integrate dT from T at r to 0 at l:
∫0TdT=∫lrml⋅ω2rdr
This simplifies to:
−T=mω2l⋅(r22)lr
Evaluating the integral gives:
−T=mω2l⋅(l22−r22)
Thus, we have:
T=mω2l⋅(l2−r22)
To find the elongation ΔL of the rod, we use the relationship between tension and elongation:
TA=E⋅dLL
where A is the cross-sectional area and E is the Young's modulus. Rearranging gives:
dL=T⋅drA⋅E
Substituting for T:
dL=1A⋅E⋅mω2l⋅(l2−r22)dr
Integrating from 0 to l:
ΔL=∫l01A⋅E⋅mω2l⋅(l2−r22)dr
Evaluating this integral leads to:
ΔL=mω2l36AE
1. The tension in the rod as a function of distance r from the rotation axis is:
T(r)=mω2l⋅(l2−r22)
2. The elongation of the rod is:
ΔL=mω2l36AE
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.2
16 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Video
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions
A thin uniform copper rod length l and mass m rotates unifomly with an angular velocity ω about a vertical axis passing through one of its ends as shown in the figure. Young's modulus of copper is Y. Breaking stress is σmax, cross sectional area of rod is A and density of rod is uniform. Based on above information, answer the following questions
The maximum angular velocity with which the rod can rotate so that is won't break, is
View SolutionA uniform rod of mass m and length l rotates in a horizontal plane with an angular velocity ω about a vertical axis passing through one end. The tension in the rod at a distance x from the axis is
View SolutionA uniform rod of mass m and length L lies radialy on a disc rotating with angular speed ω in a horizontal plane about vertical axis passing thorugh centre of disc. The rod does not slip on the disc and the centre of the rod is at a distance 2L from the centre of the disc. them the kinetic energy of the rod is

View SolutionA uniform rod of length l, mass m, cross-sectional area A and Young's modulus Y is rotated in horizontal plane about a fixed vertical axis passing through one end, with a constant angular velocity ω. Find the total extension in the rod due to the tension produced in the rod.
View SolutionA uniform rod of length l is being rotated in a horizontal plane with a constant angular speed about an axis passing through one of its ends. If the tension generated in the rod due to rotation is T(x) at a distance x from the axis. Then which of the following graphs depicts it most closely?
View SolutionA uniform rod of mass m and length l0 is rotating with a constant angular speed ω about a vertical axis passing through its point of suspension. Find the moment of inertia of the rod about the axis of rotation if it make an angle θ to the vertical (axis of rotation).
View SolutionA rod is rotating with angular velocity ω about axis AB. Find cosθ.

View SolutionA uniform rod AB of length 2l and mass m is rotating in a horizontal plane about a vertical axis through A, with angular velocity ω, when the mid-point of the rod strikes a fixed nail and is brought immediately to rest. Find the impulse exerted by the nail.
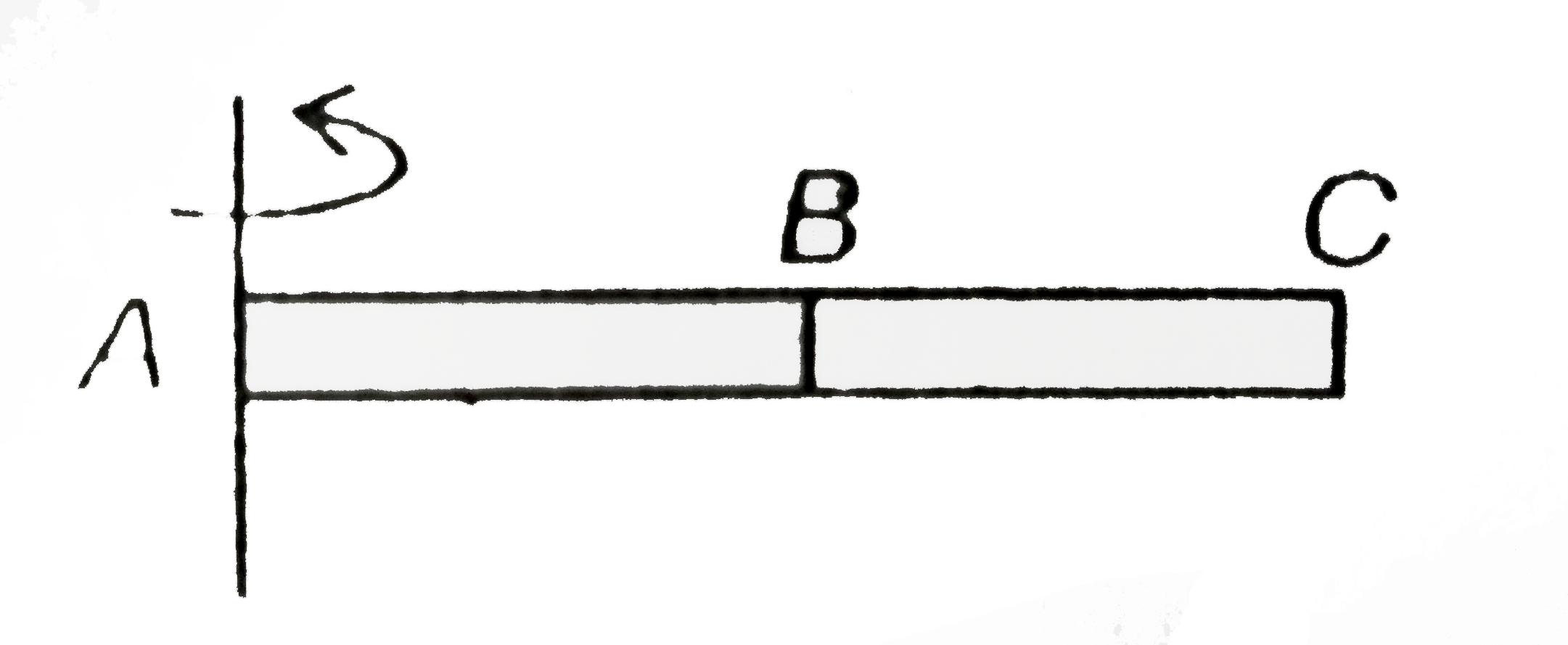
View SolutionA rigid rod of mass m and lengths l, is being rotated in horizontal plane about a vertical axis, passing through one end A. If TA,TBandTC are the tensions in rod at point A, mid point B and point C of rod repsectively, then
View SolutionA rod of length L is pivoted at one end and is rotated with as uniform angular velocity in a horizontal plane. Let T1andT2 be the tensions at the points L/4 and 3L/4 away from the pivoted ends.
View Solution
