Two block M and and m are arranged as shows in figure . If M=50kg then determine the minimum and maximum value of mass of block the heavy block M stationary
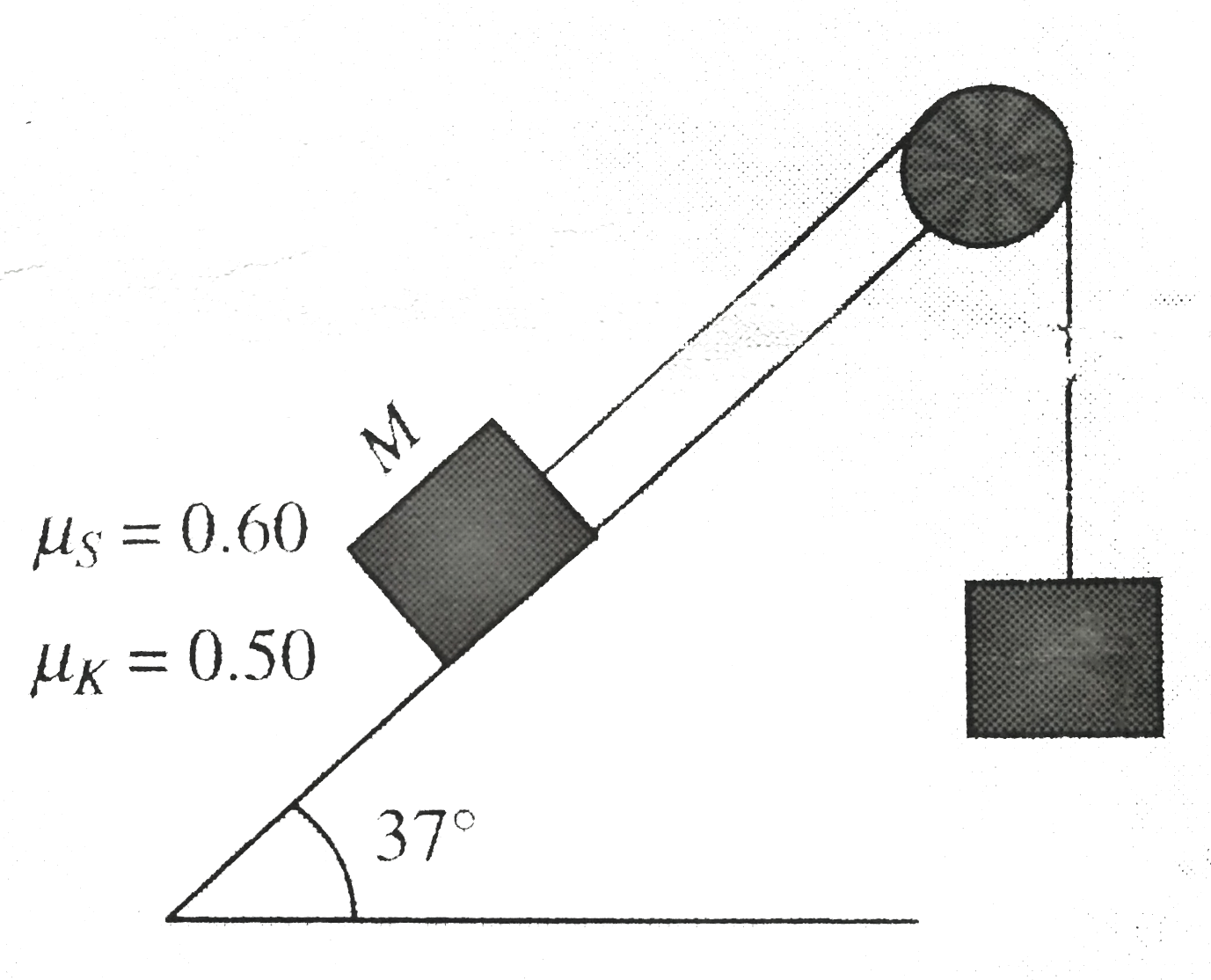
Since the angle of inclination of the plane is more than the angle of repose i, e 37∘>tan−10.5 or tan37∘>0.5 therefore the block M has a tendency to slide down in order to tension in of stationary the neccessury force is applied by the tension in the string
If the block M also has the tendency to side down the friction will act in up the plane
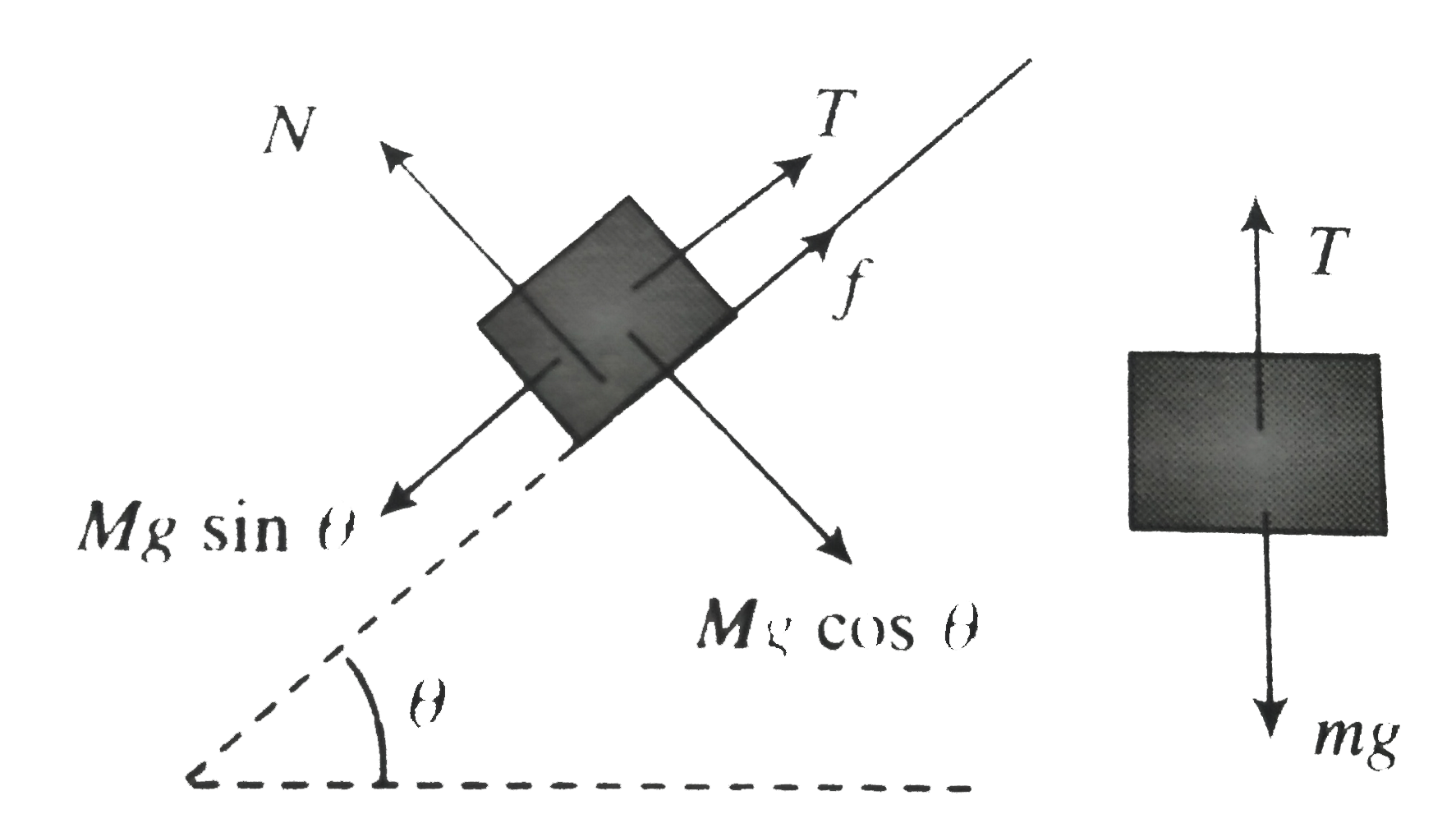
For Equation of M:f=Mgsinθ−T ...(i)
For Equation of m:T=Mg...(ii) from(i) and (ii) f=Mgsinθ−mg ....(iii)
for friction to be staticf<fmax
Mgsinθ−mg+μs(Mgsinθ)
⇒Msinθ−μscosθ<m
Hence , m>M(sinθ−μscosθ) ...(iv)
or m>50(35−35×45)⇒m>6
Hence minimum value of mass mmin=6kg
If block M has the tendency in slide down the friction with act in downward direction by the relation we get the maximum value of m This static of as can be obtation by simply substing (μs) is place of μs in fig (iv) finaly we will get the relation
m>M(sinθ−(−μs)cosθ)
⇒m>M(sinθ+μs)cosθ)
m<50(35+35×45)⇒m<54kg
Hence the maximum value of mass mmax=54kg
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Solved Examples
12 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Exercise 7.1
25 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
Similar Questions

Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :
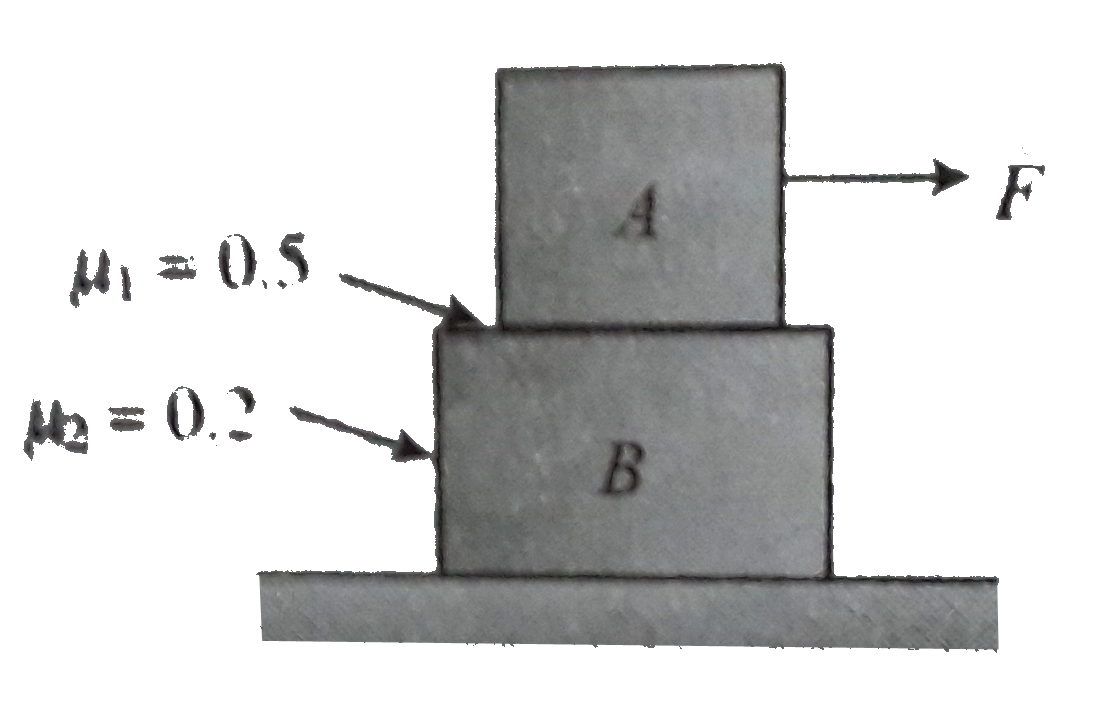
View SolutionTwo block A and B are arrenged as shown in figure (mA=5kgandmB=10kg) Find the acceleration of blocks if F=40N
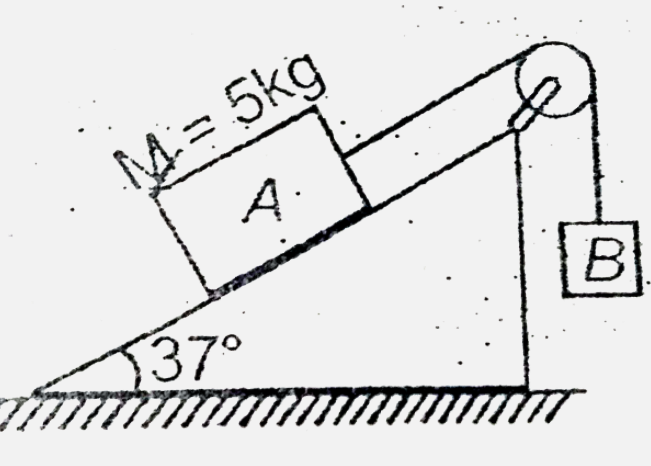
View SolutionTwo blocks A and B are connected by a string as shown as shown in the figure . Friction coefficient of the inclined plane is 0.5 . The mass of the block A is 5 kg. If minimum and maximum values of mass of the block B for which the block A remains in equilibrium are m1andm2 then find the value of (m2−m1) [in kg]
View SolutionTwo block of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is:

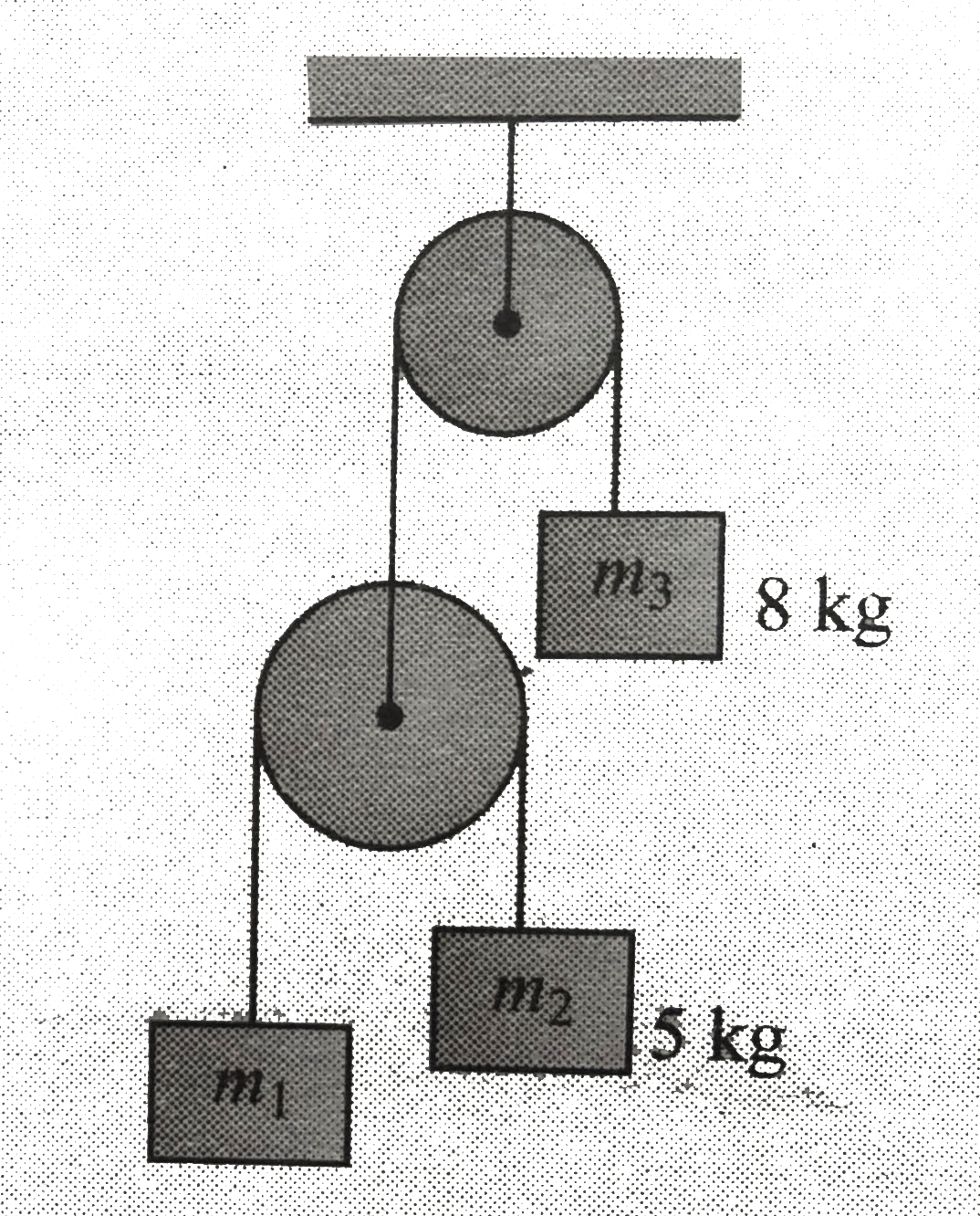
View SolutionThree blocks m1,m2, and m3 are arranged as shown in fig. if m2=5kg and m3=8kg, at what value of m1 will 8kg mass be at rest?
View SolutionTwo blocks of masses'm' and 'M' are arranged as shown in the figure. The coefficient of friction between the two blocks is ' μ ', where as between the lower block and the horizontal surface is zero. Find the force 'F' to be applied on the upper block, for the system to be under equilibrium ?

View SolutionTwo blocks M1. and M2, are tied with string and kept at rough surface as shown in figure. If F = (2t)N is applied on the block of mass M1, then the friction force on the blocks at t = 2 s. is

View SolutionThe minimum value of mass m for which the 100 kg block can remain in static equilibrium is

View SolutionThree blocks A,B and C are suspended as shown in the figure. Mass of each block A and C is m. if the system is in equilibrium and mass of B is M, then

View SolutionA block of mass m collides with another block of mass 3m completely inelastically as shown in figure. What is the maximum value of v( in m/s) for which the block B does not move. Assume that initially spring is in natural length and blocks A and B are at rest. (K/m=100S.I.unit )
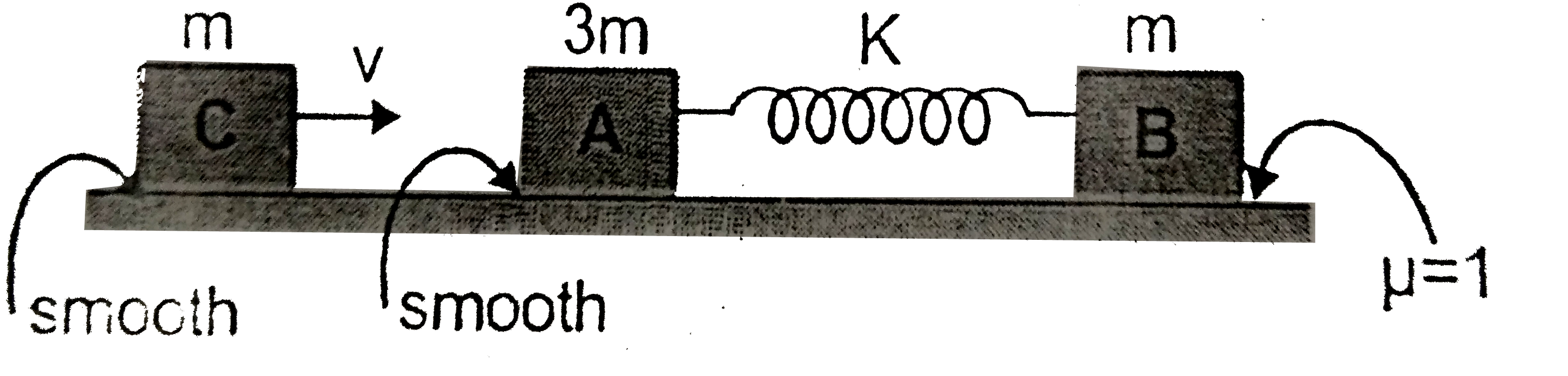
View Solution
