A rod of mass m is supported on a wedge of mass M shown in fig. Find the accelerations of rod and wedge in the arrangement. The friction between all contact surfaces in negligible.
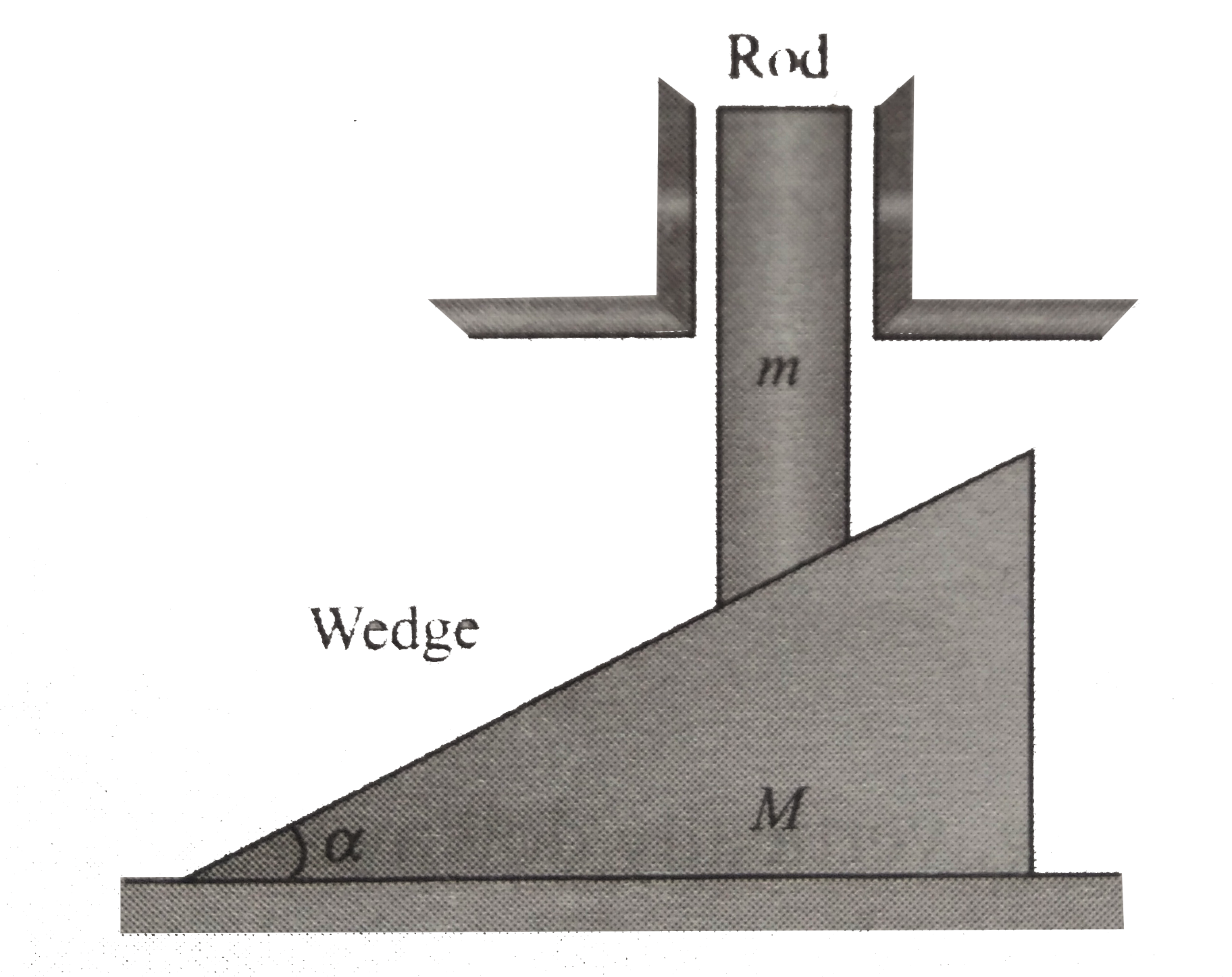
The rod is constrained to move in the vertical direction (with the help of the guids) and the wedge will move along the surface in the horizontal direction. Initially, the system is held at rest.
Constraint relation: Approach 1 Let the acceleration of m w.r.t gound be a vertically downwards and acceleration of M w.r.t ground be A horizontally towards right.
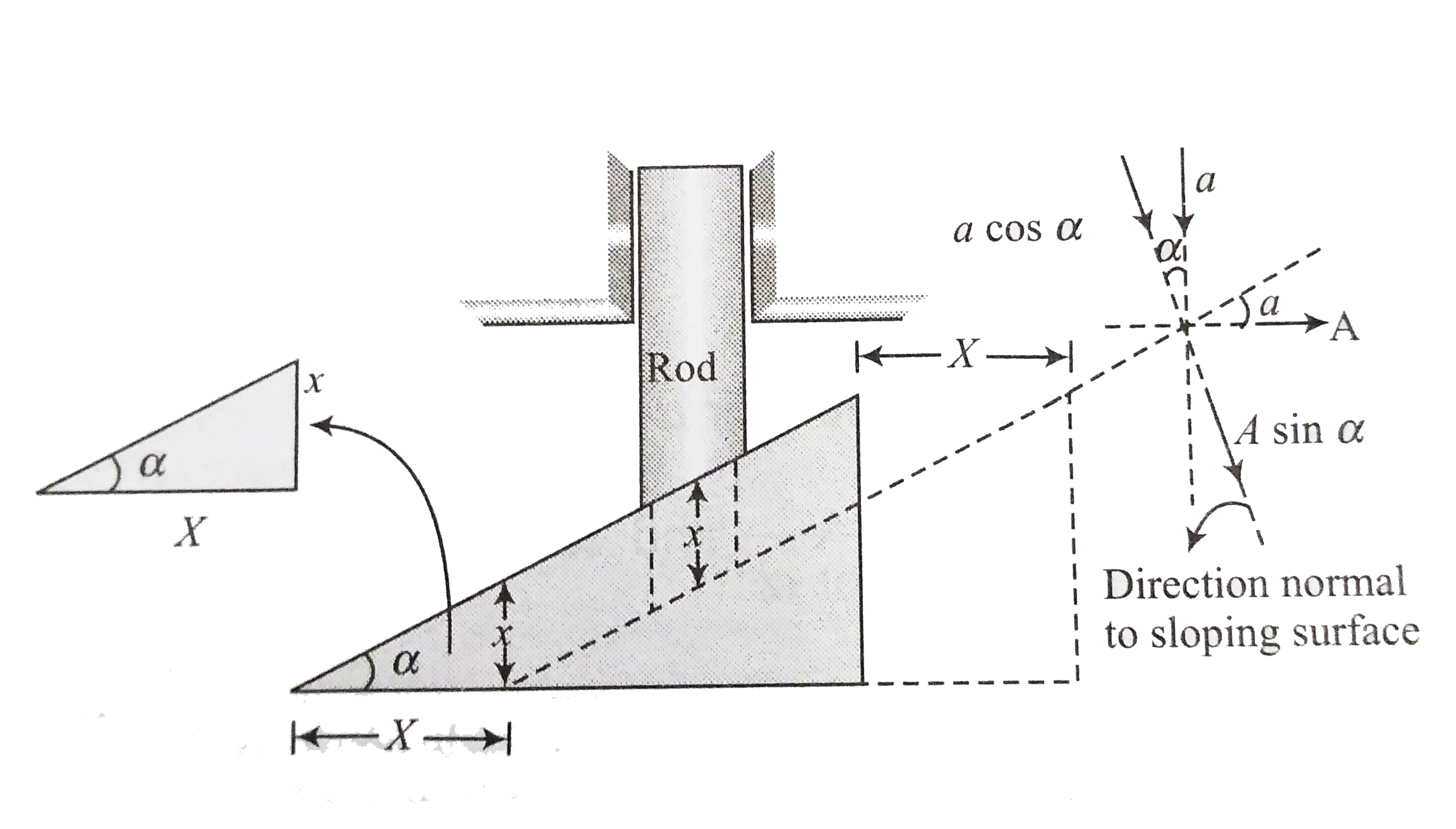
The motion of the system is constrained by the fact that the "bottom face of the rod must always be in contact with the inclined plane. If in time t, X is the displacement of the wedge and x is the displacement of the rod, then the constraint demands that
From fig. we have xX=tanα
∴x=Xtanα ...(i) Here α remains constant.
Differentating (i) w.r.t twice, we get
(d2xdt2)=(d2Xdt2)tanα [tanα=constant]
Hence, a=Atanα
Approch 2: The fact that the rod (or a particle on the wedge and the wedge must not lose contact is usually called wedge constraints. For this, the component of the acceleration of the rod perpendicular to the wedge plane = component of acceleration of the wedge perpendicular to wedge plane.
acosα=Asinα⇒a=Atanα
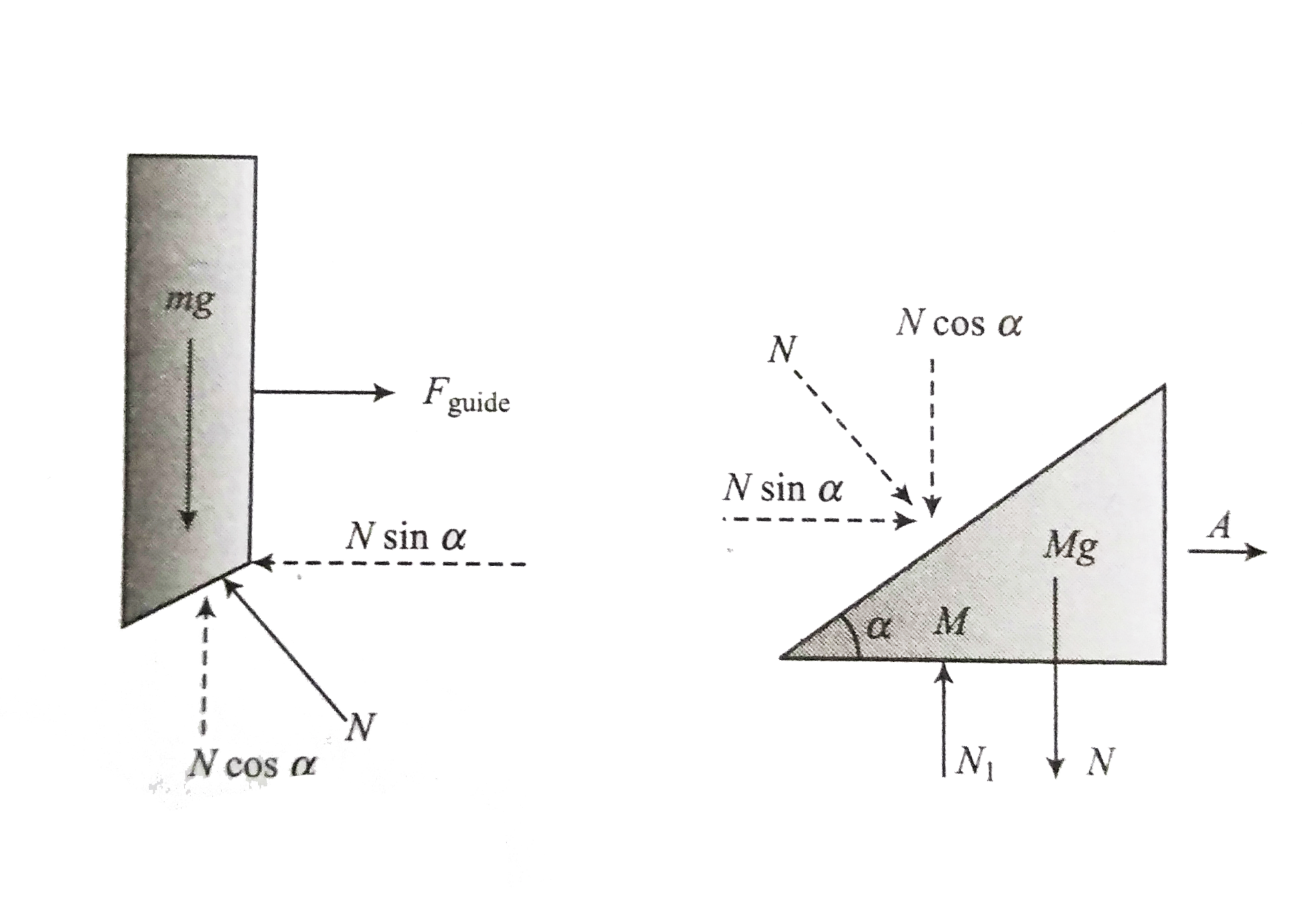
The force acting on the rod are:
 ,
,
∗ The weight mg, vertically downwars
∗ The normal force N, normal to the bottom surface of the horizontal component of N as for the rod ahorizontal=0.
Motion in vertical direction
mg−Ncosα=ma...(ii)
and the force acting on the wedge are
⋅ The weight, Mg
∗ N, reaction of N acting on the rod
∗N1, Normal force by the surface
The force equation are
N1−Mg−Ncosα=0 ...(iii)
and Nsinα=MA ...(iv)
Solving the above equation for a and A, we get
a=mgtanαmtanα+Ncotα and Amgmtanα+Mcotα.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Solved Examples
11 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.1
11 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
A plank of mass m rests symmetrically on two wedges B and C of mass M. What is the acceleration of the plank? Neglect friction between all the contact surfaces.

View SolutionFind the accelerations of rod A and wedge B in the arrangement shown in figure if the ratio of the mass of the wedge to that of the rod equals η, and the friction between all contact surfaces is negligible.

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m=5kg is placed on the wedge of mass M=32kg as shown in the figure. Find the acceleration of wedge with respect to ground. (Neglect any type of friction. Spring and pulley are ideal)
View SolutionA block of mass m is at rest on a rought wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionAll the surfaces are smooth as shown in figure. The acceleration of mass m relative to wedge is :
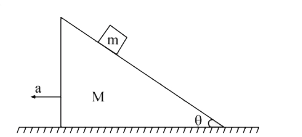
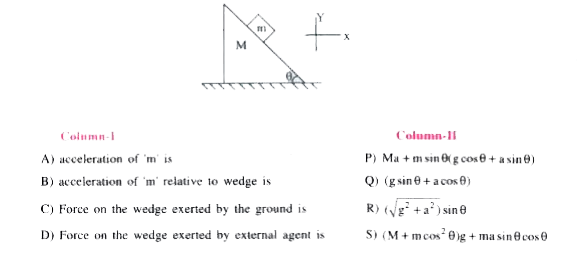
View SolutionA block of mass 'm' is kept on a smooth moving wedge. If the acceleration of the wedge is 'a'
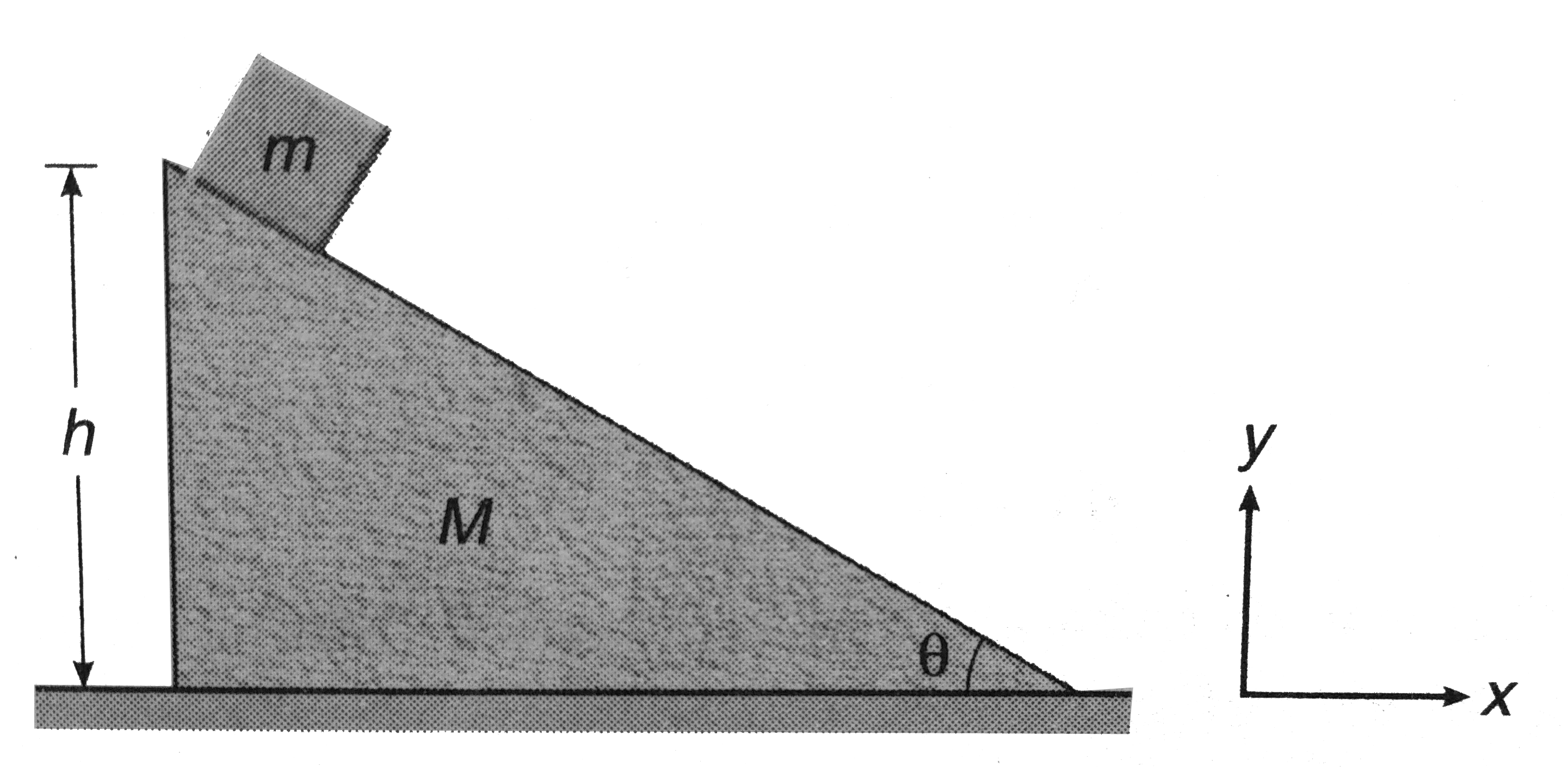
View SolutionA block of mass m is released from the top of a wedge of mass M as shown in figure. Find the displacement of wedges on the horizontal ground when the block reaches the bottom of the wedges. Neglect friction everywhere.
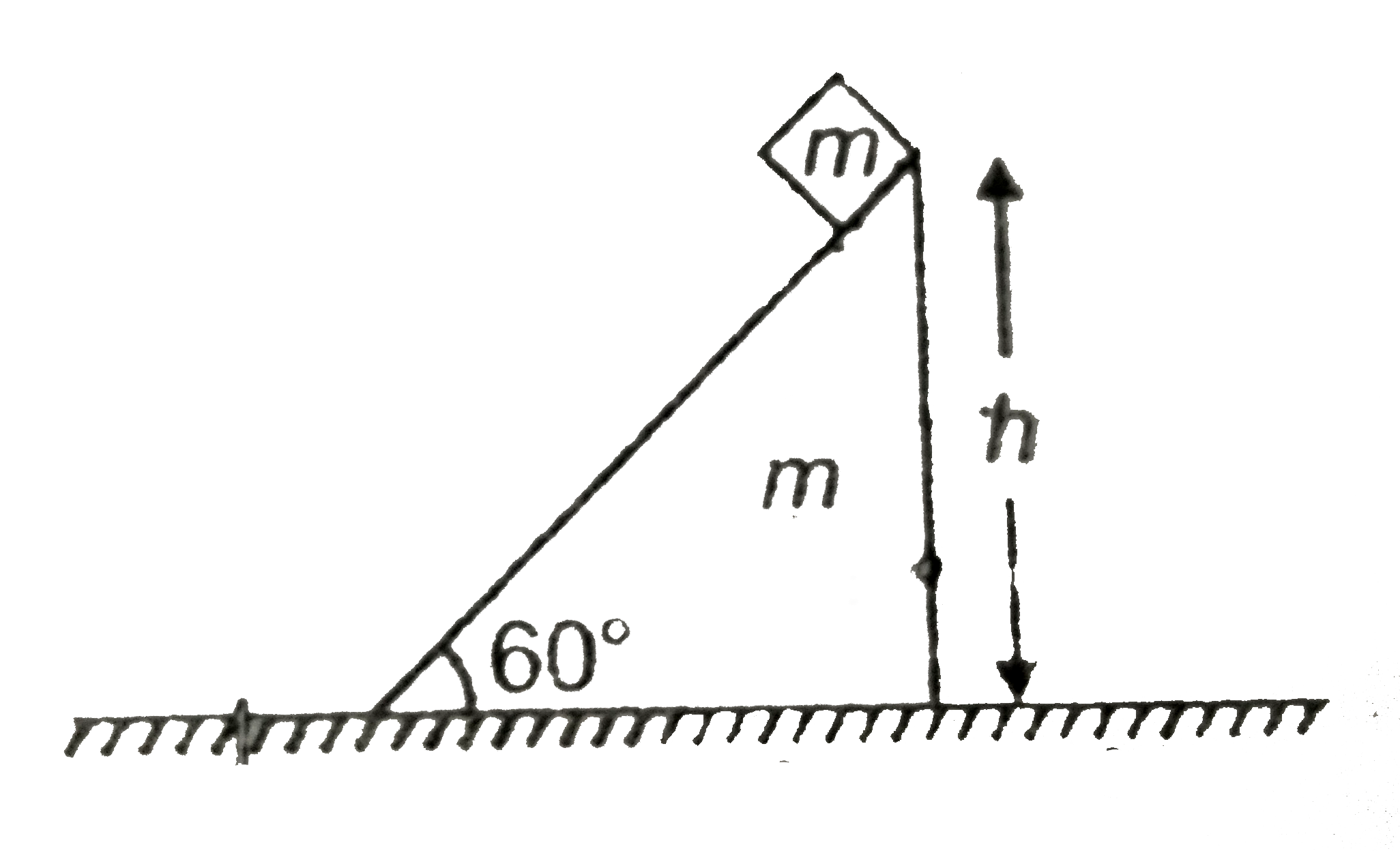
View SolutionA block of mass m is released from a wedge of mass m as shown in figure . Find the time taken by the block to reach the end of the wedge.
View Solution
