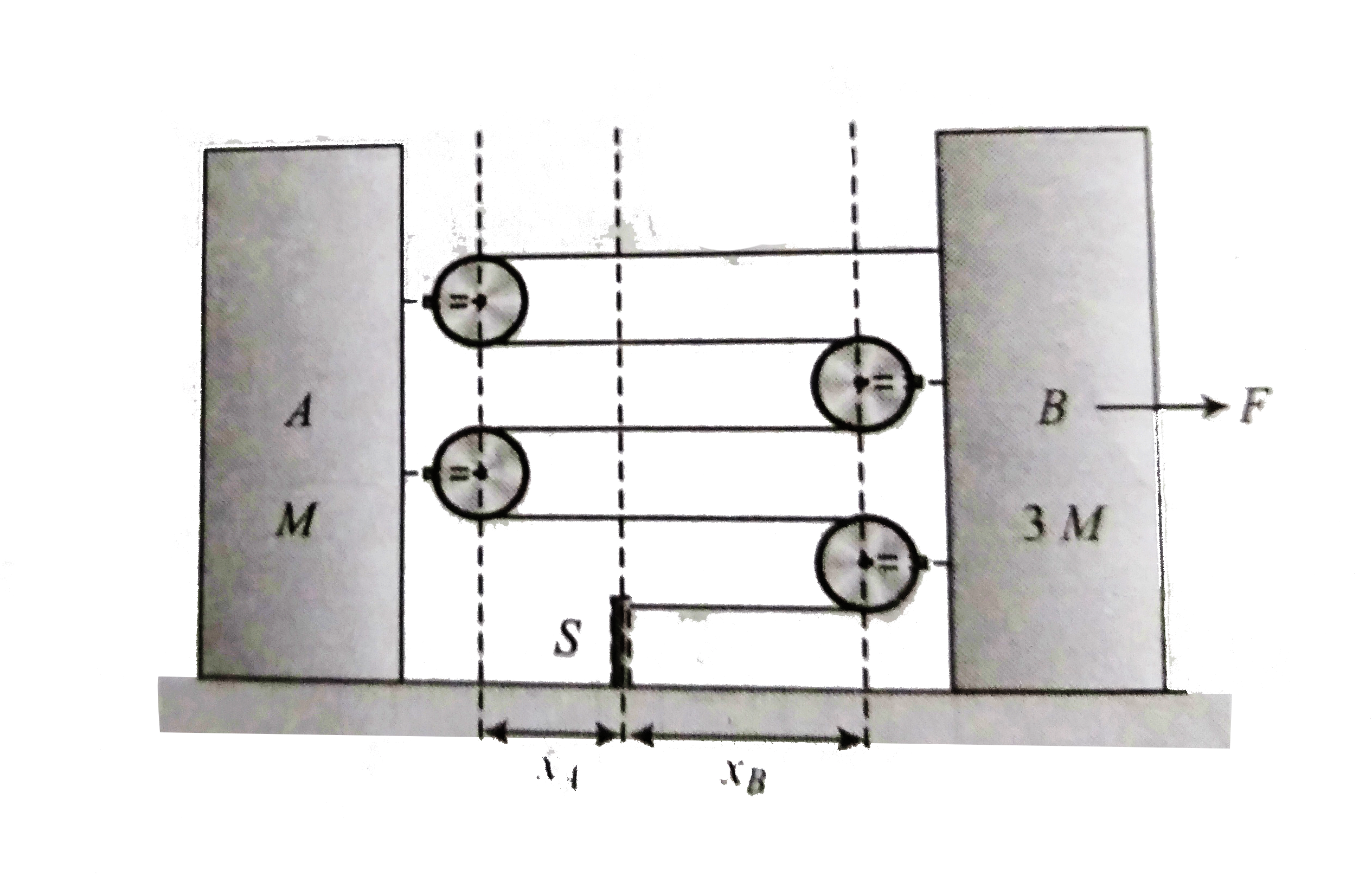
Two block A and B of mass M & 3M are connected through a light string. One end of the string is connected to the blcok B and its other end is connected to a fixed point S as shown in fig. Now a force F is applied to block B. find the acceleration of block A & B.

Taking reference line through support S let xA and xB are the distances of blocks A and B, respectively, from S. The total length of the string, l=4xA+5xB+l0. Where l0 is some part of string which is over pulley and somewhere else which remains constant.
Differentiating l w.r.t time, we get
dldt=ddt(4xA5xB+l0)
or 0=4dxadt+5dxBdt=dxAdt=54dxBdt
dxAdt=−vA (-because xA is decreasing with time)
dxBdt=−vB (+because xB is decreasing with time)
or vA=54vB,alsoaA=54aB
Method 2: charge in the length of segment I, Δl1,2=0+(xB)
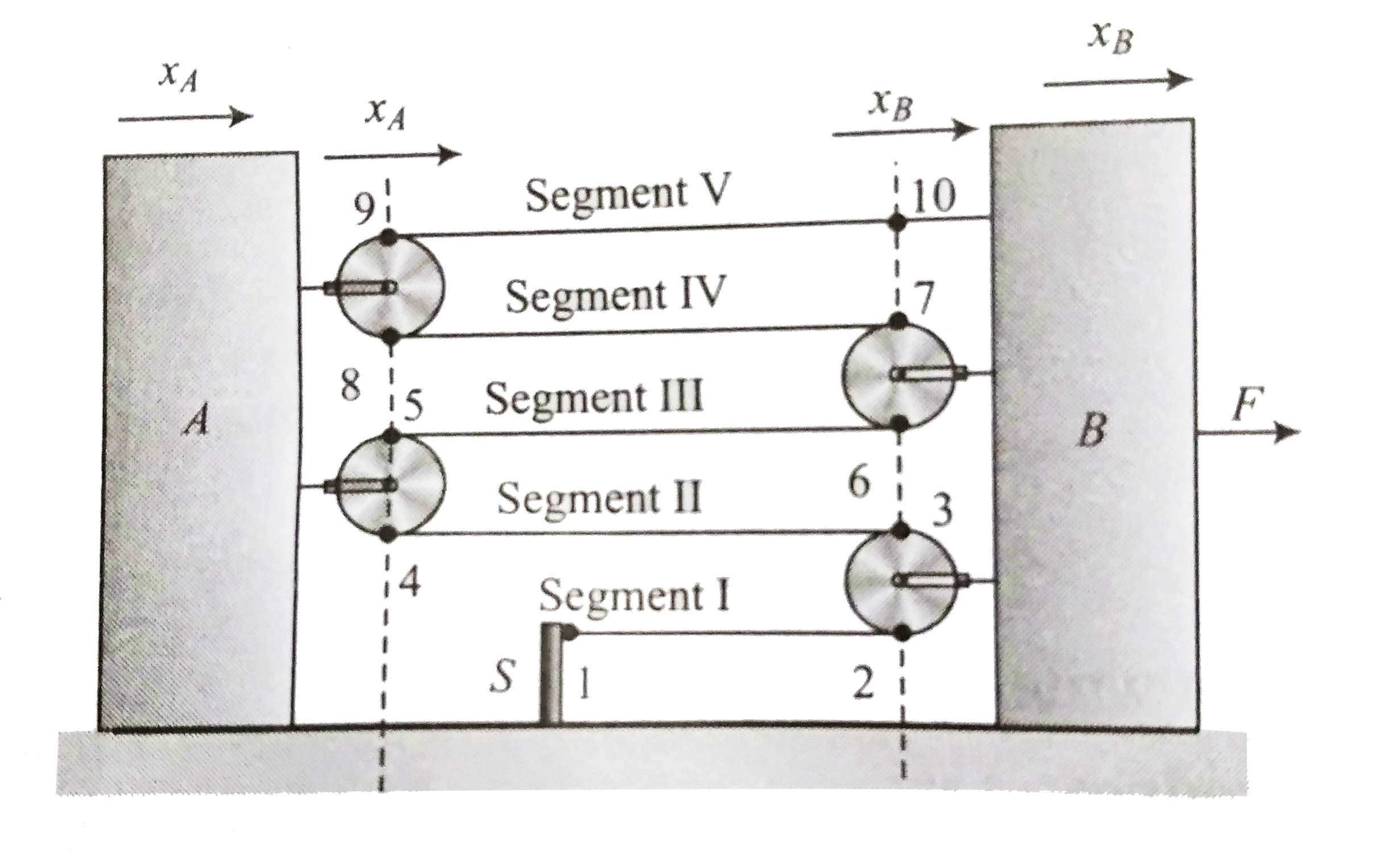
Segment II
Δl3.4=(−xA)+(xB)
Segment III
Δl5,6=(−xA)+(xB)
Segment IV
Δl7,8=(−xA)+(xB)
Segment V
Δl9,10=(−xA)+(xB)
Total charge in segment lengths should be zero. Therefore,
Δl=Δl1,2+Δl3,4+Δl5,6+Δl7,8+Δl9,10=0
=xB+[−xA+xB]+[−xA+xB]
+[−xA+xB]+[−xA+xB]=0
=−4xA+5xB=0
or xA=54xB⇒vA=54vB⇒aA=54aB
Equation of motion
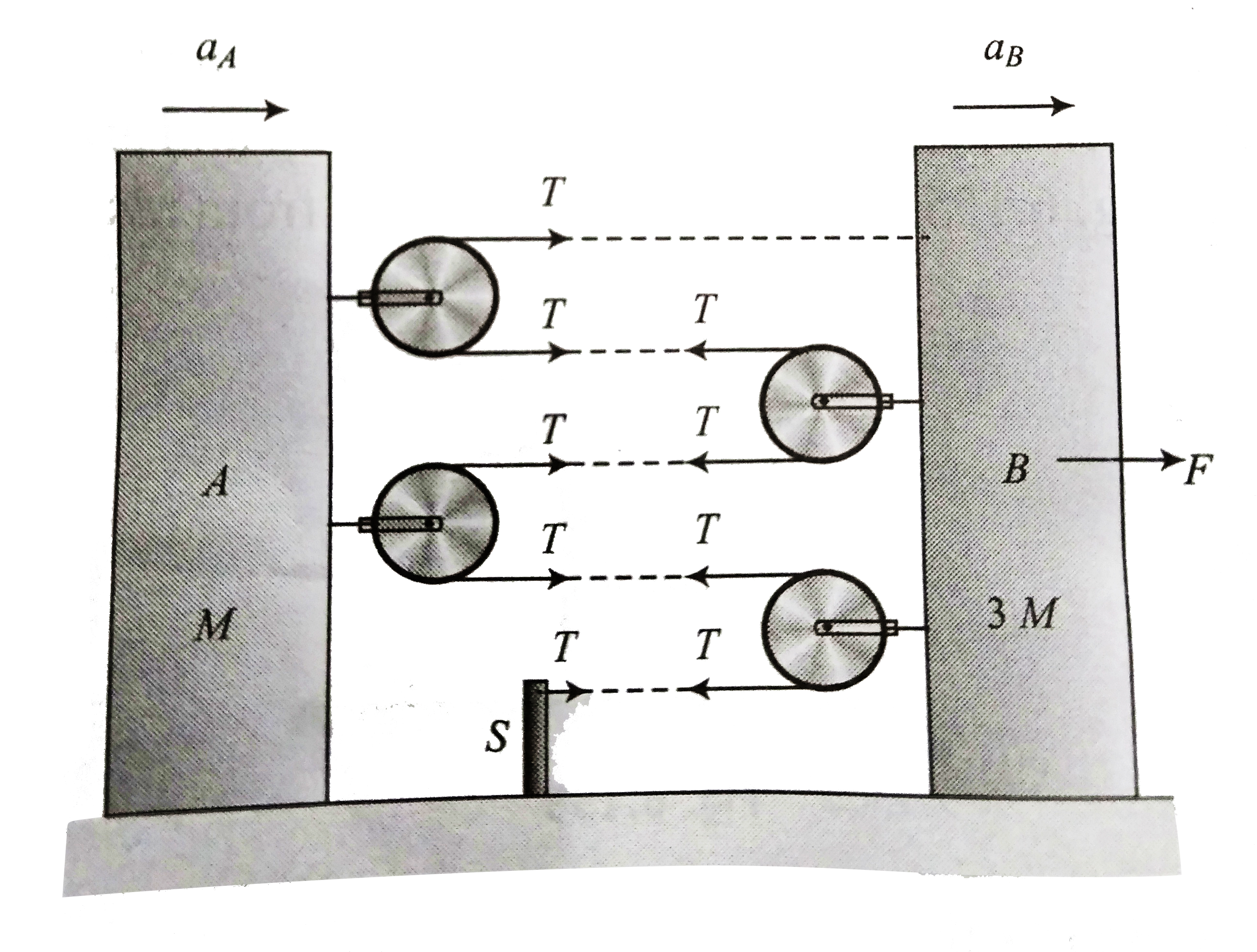
For A:
4T=mAaA=MaA...(i)
For B:
F−5T=mBaB=3MaB ..(ii)
aB=16F73
and aA=54×(16F73)=20F73.
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Solved Examples
11 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.1
11 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
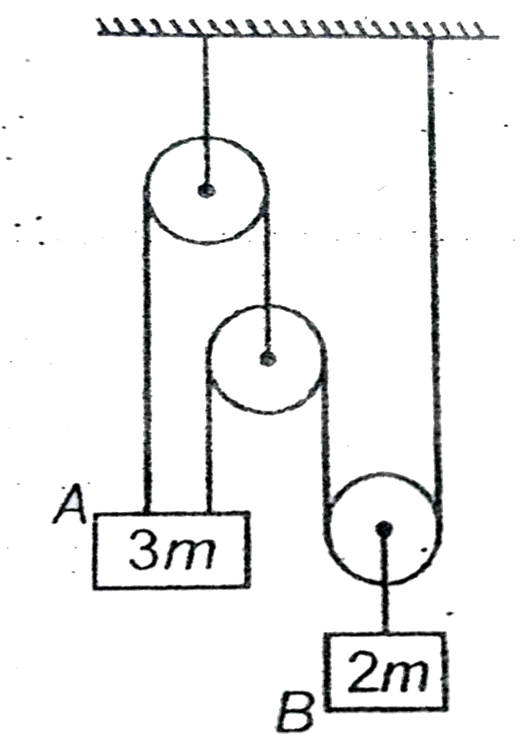
Consider the system shown in figure
Mass of block A= 3m ,
Mass of block B= 2m .
Find the acceleration of A and B.
View SolutionA block A of mass m is attached at one end of a light spring and the other end of the spring is connected to another block B of mass 2m through a light string as shown in the figure. A is held and B is in static equilibrium. Now A is released. The acceleration of A just after that instant is a . In the next case, B is held and A is in static equilibrium. Now when B is released, its acceleration immediately after the releas is b . The value of a/b is (pulley, string and the spring are massless).

View SolutionTwo identical blocks A and B each of mass M are connected to each other through a light string. The system is placed on a smooth horizontal floor. When a constant force F is applied horizontally on the block A, find the tension in the string.
View SolutionTwo blocks of masses m and M are connected by an inextensible light string . When a constant horizontal force acts on the block of mass M. The tension in the string is
View SolutionTwo block of masses m=5kgandM=10kg are connected by a string passing over a pulley B as shown. Another string connect the center of pulley B to the floor and passes over another pulley A as shown. An upwards force F is applied at the center of pulley A. Both the pulleys are massless.
Find the acceleration of block m and M, if F is
(a) 100N
(b)300N
(c)500N(Takeg=10m/s2)

View SolutionTwo unequal masses of 1kg and 2kg are connected by an inextensible light string passing over a smooth pulley as shown in figure. A force F=20N is applied on 1kg block find the acceleration of either block (g=10m/s2).

View SolutionTwo blocks A and B of same mass m attached with a light spring are suspended by a string as shown in fig. Find the acceleration of block A and B just after the string is cut.
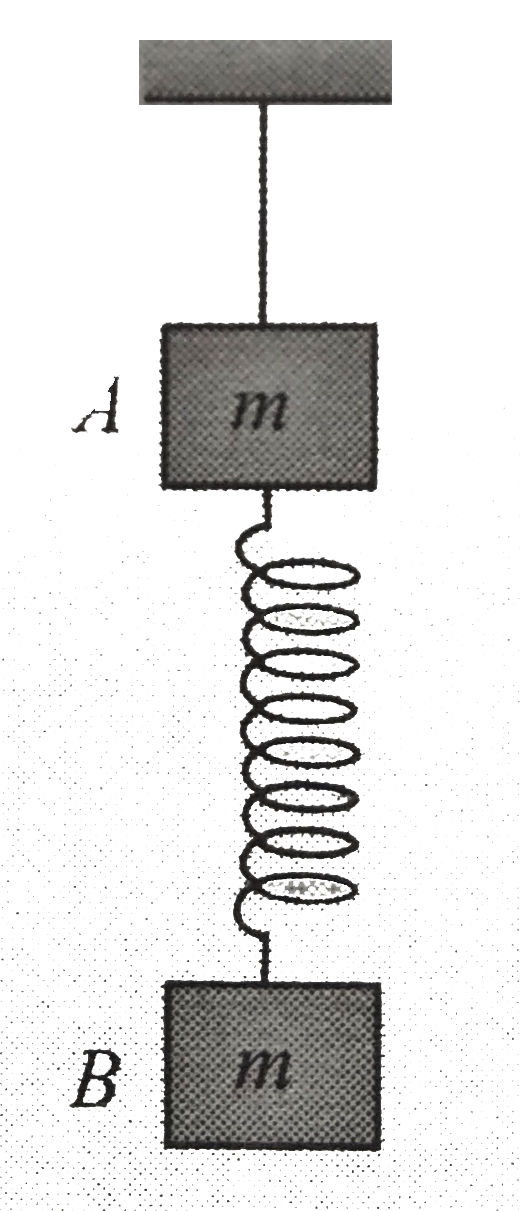
View SolutionTwo blocks of equal mass m are tied to each other through light string. One of the blocks is pulled along the line joining them with a constant force F. Find the tension in the string joining the blocks.
View Solution
Two blocks of masses m1 and m2 are connected as shown in the figure. The acceleration of the block m2 is :
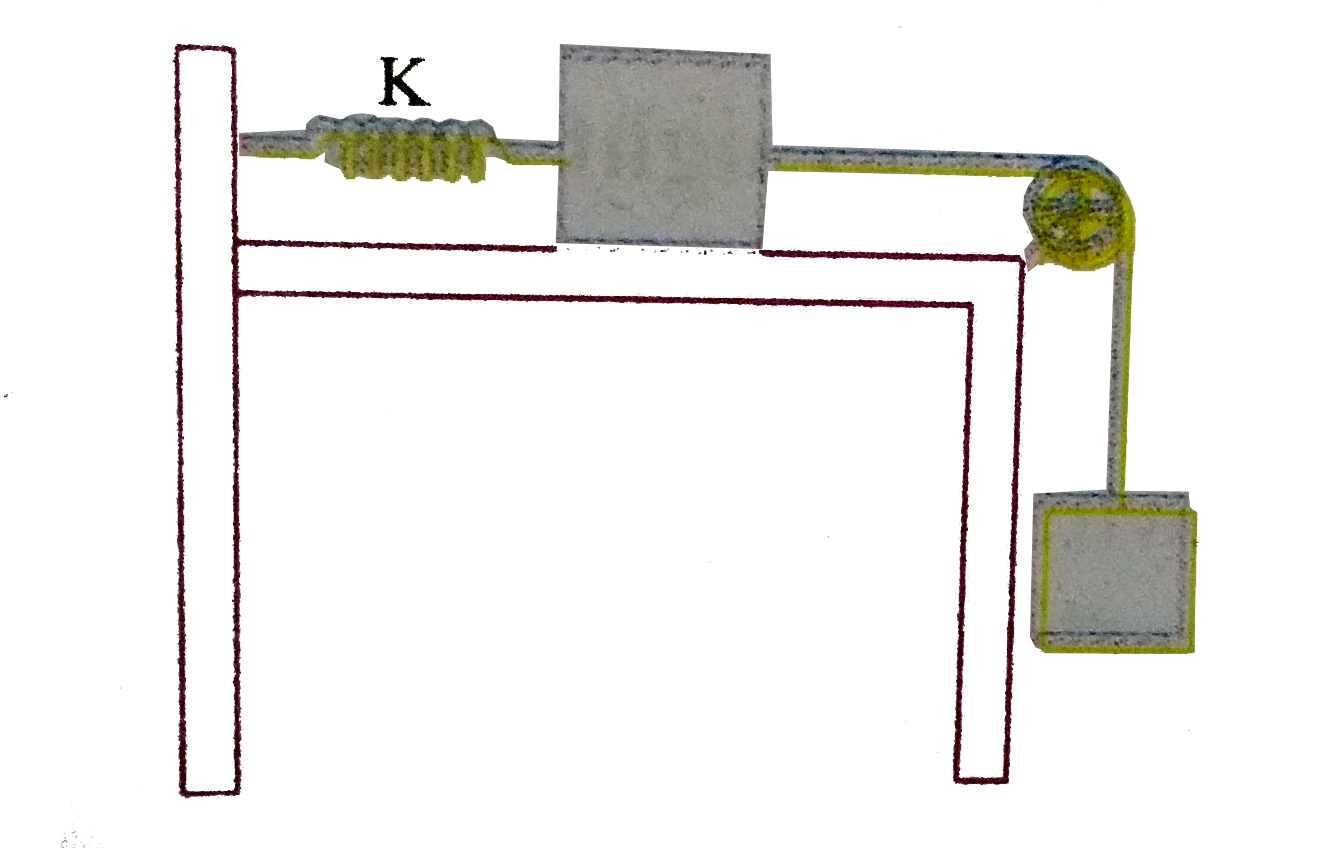
View SolutionTwo blocks, of masses M and 2M, are connected to a light spring of spring constant K that has one end fixed, as shown in figure. The horizontal surface and the pulley are frictionless. The blocks are released from when the spring is non deformed. The string is light.
 .
.
View Solution
