A 10−km long straight road connects two towns A and B, Two cyclists dimultaneously start one from town A and the other from town B .On reaching the opposite town, a cyclists simmediaeately retrns to his starting town wheras the other cyclist takes some rest and then returns to his starting town. Both of them can ride at speed 20kmh−1 in absence of wind but during their whole journey uniform wind from town A and B increase the speed of it decreases the speed of the cyclist going against the wind Both the cyclisrs meet twice, first at 2 km and then 6 km away from one of the tomns. If which town and for what perild does a cyclist rest ?
To solve the problem step by step, we will analyze the motion of the two cyclists, their speeds, and the distances they travel.
Step 1: Understand the Setup
We have two towns, A and B, connected by a 10 km road. Cyclist 1 starts from town A and Cyclist 2 starts from town B. Both cyclists ride at a speed of 20 km/h in still air. However, wind affects their speeds differently depending on their direction of travel.
Step 2: Define Variables
Let:
- v=20 km/h (speed of cyclists in still air)
- w = speed of wind (unknown)
- v1=v+w (speed of Cyclist 1 going from A to B)
- v2=v−w (speed of Cyclist 2 going from B to A)
Step 3: Analyze the Meeting Points
The cyclists meet at two points:
1. First meeting at 2 km from town A.
2. Second meeting at 6 km from town A.
Step 4: Calculate Time to First Meeting
The time taken for Cyclist 1 to reach the first meeting point (2 km) is:
t1=2v+w=220+w
The time taken for Cyclist 2 to reach the first meeting point (8 km) is:
t2=8v−w=820−w
Since they start simultaneously, we have:
t1=t2
220+w=820−w
Step 5: Solve for Wind Speed w
Cross-multiplying gives:
2(20−w)=8(20+w)
40−2w=160+8w
40−160=10w
−120=10w⟹w=−12 km/h
Step 6: Calculate Time to Second Meeting
Now, we calculate the time taken for the second meeting:
For Cyclist 1:
t3=6v+w=620−12=68=0.75 hours=45 minutes
For Cyclist 2:
t4=4v−w=420+12=432=0.125 hours=7.5 minutes
Step 7: Determine Rest Time
After reaching town B, Cyclist 1 returns immediately, while Cyclist 2 rests before returning. The total time for Cyclist 1 to reach town B and return back to the second meeting point:
Time for Cyclist 1 to B=108=1.25 hours=75 minutes
Step 8: Calculate Rest Time
Let t0 be the resting time of Cyclist 2. The total time for Cyclist 2 to reach town A and back to the second meeting point is:
t0+7.5+45=75
t0+52.5=75⟹t0=75−52.5=22.5 minutes
Final Result
Cyclist 2 rests for 22.5 minutes at town B before returning to town A.
We have two towns, A and B, connected by a 10 km road. Cyclist 1 starts from town A and Cyclist 2 starts from town B. Both cyclists ride at a speed of 20 km/h in still air. However, wind affects their speeds differently depending on their direction of travel.
Let:
- v=20 km/h (speed of cyclists in still air)
- w = speed of wind (unknown)
- v1=v+w (speed of Cyclist 1 going from A to B)
- v2=v−w (speed of Cyclist 2 going from B to A)
The cyclists meet at two points:
1. First meeting at 2 km from town A.
2. Second meeting at 6 km from town A.
The time taken for Cyclist 1 to reach the first meeting point (2 km) is:
t1=2v+w=220+w
t2=8v−w=820−w
t1=t2
220+w=820−w
Cross-multiplying gives:
2(20−w)=8(20+w)
40−2w=160+8w
40−160=10w
−120=10w⟹w=−12 km/h
Now, we calculate the time taken for the second meeting:
For Cyclist 1:
t3=6v+w=620−12=68=0.75 hours=45 minutes
t4=4v−w=420+12=432=0.125 hours=7.5 minutes
After reaching town B, Cyclist 1 returns immediately, while Cyclist 2 rests before returning. The total time for Cyclist 1 to reach town B and return back to the second meeting point:
Time for Cyclist 1 to B=108=1.25 hours=75 minutes
Let t0 be the resting time of Cyclist 2. The total time for Cyclist 2 to reach town A and back to the second meeting point is:
t0+7.5+45=75
t0+52.5=75⟹t0=75−52.5=22.5 minutes
Cyclist 2 rests for 22.5 minutes at town B before returning to town A.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.4
16 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Subjective
29 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.2
12 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A cyclist travels a distance of 1 km in the first hour, 0.5 km in the second hour and 0.3 km in the third hour. Find the average speed of the cyclist in kmh−1 .
View SolutionA cyclist travels a distance of 1 km in the first hour, 0.5 km in the second hour and 0.3 km in the third hour. Find the average speed of the cyclist in ms−1 .
View Solution
Knowledge Check
A boy goes from his house to school by bus at a speed of 20kmh−1 and returns back through the same route at a speed of 30kmh−1 . The average speed of his journey is :
A24kmh−1B25kmh−1C30kmh−1D20kmh−1
Which is the most important town in th above image ?AIdarlaBMalawaCDantraiDDhannThe distance between station A and B by road is 240 km and by train it is 300 km. A car starts from station A with a speed : km/hr whereas a train starts from station B with a speed 20 km/hr more than the speed of the car.
The speed of the car is:A60 km/hrB120 km/hrC40 km/hrD80 km/hr
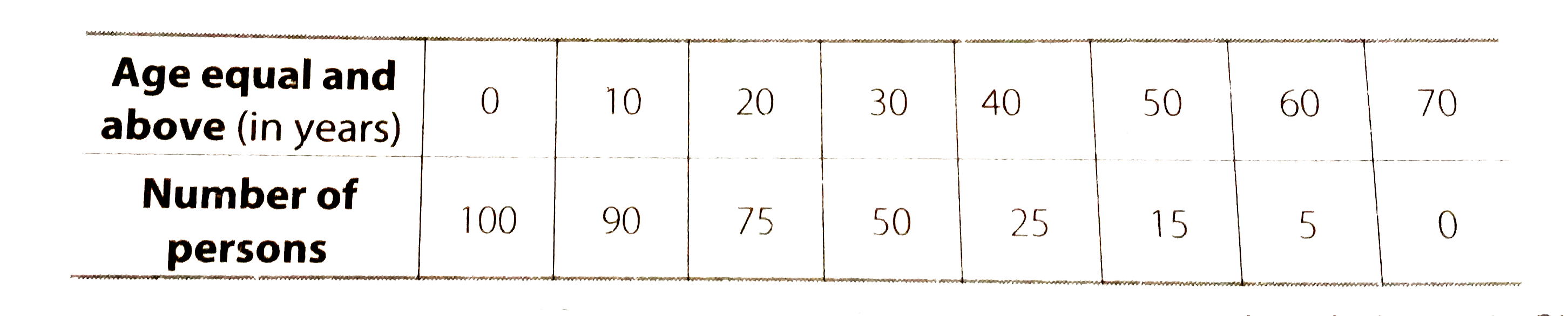
Find the mean age of 100 residents of a town from the following data.
View SolutionRam covers a distance of 100 km with variable speed. During the first half of his journey his speed was 20 km/h and during the rest of his journey his speed was 40 km/h. Calculate the average speed of Ram during the whole course of his motion.
View SolutionThe distance between two stations is 340 km. Two trains start simultaneously from these stations on parallel tracks to cross each other. The speed of one of them is greater than that of the other by 5 km/hr. If the distance between the two trains after 2 hours of their start is 30 km, find the speed of each train.
View SolutionA man travels at a speed of 20 Km/hr. and then returns at a speed of 30 Km/hr. His average speed of whole journey is
View SolutionThe population of town was 12,000 and ten years it was 16,000. What was the percent increase in the population of the town during this period?
View Solution
