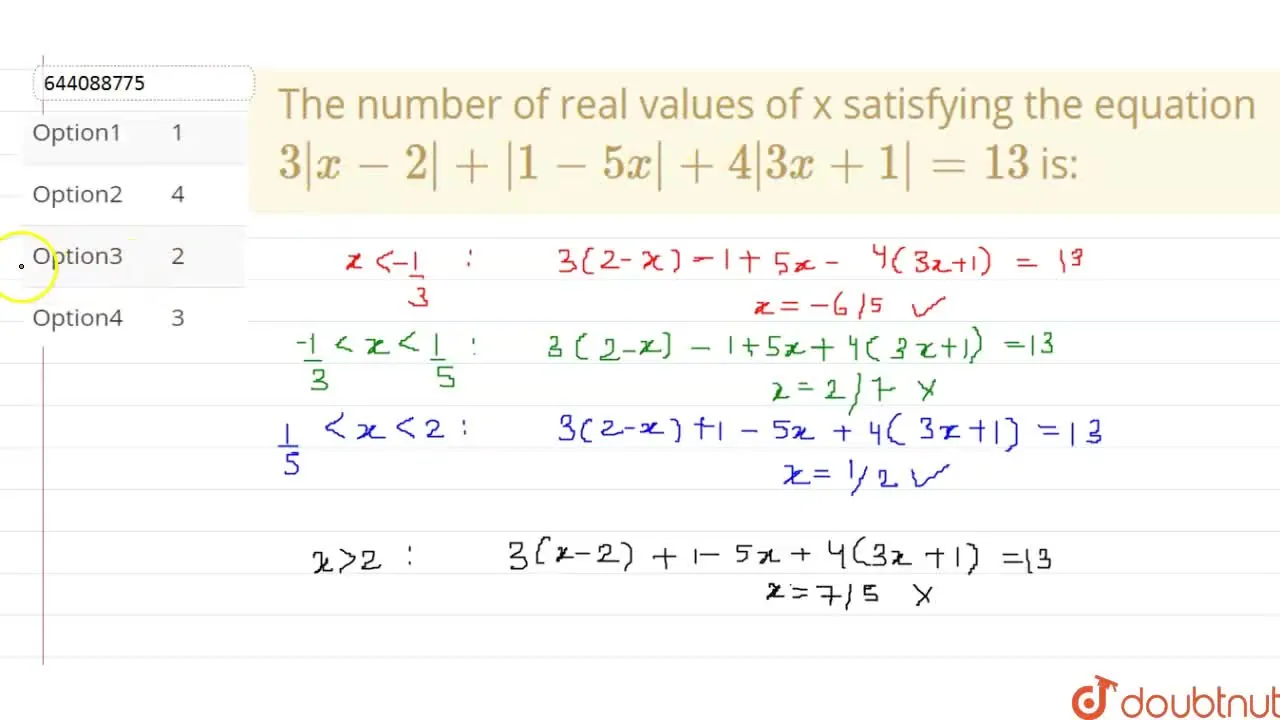
The number of real values of x satisfying the equation 3|x−2|+|1−5x|+4|3x+1|=13 is:
1
4
2
3
The correct Answer is:C
Step by step video & image solution for The number of real values of x satisfying the equation 3 |x-2| +|1-5x|+4|3x+1|=13 is: by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - VK JAISWAL ENGLISHCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (ONE OR MORE THAN ONE ANSWER IS/ARE CORRECT)42videos
QUADRATIC EQUATIONS
- BOOK - VK JAISWAL ENGLISHCHAPTER - QUADRATIC EQUATIONS EXERCISE - EXERCISE (COMPREHENSION TYPE PROBLEMS)23videos
QUADRATIC EQUATIONS
- BOOK - VK JAISWAL ENGLISHCHAPTER - PROBABILITY EXERCISE - Exercise -5 : Subjective Type problems11videos
PROBABILITY
- BOOK - VK JAISWAL ENGLISHCHAPTER - SEQUENCE AND SERIES EXERCISE - EXERCISE (SUBJECTIVE TYPE PROBLEMS)21videos
SEQUENCE AND SERIES
Similar Questions
The number of real values of x satisfying the equation 3sin−1x+πx−π=0 is/are :
View SolutionThe number of integral value(s) of x satisfying the equation ∣∣x4.3|x−2|.5x−1∣∣=−x4.3|x−2|.5x−1 is
View SolutionThe sum of all real values of X satisfying the equation (x2−5x+5)x2+4x−60=1 is:
View SolutionThe sum of all real values of x satisfying the equation (x2−5x+5)x2+4x−45=1 is :
View SolutionFind the number or real values of x satisfying the equation 92log9x+4x+3=0.
View SolutionThe number of real values of x satisfying the equation;[2x+13]+[4x+56]=3x−12 are greater than or equal to {[*] denotes greatest integer function):
View SolutionFind the sum of all real values of X satisfying the equation (x2−5x+5)x2+4x−60=1 .
View SolutionThe number of integral values of x satisfying the equation tan−1(3x)+tan−1(5x)=tan−1(7x)+tan−1(2x) is ____
View SolutionThe number of real values of x satisfying the equation
log10√1+x+3log10√1−x=2+log10√1−x2 is :View SolutionIf 0≤x≤2π, then the number of real values of x, which satisfy the equation cosx+cos2x+cos3x+cos4x=0, is
View Solution