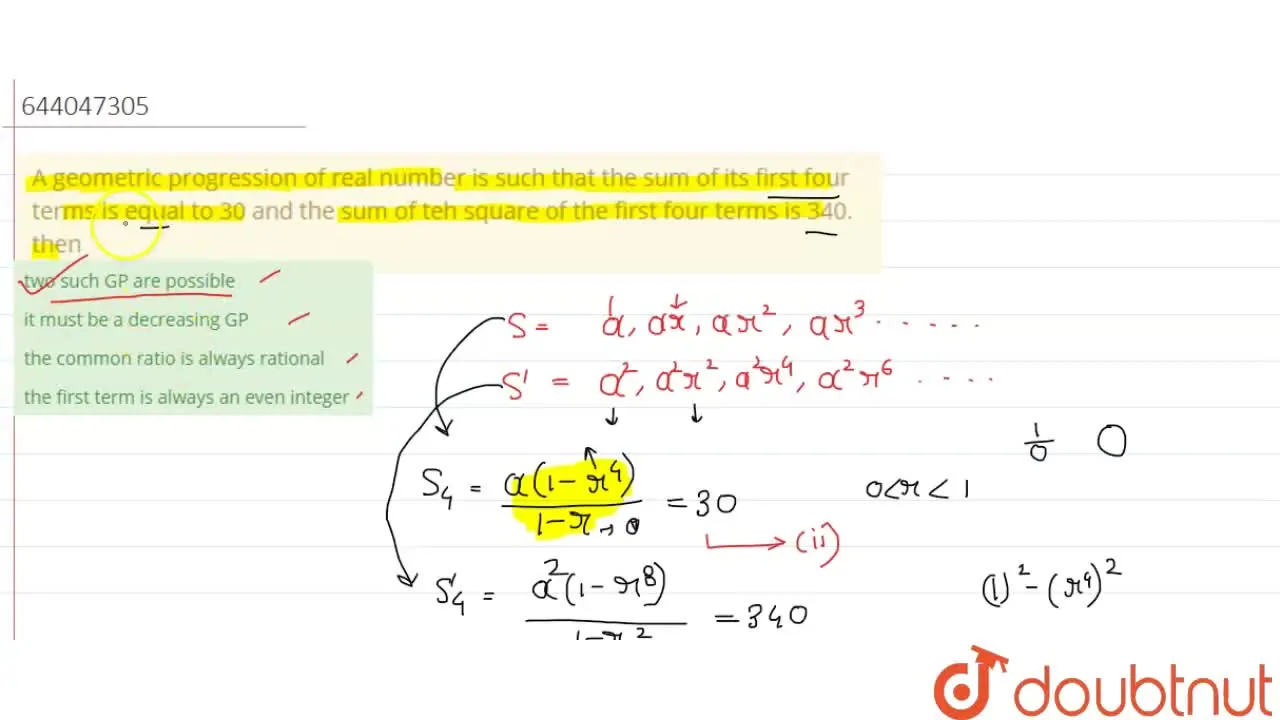
A geometric progression of real number is such that the sum of its first four terms is equal to 30 and the sum of teh square of the first four terms is 340. then Solution in Bengali
two such GP are possible
it must be a decreasing GP
the common ratio is always rational
the first term is always an even integer
The correct Answer is:1,3&4
Step by step video, text & image solution for A geometric progression of real number is such that the sum of its first four terms is equal to 30 and the sum of teh square of the first four terms is 340. then by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
Similar Questions
An um contains 30 tickets numbered 1 to 30 two tickets are drawn at random the probability that both the numbers are prime is
04:39View SolutionSolve the equation 4x2+9=0 by factorisation mathod .
03:50View SolutionIn a geometric progression,if the ratio of the sum of first 5 terms to the sum of their reciprocals is 49, and the sum of the first and the third term is 35. Then the first term of this geometric progression is
03:43View SolutionThe sum of the first four terms of a geometric series is 520. The sum of the first five terms is 844. The sum of the first six terms is 1330. (a) Find the common ratio of the geometric progression.(b) Find the sum of the first two terms.
03:38View SolutionIf the sum of the first two terms and the sum of the first four terms of a geometric progression with positive common ratio are 8 and 80 respectively, then what is the 6th term?
10:11View SolutionThe sum of an infinite geometric progression is 243 and the sum of its first five terms is 275 The first term of the progression
06:33View SolutionIn a geometric progression, the sum of first three terms is 35 and the sum of squares of the first three terms is 525. The second term of the geometric progression is
01:44View SolutionA geometric progression of real numbers is such that the sum of its first four terms is equal to 30 and the sum of the squares of the first four terms is 340. Then
03:50View SolutionIf the sum of first 11 terms of an arithmetic progression equal that of the first 19 terms, Then, what si the sum of first 30 terms?
01:32View SolutionIn a GP of real number , the sum of first four number is 30 and sum of their squares is 340 . The third term of the series is.
03:38View SolutionThe first term of an arithmetic progression is 1 and the sum of the first nine terms equal to 369. The first and the ninth term of a geometric progression coincide with the first and the ninth term of the arithmetic progression. Find the seventh term of the geometric progression.
02:09View SolutionIn a G.P. of real numbers, the sum of the first two terms is 7. The sum of the first six terms is 91. The sum of the first four terms is______________
06:21View SolutionFind the sum of the squares of first 50 natural numbers
03:20View SolutionIn an infinite geometric progression, the sum of first two terms is 6 and every terms is four times the sum of all the terms that it . Find :
(i) the geometric progression and (ii) its sum to infinity .02:02View SolutionIn an infinite geometric progression , the sum of first two terms is 6 and every term is four times the sum of all the terms that follow it . Find (i) the geometric progression and (ii) its sum of infinity .
05:18View Solution