Question

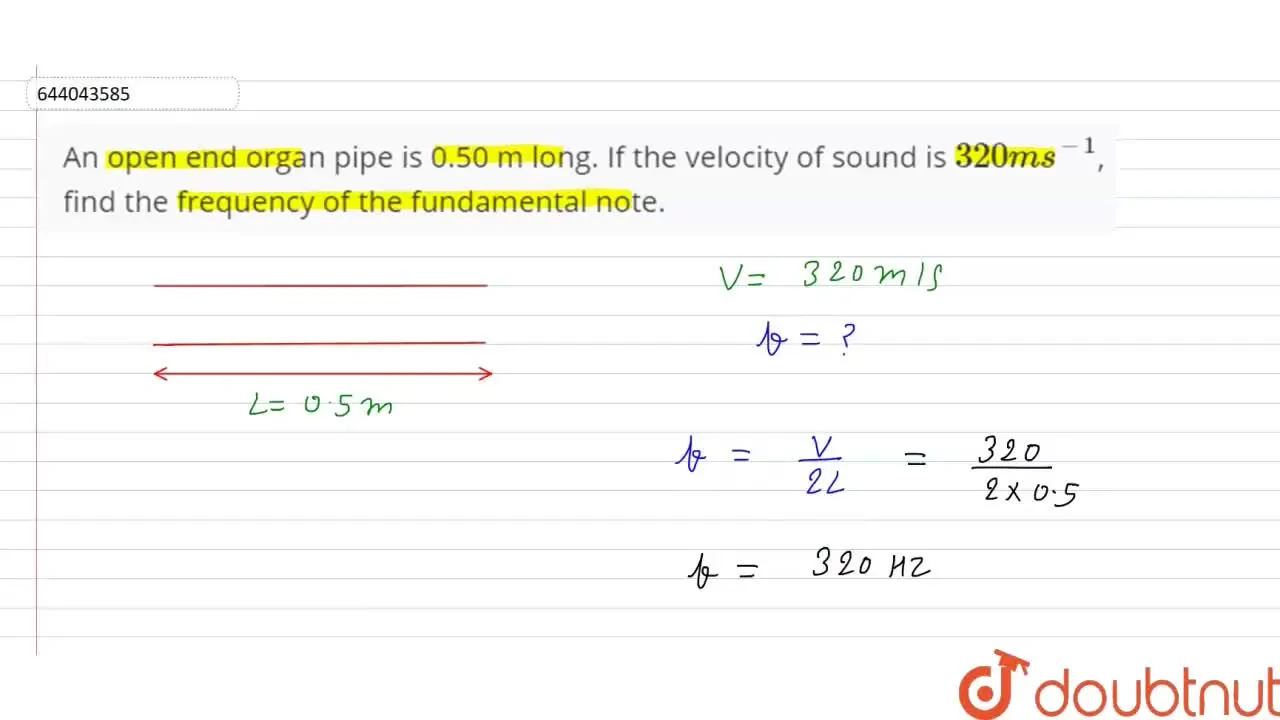
An open end organ pipe is 0.50 m long. If the velocity of sound is $320m{s}^{-1}$, find the frequency of the fundamental note.

Answer

`n=V//2l=320//2 xx 0.5=320Hz`

Explanation

To find the frequency of the fundamental note of an open-end organ pipe, we can follow these steps:

Step 1: Understand the formula for the fundamental frequency
For an open-end organ pipe, the fundamental frequency (f) is given by the formula:
\( f = \frac{v}{2L} \)
where:
- \( f \) = fundamental frequency
- \( v \) = velocity of sound in air
- \( L \) = length of the pipe

Step 2: Identify the given values
From the question, we have:
- Length of the pipe, \( L = 0.50 \, m \)
- Velocity of sound, \( v = 320 \, m/s \)

Step 3: Substitute the values into the formula
Now, we substitute the values into the formula:
\( f = \frac{320 \, m/s}{2 \times 0.50 \, m} \)

Step 4: Calculate the denominator
Calculate \( 2L \):
\( 2L = 2 \times 0.50 \, m = 1.00 \, m \)

Step 5: Calculate the frequency
Now, substitute \( 2L \) back into the frequency formula:
\( f = \frac{320 \, m/s}{1.00 \, m} = 320 \, Hz \)

Conclusion
Thus, the frequency of the fundamental note of the open-end organ pipe is:
\( f = 320 \, Hz \)

---