Name the geometrical instrument that has two legs, one with a pointed end and the other with a pencil/lead used for making geometrical constructions.
The correct Answer is:Compass
Step-by-Step Solution:
1. Identify the Instrument: The question asks for a geometrical instrument that has two legs, one with a pointed end and the other with a pencil or lead.
2. Describe the Features: The instrument has one leg that is pointed, which is used to pivot or anchor the instrument on a point. The other leg holds a pencil or lead, which is used to draw.
3. Name the Instrument: Based on the description, the instrument that fits this criteria is called a "compass."
4. Usage of the Compass: A compass is commonly used in geometry for making constructions such as drawing circles, creating angles, and bisecting lines.
Final Answer: The geometrical instrument is called a "compass."
---
Similar Questions
Knowledge Check
Write the names of the following geometrical instruments.
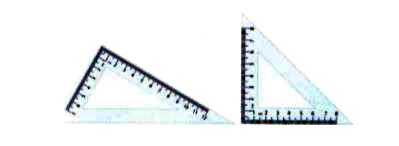 ARulersBSet squaresCProtractorsDCompasses
ARulersBSet squaresCProtractorsDCompasses
Write the names of the following geometrical instruments.
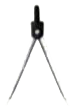
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View SolutionIf a line is drawn to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio.
View Solution

