Name the solid whose net consists of:
a. One circle and one sector
b. Six rectangles
c. Six identical squares
d. Two circles and one rectangle
The correct Answer is:a. Cone
b. Cuboid
c. Cube
d. Cylinder
Step by step video, text & image solution for Name the solid whose net consists of: a. One circle and one sector b. Six rectangles c. Six identical squares d. Two circles and one rectangle by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Updated on:21/07/2023Similar Questions
Name one d.c. source and one a.c. source.
View SolutionSee the figure and find the ratio of(a) Number of triangles to the number of circles inside the rectangle.(b) Number of squares to all the figures inside the rectangle.(c) Number of circles to all the figures inside the rectangle.
View SolutionFill in the blanks: Two square are congruent if
Two rectangles are congruent if
Two circles are congruent if
View SolutionThe figure formed by the lines ax±y±c=0 is a. a rectangle b. a square c. a rhombus d. none of these
View SolutionProve that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base. Given: A △ABC in which AB=AC and a circle is drawn by taking AB as diameter which intersects the side BC of triangle at D. To Prove: BD=DC Construction : Join AD.
View SolutionA reactangle with one side 4 cm is inscribed in a circle of radius 2.5 cm. Find the area of the rectangle
View SolutionIn the given figure ABCD is a rectangle. It consists of a circle and two semi-circles each of which are of radius 5 cm. Find the area of the shaded region. Give your answer correct to three significant figures.**
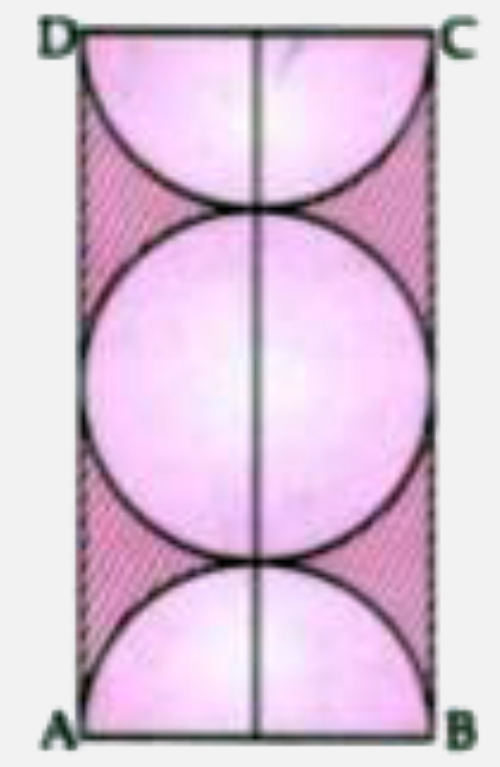
View SolutionA wire of length l is cut into two parts. One part is bent into a circle and the other into a square. Prove that the sum of the areas of the circle and the square is the least, if the radius of the circle is half of the side of the square.
View SolutionFind the area of a trapezium whose parallel sides of lengths 10cm and 15cm are at a distance of 6cm from each other. Calculate this area as (a) the sum of the areas of two triangles and one rectangle. (b) the difference of the area of a rectangle and the sum of the areas of two triangles.
View SolutionShow that if two chords of a circle bisect one another they must be diameters.
View Solution
