Write the supplement of each of the following angles:
105∘
The correct Answer is:75∘
To find the supplement of the angle 105∘, we can follow these steps:
1. Understand the Concept of Supplementary Angles:
- Supplementary angles are two angles whose sum is 180∘.
2. Identify the Given Angle:
- The given angle is 105∘.
3. Set Up the Equation:
- Let the supplement of 105∘ be x.
- According to the definition of supplementary angles, we can write the equation:
105∘+x=180∘
4. Solve for x:
- To find x, we subtract 105∘ from 180∘:
x=180∘−105∘
5. Calculate the Value:
- Perform the subtraction:
x=75∘
6. Conclusion:
- The supplement of 105∘ is 75∘.
Final Answer:
The supplement of 105∘ is 75∘.
---
- Supplementary angles are two angles whose sum is 180∘.
- The given angle is 105∘.
- Let the supplement of 105∘ be x.
- According to the definition of supplementary angles, we can write the equation:
105∘+x=180∘
- To find x, we subtract 105∘ from 180∘:
x=180∘−105∘
- Perform the subtraction:
x=75∘
- The supplement of 105∘ is 75∘.
The supplement of 105∘ is 75∘.
Topper's Solved these Questions
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Exercise 17B
13 Videos
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Exercise 17C
44 Videos
- LINES AND ANGLESBOOK - ICSECHAPTER - LINES AND ANGLESEXERCISE - Challenge
2 Videos
- LINEAR EQUATIONSBOOK - ICSECHAPTER - LINEAR EQUATIONSEXERCISE - EXERCISE 14B
27 Videos
- MENSURATIONBOOK - ICSECHAPTER - MENSURATIONEXERCISE - EXERCISE 23 G
14 Videos
Similar Questions
Knowledge Check
Write the supplement of each of the following angles:
58∘A125∘B142∘C112∘D122∘Name each of the following angles
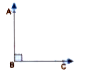 A∠BACB∠ABCC∠BCAD∠CAB
A∠BACB∠ABCC∠BCAD∠CABName each of the following angles
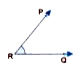 A∠QPRB∠RQPC∠RPQD∠PRQ
A∠QPRB∠RQPC∠RPQD∠PRQ
Write the supplement of each of the following angles:
62∘56'
View SolutionWrite the supplement of each of the following angles:
83∘45'30''
View SolutionWrite the supplement of each of the following angles: (i) 540 (ii) 1320 (iii) 1380
View SolutionWrite the complement of each of the following angles:
(x+12)∘
View SolutionName each of the following angles

View Solution
