Obtain an expression for magnetic flux density B at the centre of a circular coil of radius R, having N turns and carrying a current I.
To derive the expression for the magnetic flux density B at the center of a circular coil of radius R, having N turns and carrying a current I, we can follow these steps:
Step 1: Consider a small element of the coil
Let's consider a small element dl of the circular coil. The magnetic field dB at the center of the coil due to this small element can be expressed using the Biot-Savart law.
Step 2: Apply the Biot-Savart Law
According to the Biot-Savart law, the magnetic field dB due to a current element I is given by:
dB=μ0I4πdlr2
where:
- μ0 is the permeability of free space,
- I is the current,
- dl is the length of the current element,
- r is the distance from the current element to the point where the field is being calculated (which is the center of the coil).
Step 3: Determine the distance r
For a circular coil of radius R, the distance r from any point on the coil to the center is simply R.
Step 4: Substitute r into the equation
Substituting r=R into the equation for dB:
dB=μ0I4πdlR2
Step 5: Integrate over the entire coil
To find the total magnetic field B at the center, we need to integrate dB over the entire coil. The total length of the coil is 2πR (the circumference of the coil). Thus, we have:
B=∫dB=∫μ0I4πdlR2
Since dl integrates to the total length of the coil:
B=μ0I4πR2∫2πR0dl=μ0I4πR2(2πR)
Step 6: Simplify the expression
Now, simplifying the expression:
B=μ0I4πR2⋅2πR=μ0I2R
Step 7: Consider the number of turns
Since the coil has N turns, the total magnetic field Bt at the center due to N turns is:
Bt=N⋅B=N⋅μ0I2R
Final Expression
Thus, the expression for the magnetic flux density B at the center of a circular coil of radius R, having N turns and carrying a current I is:
B=Nμ0I2R
Let's consider a small element dl of the circular coil. The magnetic field dB at the center of the coil due to this small element can be expressed using the Biot-Savart law.
According to the Biot-Savart law, the magnetic field dB due to a current element I is given by:
dB=μ0I4πdlr2
where:
- μ0 is the permeability of free space,
- I is the current,
- dl is the length of the current element,
- r is the distance from the current element to the point where the field is being calculated (which is the center of the coil).
For a circular coil of radius R, the distance r from any point on the coil to the center is simply R.
Substituting r=R into the equation for dB:
dB=μ0I4πdlR2
To find the total magnetic field B at the center, we need to integrate dB over the entire coil. The total length of the coil is 2πR (the circumference of the coil). Thus, we have:
B=∫dB=∫μ0I4πdlR2
Since dl integrates to the total length of the coil:
B=μ0I4πR2∫2πR0dl=μ0I4πR2(2πR)
Now, simplifying the expression:
B=μ0I4πR2⋅2πR=μ0I2R
Since the coil has N turns, the total magnetic field Bt at the center due to N turns is:
Bt=N⋅B=N⋅μ0I2R
Thus, the expression for the magnetic flux density B at the center of a circular coil of radius R, having N turns and carrying a current I is:
B=Nμ0I2R
Similar Questions
(a) Using Biot-Savart's law, derive an expression for the magnetic field at the centre of a circular coil of radius R, number of turns N, carrying current.
(b) Two small identical circular coils marked 1,2 carry equal currents and are placed with their geometric axes perpendicular to each other as shown in the figure. Derive an expression for the resultant magnetic field at O.
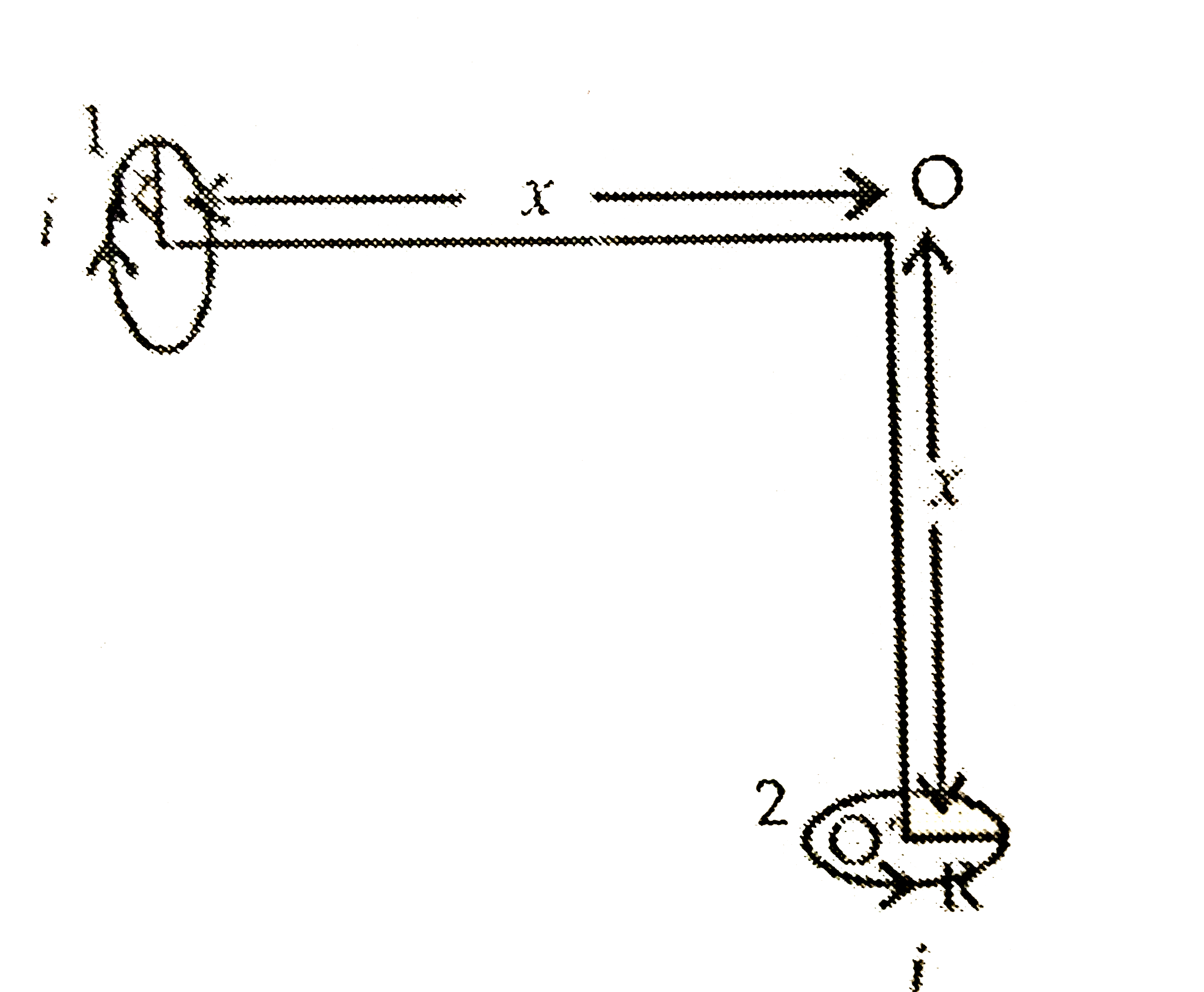
View SolutionCalculate magnetic flux density of the magnetic field at the centre of a circular coil of 50 turns, having radius of 0.5 m and carrying a current of 5A.
View Solution
Knowledge Check
The magnetic moment of a current I carrying circular coil of radius r and number of turns N varies as
A1r2B1rCrDr2
Find magnetic flux density at a point on the axis of a long solenoid having 5000 turns/m when it carrying a current of 2 A
View Solution(a) Write the expression for the equivalent megnetic moment of a planer current loop of area A, having N turns and carrying a current i. Use the expression to find the magentic dipole moment of a revolving electron ?
(b) A circuit loop of radius r, having N turns and carrying current I, is kept in the XY plane. It is then subjected to a uniform magnetic field →B=Bxˆi+Byˆj+Bzˆk . Obtain expression for the magnetic potential energy of the coil-magnetic field system.
View SolutionMagnetic field intensity at the centre of coil of 50 turns, radius 0.5m and carrying a current of 2A is
View SolutionThe magnetic field at the centre of the current carrying coil is
View SolutionThe magneitc field produced at the center of a current carrying circular coil of radius r, is
View Solution(a) State Biot - Savart law and ecpress it in the vector form.
(b) Using Biot - Savart law, law obtain the expression for the magnetic field due to a circular coil of radius r, carrying a current I at point on its axis distant x from the centre of the coil.
View SolutionUsing Ampere.s circuital law, obtain an expression for the magnetic flux density .B. at a point .X. at a perpendicular distance .r. from a long current carrying conductor. (Statement of the law is not required).
View Solution
