A shot fired from cannon explodes in air. What will be the changes in the momentum and the kinetic energy ?
To analyze the changes in momentum and kinetic energy when a shot fired from a cannon explodes in the air, we can break down the problem into clear steps:
Step 1: Understand the Initial Conditions
When the cannon fires the shot, it has an initial momentum and kinetic energy. The momentum (p) of the shot can be expressed as:
pinitial=MV
where M is the mass of the shot and V is its velocity. The initial kinetic energy (KE) can be expressed as:
KEinitial=12MV2
Hint: Remember that momentum is the product of mass and velocity, while kinetic energy depends on the mass and the square of the velocity.
Step 2: Analyze the Explosion
When the shot explodes, it breaks into smaller pieces. Importantly, no external forces are acting on the system (the shot and the pieces), so the total momentum of the system must be conserved.
Hint: Conservation of momentum means that the total momentum before the explosion equals the total momentum after the explosion.
Step 3: Apply Conservation of Momentum
Since momentum is conserved, we can state that:
pinitial=pfinal
This means that the momentum of the pieces after the explosion will equal the initial momentum of the shot. The pieces may have different velocities, but their combined momentum will still equal MV.
Hint: Think of how the pieces might move in different directions but still add up to the same total momentum.
Step 4: Analyze the Kinetic Energy After the Explosion
After the explosion, the kinetic energy of the system changes. The explosion releases energy, which increases the kinetic energy of the fragments. Therefore, the kinetic energy after the explosion will be greater than the initial kinetic energy:
KEfinal>KEinitial
Hint: The explosion adds energy to the system, which is why the kinetic energy increases.
Step 5: Conclusion
In summary, after the explosion:
- The momentum of the system is conserved (remains the same).
- The kinetic energy of the system increases due to the explosion.
Final Answer
- Change in Momentum: Momentum is conserved.
- Change in Kinetic Energy: Kinetic energy increases.
When the cannon fires the shot, it has an initial momentum and kinetic energy. The momentum (p) of the shot can be expressed as:
pinitial=MV
where M is the mass of the shot and V is its velocity. The initial kinetic energy (KE) can be expressed as:
KEinitial=12MV2
When the shot explodes, it breaks into smaller pieces. Importantly, no external forces are acting on the system (the shot and the pieces), so the total momentum of the system must be conserved.
Since momentum is conserved, we can state that:
pinitial=pfinal
This means that the momentum of the pieces after the explosion will equal the initial momentum of the shot. The pieces may have different velocities, but their combined momentum will still equal MV.
After the explosion, the kinetic energy of the system changes. The explosion releases energy, which increases the kinetic energy of the fragments. Therefore, the kinetic energy after the explosion will be greater than the initial kinetic energy:
KEfinal>KEinitial
In summary, after the explosion:
- The momentum of the system is conserved (remains the same).
- The kinetic energy of the system increases due to the explosion.
- Change in Momentum: Momentum is conserved.
- Change in Kinetic Energy: Kinetic energy increases.
Topper's Solved these Questions
- SAMPLE QUESTION PAPER - 01 Book:ICSEChapter:SAMPLE QUESTION PAPER - 01 Exercise:SECTION - C
Explore 14 Videos
- SAMPLE QUESTION PAPER - 01 Book:ICSEChapter:SAMPLE QUESTION PAPER - 01 Exercise:SECTION - D
Explore 12 Videos
- SAMPLE QUESTION PAPER - 01 Book:ICSEChapter:SAMPLE QUESTION PAPER - 01 Exercise:SECTION - D
Explore 12 Videos
- PROPERTIES OF MATTERBook:ICSEChapter:PROPERTIES OF MATTERExercise:MODULE 4 ( TEMPERATURE ) UNSOLVED PROBLEMS
Explore 12 Videos
- SAMPLE QUESTION PAPER 4Book:ICSEChapter:SAMPLE QUESTION PAPER 4Exercise:SECTION - D (Answer all questions.)
Explore 7 Videos
Similar Questions
Knowledge Check
the kinetic energy of the air is
A12AρvtB12Aρv2tC12Aρv3tD2Aρv3t
A rocket explodes in mid air. What happens to its total momentum and total KE?
View SolutionA particle of mass m has momentum p. Its kinetic energy will be
View SolutionA rifle man, who together with his rifle has a mass of 100kg, stands on a smooth surface and fires 10 shots horizontally. Each bullet. has a mass 10g and a muzzle velocity of 800m/s,
a. What velocity does the rifle man acquire at the end of 10 shots?
b. If the shots are fired in 10s, what will he the average force exerted on him?
c. Compare his kinetic energy with that of 10 bullets
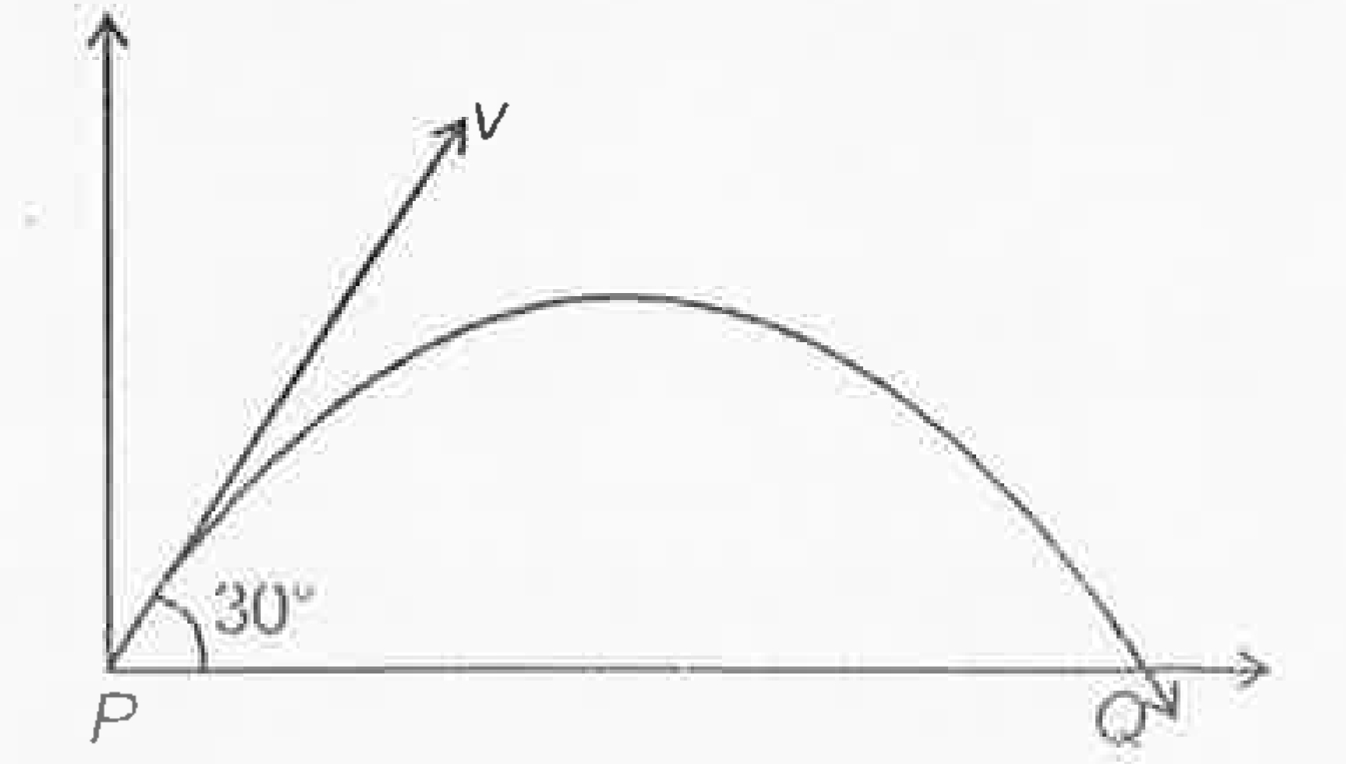
View SolutionA projectile of a mass m is fired with velocity v from point a as shown in figure neglecting air resistance, what is the change in momentum in x direction when leaving from P and arriving at Q?
View SolutionWhat is the kinetic energy of an object ?
View SolutionWhen a cannon shell explodes in mid air, then identify the incorrect statement
View SolutionA body explodeds in mid - air. Does its momentum remain conserved ?
View Solution