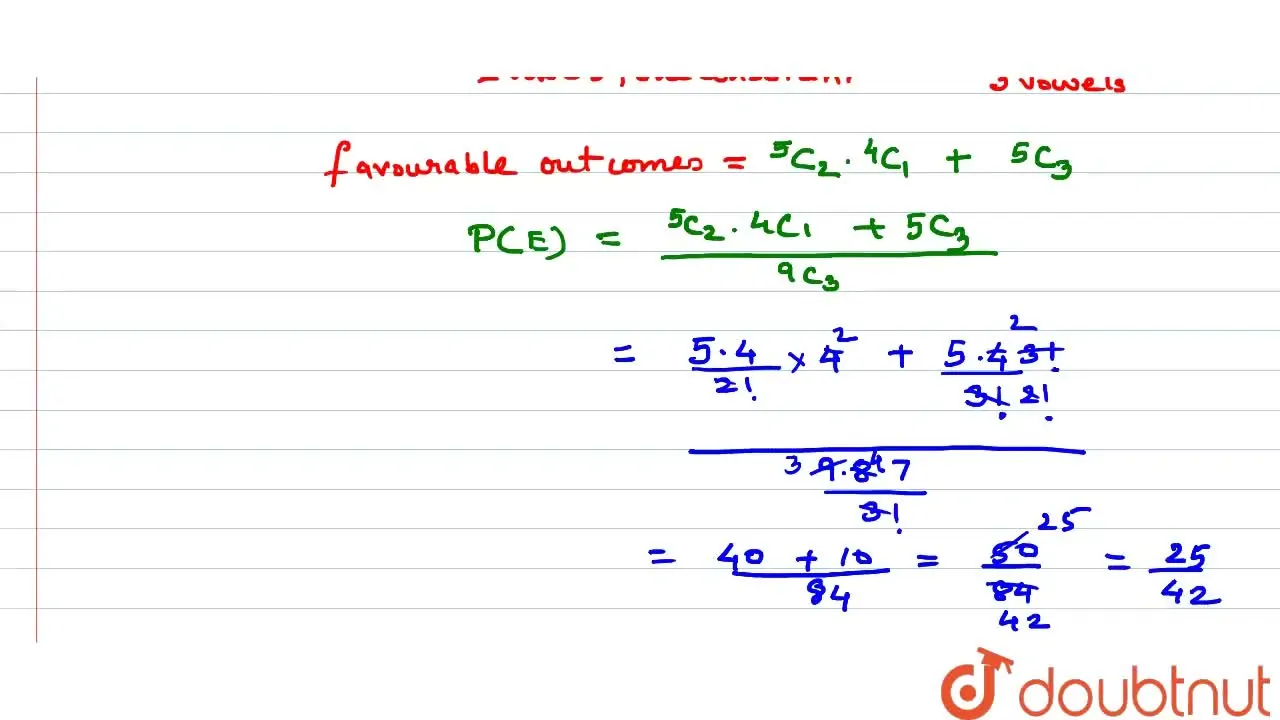
A word consists of 9 different alphabets, in which there are 4 consonants and 5 vowels. Three alphabets are chosen at random. What is the probability that more than one vowel is selected?
Step by step video & image solution for A word consists of 9 different alphabets, in which there are 4 consonants and 5 vowels. Three alphabets are chosen at random. What is the probability that more than one vowel is selected? by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 2EXERCISE - SECTION-B10videos
SELF ASSESSMENT PAPER 2
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 2EXERCISE - Section - C12videos
SELF ASSESSMENT PAPER 2
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 10EXERCISE - SECTION B3videos
SELF ASSESSMENT PAPER 10
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 9EXERCISE - SECTION C10videos
SELF ASSESSMENT PAPER 9
Similar Questions
A word consists of 9 different alphabets, in which there are 4 consonants and 5 vowels. Three alphabets are chosen at random. What is the probability that more than one vowel will be selected ?
View SolutionA word consist of 9 letters; 5 consonants and 4 vowels. Three letters are chosen at random. What is the probability that more than one vowel will be selected?
View SolutionA word consists of 9 letters. 5 consonants and 4 vowels. Three letters are chosen at random. What is the probability that more than one vowel will be selected ?
View SolutionA word consists of 7 letters : 4 consonants and 3 vowels. If three letters are chosen at random, then the probability that more than one vowel will be selected, is
View SolutionThe letters of SOCIETY are placed at random in a row. What is the probability that three vowels come together?
View SolutionA letter of English alphabet is chosen at random. Determine the probability that the chosen letter is a consonant.
View SolutionA letter of English alphabet is chosen at random. Determine the probability that the chosen letter is a consonant.
View SolutionA letter is chosen from the word 'TRIANGLE'. What is the probability that it is a vowel?
View SolutionA bag has 10 green 5 white, and 4 red balls. One ball is drawn at random. What is the probability that it is white?
View SolutionA bag has 10 green, 5 white, and 4 green balls. One ball is drawn at random. What is the probability that it is white?
View Solution