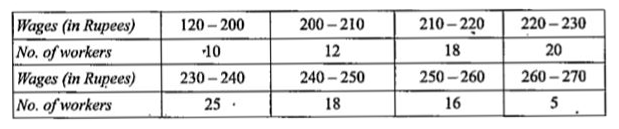
For a group of 50 male workers, the mean and standard deviations of their daily wages are ₹ 63 and ₹ 9 respectively for a group of 40 female workers, these are ₹ 54 and ₹ 6 respectively. Find the mean and standard deviation for the combined group.
The correct Answer is:9
To find the combined mean and standard deviation for the group of male and female workers, we will follow these steps:
Step 1: Calculate the Combined Mean
The formula for the combined mean ¯X of two groups is given by:
¯X=nm⋅¯Xm+nf⋅¯Xfnm+nf
Where:
- nm = number of male workers = 50
- ¯Xm = mean wage of male workers = ₹ 63
- nf = number of female workers = 40
- ¯Xf = mean wage of female workers = ₹ 54
Substituting the values:
¯X=50⋅63+40⋅5450+40
Calculating the numerator:
50⋅63=3150
40⋅54=2160
3150+2160=5310
Now, calculating the denominator:
50+40=90
Now, substituting back into the formula:
¯X=531090=59
Step 2: Calculate the Combined Standard Deviation
The formula for the combined standard deviation σ is:
σ=
⎷nm⋅σ2m+nf⋅σ2f+nm⋅(d2m)+nf⋅(d2f)nm+nf
Where:
- σm = standard deviation of male workers = ₹ 9
- σf = standard deviation of female workers = ₹ 6
- dm = deviation of male mean from combined mean = |¯X−¯Xm|=|59−63|=4
- df = deviation of female mean from combined mean = |¯X−¯Xf|=|59−54|=5
Calculating σ2m and σ2f:
σ2m=92=81
σ2f=62=36
Now substituting into the formula:
σ=√50⋅81+40⋅36+50⋅42+40⋅5290
Calculating each term:
50⋅81=4050
40⋅36=1440
50⋅16=800(since 42=16)
40⋅25=1000(since 52=25)
Now summing these:
4050+1440+800+1000=7290
Now substituting back into the formula:
σ=√729090=√81=9
Final Results
- Combined Mean = ₹ 59
- Combined Standard Deviation = ₹ 9
- nm = number of male workers = 50
- ¯Xm = mean wage of male workers = ₹ 63
- nf = number of female workers = 40
- ¯Xf = mean wage of female workers = ₹ 54
40⋅54=2160
3150+2160=5310
⎷nm⋅σ2m+nf⋅σ2f+nm⋅(d2m)+nf⋅(d2f)nm+nf
- σm = standard deviation of male workers = ₹ 9
- σf = standard deviation of female workers = ₹ 6
- dm = deviation of male mean from combined mean = |¯X−¯Xm|=|59−63|=4
- df = deviation of female mean from combined mean = |¯X−¯Xf|=|59−54|=5
σ2f=62=36
40⋅36=1440
50⋅16=800(since 42=16)
40⋅25=1000(since 52=25)
- Combined Standard Deviation = ₹ 9
Topper's Solved these Questions
Similar Questions
Knowledge Check
If the mean and standard deviation of a binomial distribution are 12 and 2 respectively , then the value of its parameter p is
A14B13C12D23
Find the mean and standard deviation using short cut method

View SolutionThe mean and standard deviation of a binomial variate X are 4 and √3 respectively. Then ,P(X≥1)=
View SolutionFind the mean and standard deviation of the following frequency distribution :
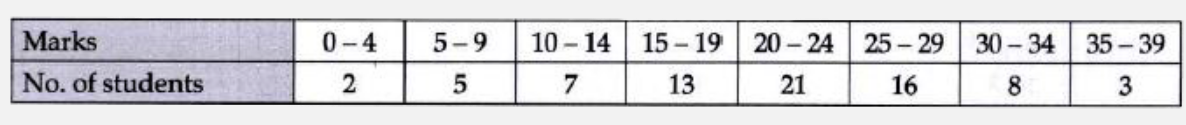
View SolutionFind the mean variance and standard deviation using short-cut method
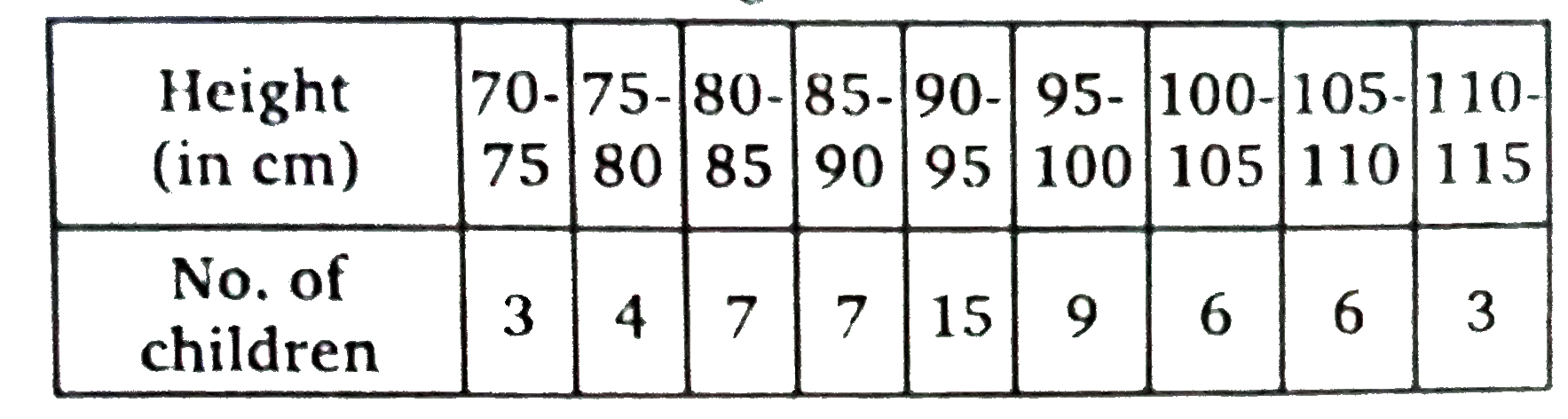
View SolutionFind the mean, variance and standard deviation using short-cut method.
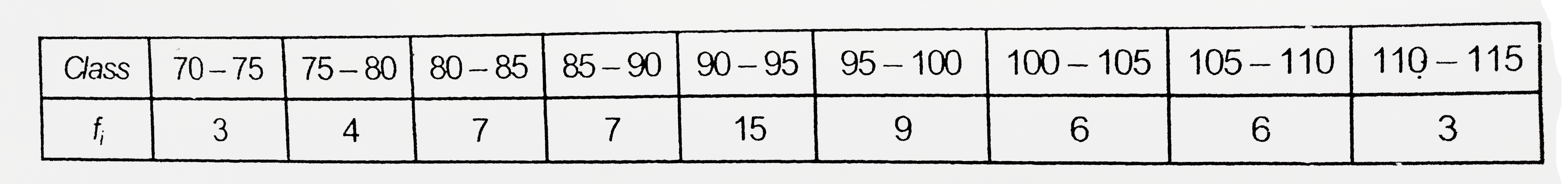
View SolutionFor a group of 200 candidates the mean and standard deviation were found to be 40 and 15 repectively . Later on it was found that the score 43 was misread as 34. Find the correct mean and standard deviation.
View SolutionThe mean and standard deviation of six observations are 8 and 4, respectively. If each observation is multiplied by 3, find the new mean and new standard deviation of the resulting observations.
View Solution