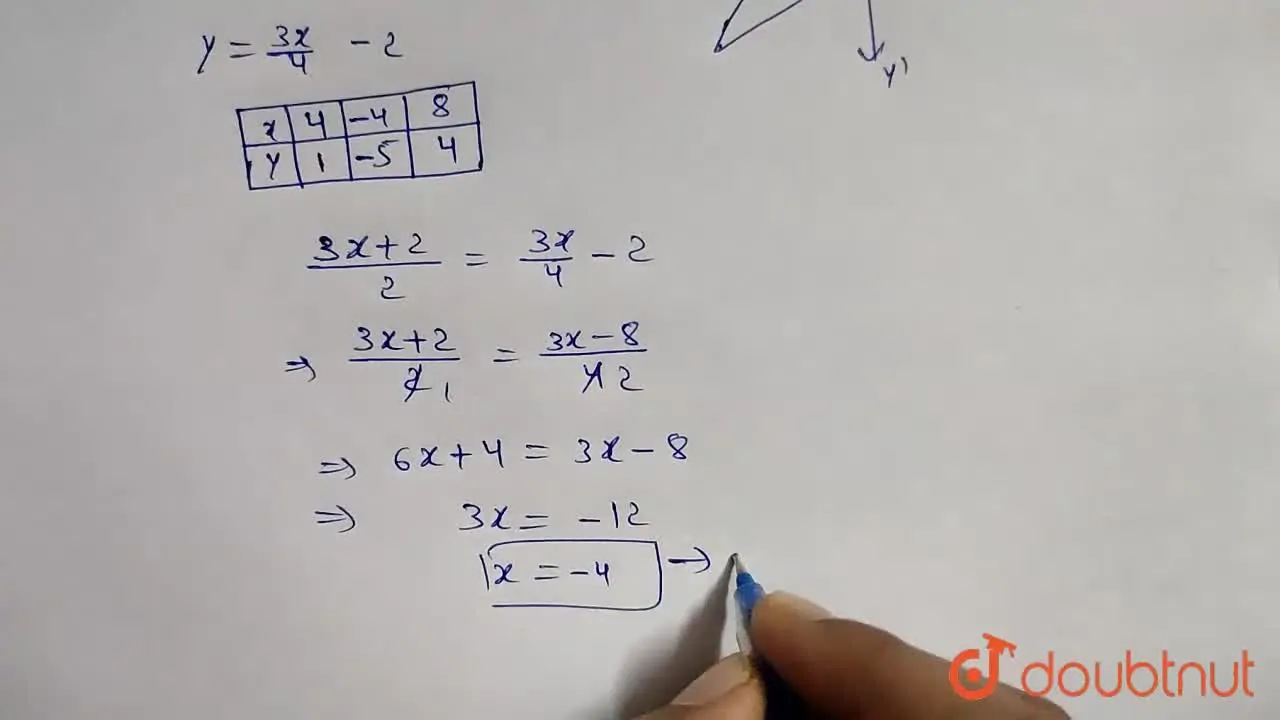
Use the graphical method to find the value of x for which the expressions 3x+22and3x4−2 are equal.

Video Solution
Doubtnut Promotions Banner
Text Solution
Generated By DoubtnutGPT

The correct Answer is:1

To solve the problem using the graphical method, we need to find the value of x for which the expressions 3x+22 and 3x4−2 are equal. Here’s a step-by-step solution:

Step 1: Define the equations
We start by defining the two expressions as equations:
1. y1=3x+22
2. y2=3x4−2

Step 2: Create a table of values for y1
We will calculate several values of y1 for different values of x:

- For x=−2:
y1=3(−2)+22=−6+22=−42=−2

- For x=2:
y1=3(2)+22=6+22=82=4

- For x=4:
y1=3(4)+22=12+22=142=7

Step 3: Create a table of values for y2
Now, we will calculate several values of y2 for the same values of x:

- For x=−4:
y2=3(−4)4−2=−3−2=−5

- For x=4:
y2=3(4)4−2=3−2=1

- For x=8:
y2=3(8)4−2=6−2=4

Step 4: Plot the points on a graph
Next, we plot the points for both equations on a graph:
- For y1:
- Point 1: (−2,−2)
- Point 2: (2,4)
- Point 3: (4,7)

- For y2:
- Point 1: (−4,−5)
- Point 2: (4,1)
- Point 3: (8,4)

Step 5: Draw the lines
Draw straight lines through the points for both y1 and y2. The intersection point of these two lines will give us the value of x we are looking for.

Step 6: Find the intersection point
From the graph, we can observe where the two lines intersect. This intersection point corresponds to the value of x where y1=y2.

Step 7: Solve algebraically (if needed)
To confirm the intersection point, we can set the two equations equal to each other and solve for x:
3x+22=3x4−2
Multiplying through by 4 to eliminate the denominators:
4⋅3x+22=3x−8
This simplifies to:
6x+4=3x−8
Rearranging gives:
6x−3x=−8−4
3x=−12
x=−4

Final Answer
Thus, the value of x for which the two expressions are equal is x=−4.

---

Updated on:7/8/2024
|