Evaluate :
The correct Answer is:
To evaluate the expression
(xqxr)1qr⋅(xrxp)1rp⋅(xpxq)1pq,
we will follow these steps:
Step 1: Apply the Quotient Rule of Exponents
Using the property of exponents that states xmxn=xm−n, we can simplify each fraction inside the parentheses.
xqxr=xq−r,xrxp=xr−p,xpxq=xp−q.
Step 2: Rewrite the Expression
Now we can rewrite the original expression using the results from Step 1:
(xq−r)1qr⋅(xr−p)1rp⋅(xp−q)1pq.
Step 3: Apply the Power Rule of Exponents
Using the property of exponents that states (xm)n=xm⋅n, we can simplify further:
x(q−r)⋅1qr⋅x(r−p)⋅1rp⋅x(p−q)⋅1pq.
Step 4: Combine the Exponents
Now, we can combine the exponents since they have the same base x:
xq−rqr⋅xr−prp⋅xp−qpq=x(q−rqr+r−prp+p−qpq).
Step 5: Simplify the Exponent
Now we need to simplify the exponent:
q−rqr+r−prp+p−qpq.
Finding a common denominator, which is pqr:
=(q−r)p+(r−p)q+(p−q)rpqr.
Step 6: Expand and Combine Terms
Expanding the numerator:
=qp−rp+rq−pq+pr−qrpqr=(qp−pq)+(rq−qr)+(pr−rp)pqr=0pqr=0.
Step 7: Final Result
Thus, we have:
x0=1.
Final Answer:
The evaluated expression is
1.
---
Using the property of exponents that states xmxn=xm−n, we can simplify each fraction inside the parentheses.
Now we can rewrite the original expression using the results from Step 1:
Using the property of exponents that states (xm)n=xm⋅n, we can simplify further:
Now, we can combine the exponents since they have the same base x:
Now we need to simplify the exponent:
Expanding the numerator:
Thus, we have:
The evaluated expression is
Topper's Solved these Questions
- INDICES (EXPONENTS)Book:ICSEChapter:INDICES (EXPONENTS)Exercise:4 Marks Questions
Explore 9 Videos
- ICSE EXAMINATION PAPER 2020 Book:ICSEChapter:ICSE EXAMINATION PAPER 2020 Exercise:SECTION - B
Explore 21 Videos
- INDICES [EXPONENTS]Book:ICSEChapter:INDICES [EXPONENTS]Exercise:EXERCISE 7 (C)
Explore 19 Videos
Similar Questions
Solve : 1p+1q+1x=1x+p+q
View SolutionIf Pr is the coefficient of x® in the expansion of
(1+x)2(1+x2)2(1+x22)2(1+x23)2... prove that
Pr=22(2r−1)(Pr−1+Pr−2)andP4=1072315 .
View Solution∫pxp+2q−1−qxq−1x2p+2q+2xp+q+1dx is equal to
(1) −xpxp+q+1+C
(2) xqxp+q+1+C
(3) −xqxp+q+1+C
(4) xpxp+q+1+C
View Solution∫x132(1+x52)12dx=P(1+x52)72+Q(1+x52)52+R(1+x52)32+C then P, Q ,R are
View SolutionConsider
∫x3+3x2+2x+1√x2+x+1dx
=(ax2+bx+c)√x2+x+1+λ∫dx√x2+x+1
Now, match the following lists and then choose the correct code.
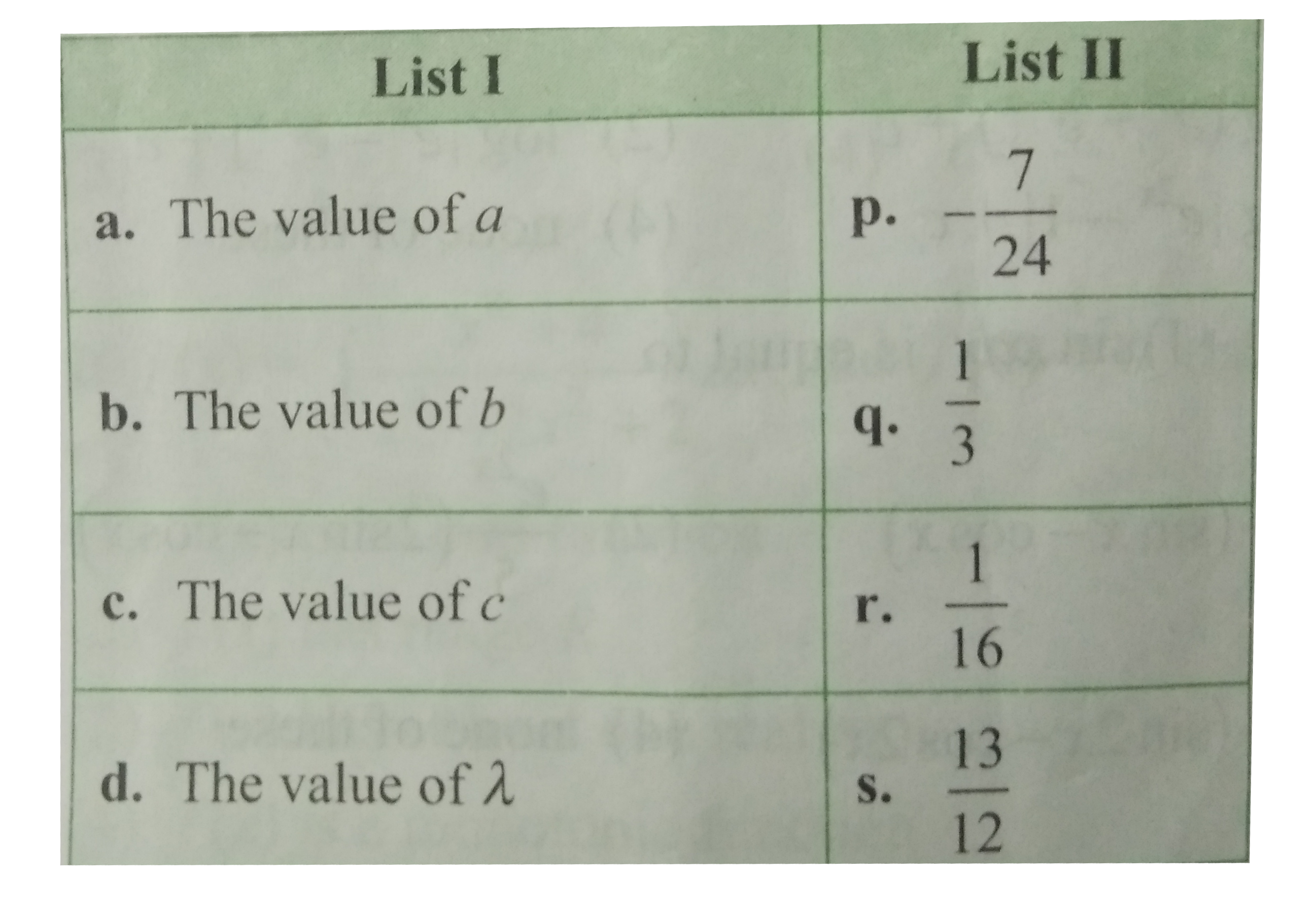
Codes:
abcd(1)qpsr(2)spqr(3)rqps(4)qspr
View Solution(3p2qr3)×(−4p3q2r2)×(7pq2r) is equal to
View SolutionThe roots of the equation (q−r)x2+(r−p)x+p−q=0 are (A) r−pq−r,1 (B) p−qq−r,1 (C) q−rp−q,1 (D) r−pp−q,1
View SolutionIf p>q>0 and pr<−1<qr, then find the value of tan−1(p−q1+pq)+tan−1(q−r1+qr)+tan−1(r−p1+rp)
View SolutionThe lines 1)(p−q)x+(q−r)y+(r−p)=0,2)(q−r)x+(r−p)y+(p−q)=0,3)(r−p)x+(p−q)y+(q−r)=0
View SolutionIf logxq−r=logyr−p=logzp−q prove that xq+r.yr+p.zp+q=xp.yq.zr
View Solution