When two thin lenses of focal lengths fi and f, are kept coaxially and in contact, prove that their cobined focal length "f" is given by :
1f=1f1+1f2
Suppose two thin convex lenses l1andL2 of focal lengths f1andf2 are placed in contact in air having a common principal axis. A point object O is placed on the principal axis at a distance u from the first lens L1. Its real image would be formed by the lens l1 alone at I., distinct v. (say) from L1. Then, from the lens formula, we have
1v.−1u=1f1

I. serves as a virtual object for the second lens l2 which forms a final image I at a distance v (say) from it. Then, we have
1v−1v.=1f2 ..(ii)
on adding equations (i) and (ii), we get: 1v−1u=1f1+1f2 ..(iii)
If we replaces these two lenses by a single lens which forms the image of an object placed at distance u from it, at a distance v, then the focal length F of this equivalent lens would be given by
1v−1u=1F ..(iv)
From equations (iii) and (iv) we get
1F=1f1+1f2
Similar Questions
Knowledge Check
A plano convex lens has focal length f=20cm. If its plane surface is silvered, then new focal length will be
A20cmB40 cmC30 cmD10 cm
Two thin lenses of focal length f1 and f2 are in contact and coaxial. The power of the combination is
View SolutionTwo thin lenses, one of focal length + 60 cm and the other of focal length – 20 cm are put in contact. The combined focal length is
View SolutionTwo similar thin equi-convex lenses, of focal f each, are kept coaxially in contact with each other such that the focal length of the combination is F1, When the space between the two lens is filled with glycerin (which has the same refractive index (μ=1.5) as that of glass) then the equivlent focal length is F2, The ratio F1:F2 will be
View SolutionA convex lens of focal length f cut into parts first horizontally and then vertically. Find the focal length of part A of the lens, as shown:
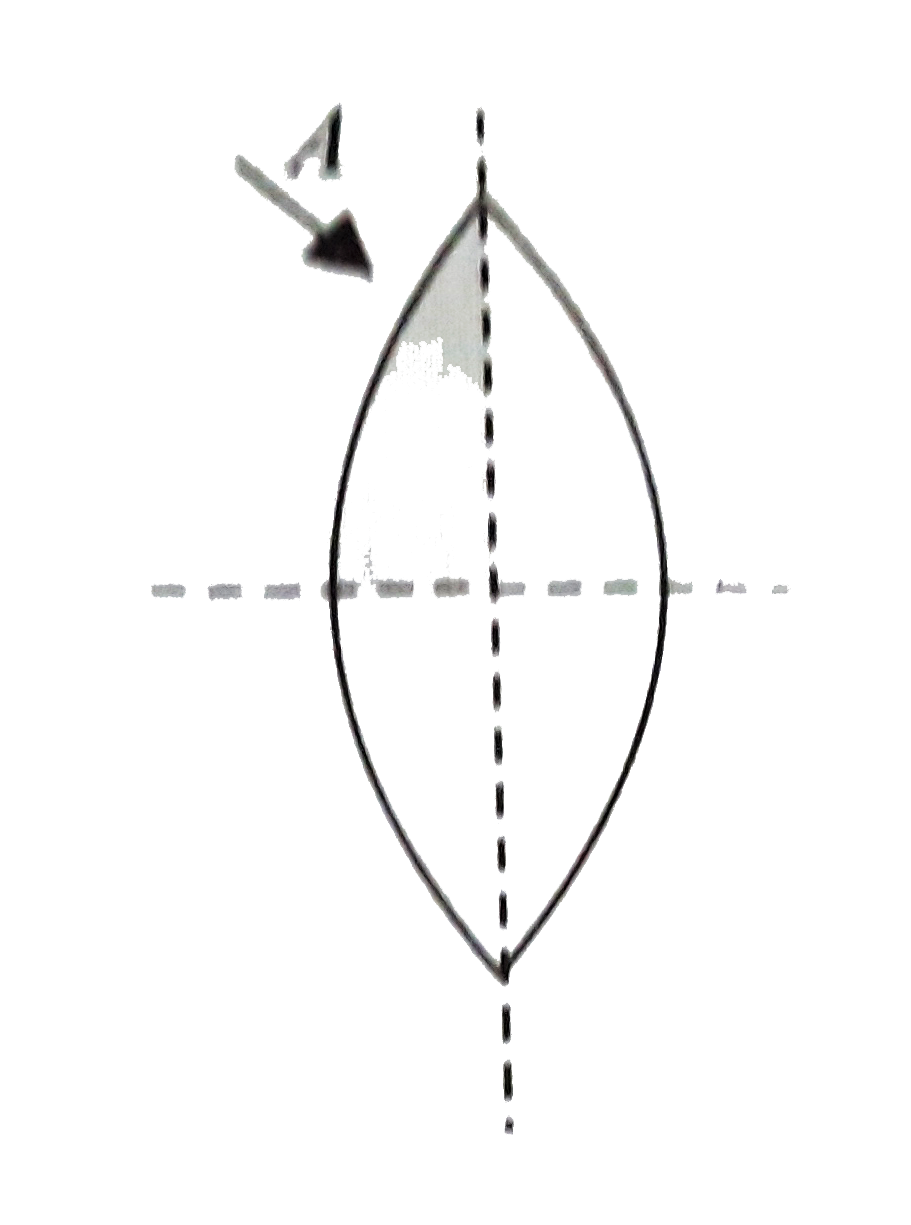
View SolutionA convex lens of focal length f1 is kept in contact with a concave lens of focal length f2. Find the focal length of the combination.
View Solution(a) Using the ray diagram for a system of two lenses of focal lengths f1andf2 in contact with each other, show that the two lens system can be regarded as equivalent to a single lens of focal length f, where
1f=1f1+1f2
Also write the relation for the equivalent power of the lens combination.
(b) Determine the position of the image formed by the lens combination given in the figure.
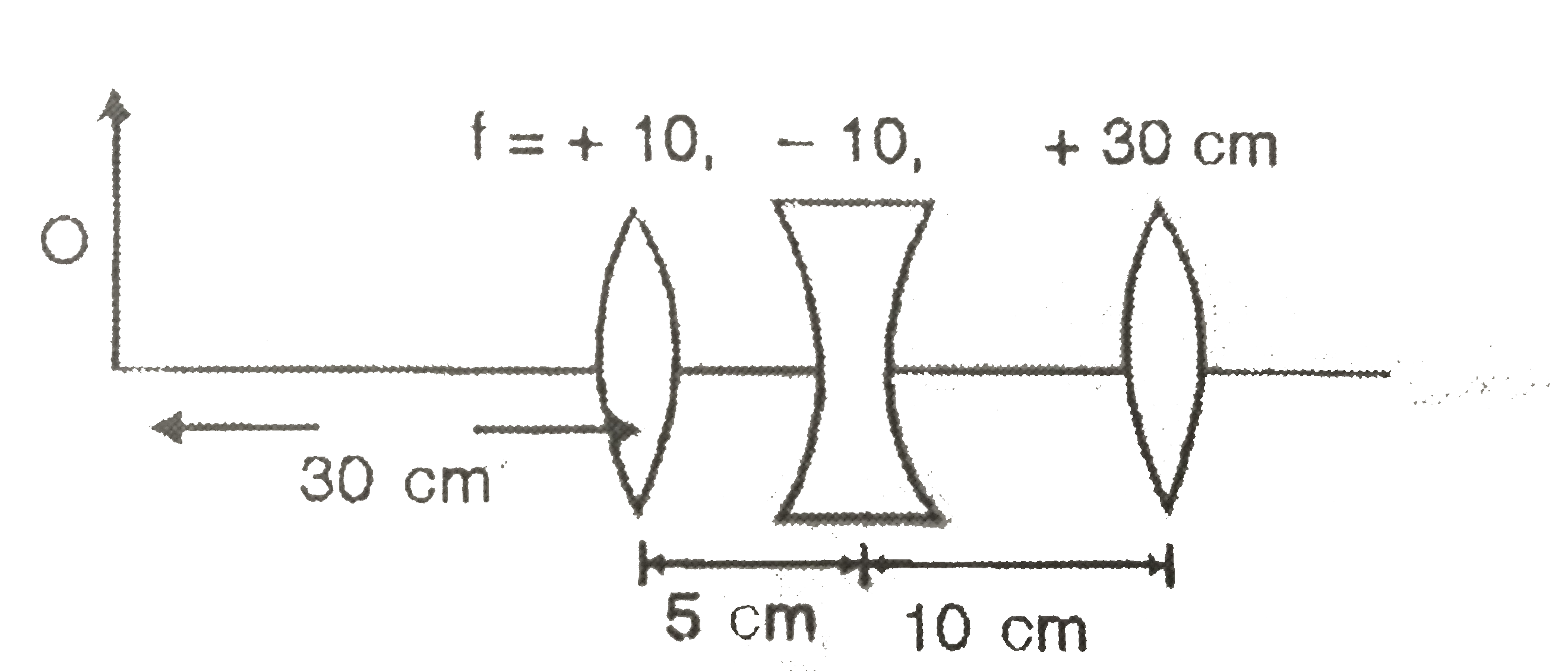
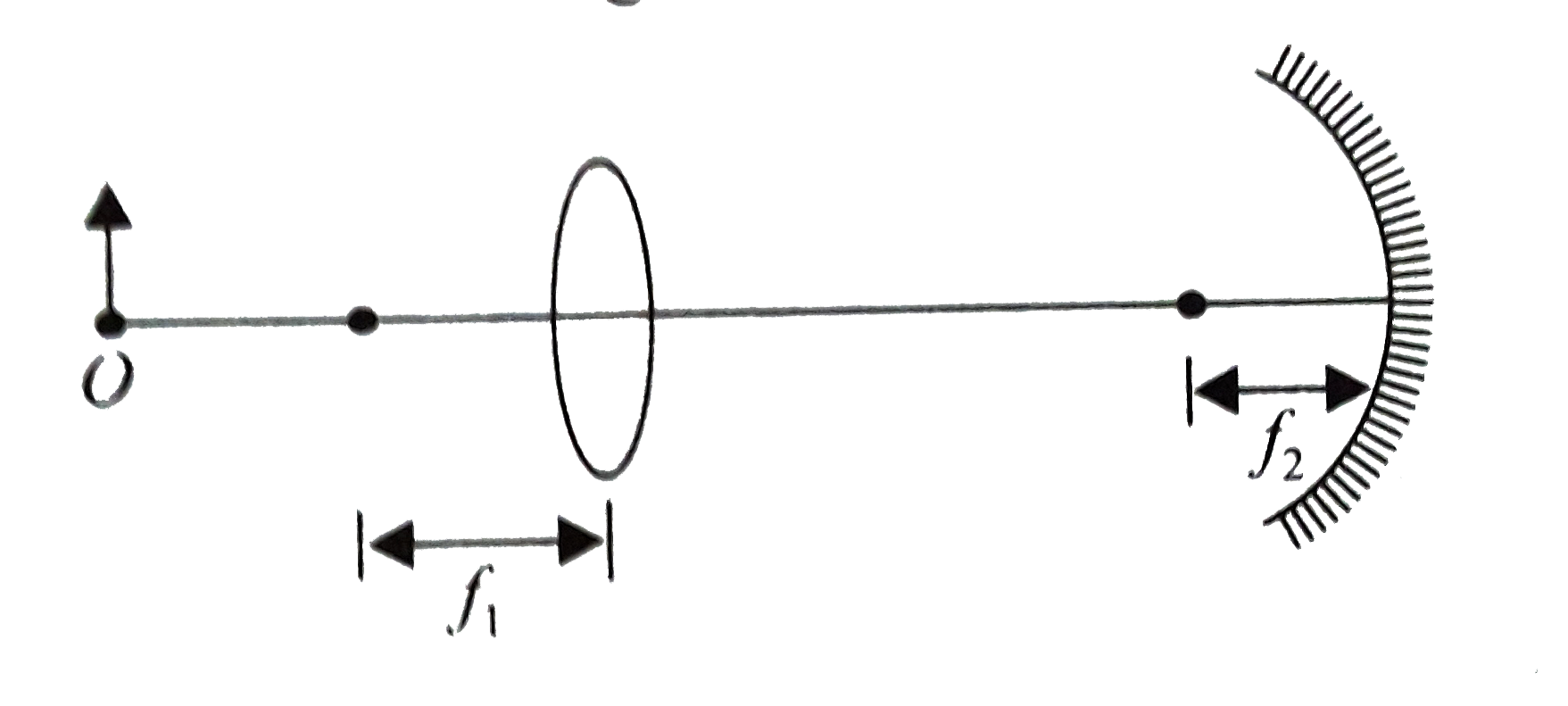
View SolutionAn object is placed in front of a converging lens at a distance equal to twice the focal length f1 of the lens. On the other side of the lens is a concave mirror of focal length f2 separated from the lens is a concave mirror of focal length f2 separated from the lens by a distance 2(f1+f2). Light from the object passes rightward through the lens, reflects from the mirror, passes leftward throught the lens, and forms a final image of the object.
View Solution