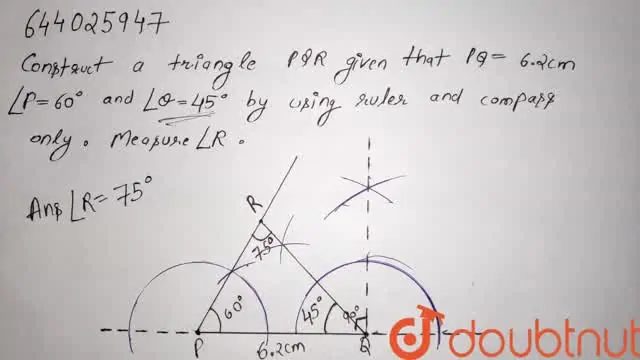
Construct a triangle PQR given that PQ = 6 . 2 cm ∠P=60∘and∠Q=45∘ by using ruler and compass only. Measure ∠R
The correct Answer is:75∘
Step by step video, text & image solution for Construct a triangle PQR given that PQ = 6 . 2 cm angle P = 60^(@) and angle Q = 45^(@) by using ruler and compass only. Measure angle R by Maths experts to help you in doubts & scoring excellent marks in Class 6 exams.
Similar Questions
Construct a triangle ABC such that AB = 6 . 5 cm , ∠A=60∘and∠B=45∘ by using ruler and compass .
View SolutionConstruct a triangle ABC given that
AB = 6 cm, ∠A=55∘and∠B=65∘View SolutionConstruct a triangle PQR with PQ = 4 cm, ∠P=75∘,and∠R=65∘.
View SolutionConstruct a triangle ABC given that :
AB = 5 cm, AC = 6 cm, ∠A=68∘View SolutionConstruct a triangle PQR with PQ = 9 cm, ∠P=55∘,∠R=35∘.
View SolutionConstruct a triangle ABC in which BC=5cm,∠B=60∘and∠C=45∘.
View SolutionFind PQ, if AB=150 m, ∠P=30∘and∠Q=45∘ (ii)
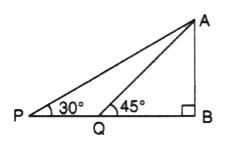 View Solution
View SolutionFind PQ, if AB=150 m, ∠P=30∘and∠Q=45∘ (i)
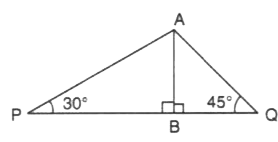 View Solution
View SolutionConstruct a triangle PQR in which QR = 6cm, ∠Q=60∘ and PR – PQ = 2cm.
View SolutionBy using ruler and compass, construct angles of :
105∘.View Solution