The coefficient of (x3⋅b6⋅C8⋅d9⋅e⋅f) in the expansion
of (a+b+c−d−e−f)31 is
12632
23110
3110
None of these
The correct Answer is:d
To find the coefficient of x3b6c8d9e1f1 in the expansion of (a+b+c−d−e−f)31, we will use the multinomial theorem.
1. Identify the total exponent and the terms:
We have the expression (a+b+c−d−e−f)31. The total exponent is 31.
2. Set up the multinomial coefficient:
The general term in the expansion can be represented as:
31!p1!p2!p3!p4!p5!p6!⋅ap1bp2cp3(−d)p4(−e)p5(−f)p6
where p1+p2+p3+p4+p5+p6=31.
3. Assign the powers to each variable:
From the term x3b6c8d9e1f1, we assign:
- p1=3 (for x)
- p2=6 (for b)
- p3=8 (for c)
- p4=9 (for d)
- p5=1 (for e)
- p6=1 (for f)
4. Check the sum of powers:
We need to ensure that the sum of the powers equals 31:
3+6+8+9+1+1=28
Since 28≠31, we need to adjust our powers.
5. Calculate the remaining power:
The remaining power is 31−28=3. This means we need to distribute 3 among the variables a (which corresponds to p1).
6. Calculate the multinomial coefficient:
The multinomial coefficient will be:
31!3!6!8!9!1!1!
7. Account for the negative signs:
The terms −d,−e,−f contribute a factor of (−1)p4+p5+p6=(−1)9+1+1=(−1)11=−1.
8. Final coefficient:
Therefore, the coefficient of x3b6c8d9e1f1 is:
−31!3!6!8!9!1!1!
Conclusion:
Since the coefficient is negative and the options do not include negative values, the answer is "none of these".
We have the expression (a+b+c−d−e−f)31. The total exponent is 31.
The general term in the expansion can be represented as:
31!p1!p2!p3!p4!p5!p6!⋅ap1bp2cp3(−d)p4(−e)p5(−f)p6
where p1+p2+p3+p4+p5+p6=31.
From the term x3b6c8d9e1f1, we assign:
- p1=3 (for x)
- p2=6 (for b)
- p3=8 (for c)
- p4=9 (for d)
- p5=1 (for e)
- p6=1 (for f)
We need to ensure that the sum of the powers equals 31:
3+6+8+9+1+1=28
Since 28≠31, we need to adjust our powers.
The remaining power is 31−28=3. This means we need to distribute 3 among the variables a (which corresponds to p1).
The multinomial coefficient will be:
31!3!6!8!9!1!1!
The terms −d,−e,−f contribute a factor of (−1)p4+p5+p6=(−1)9+1+1=(−1)11=−1.
Therefore, the coefficient of x3b6c8d9e1f1 is:
−31!3!6!8!9!1!1!
Since the coefficient is negative and the options do not include negative values, the answer is "none of these".
Topper's Solved these Questions
- BIONOMIAL THEOREMBOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise (More Than One Correct Option Type Questions)
15 Videos
- BIONOMIAL THEOREMBOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise (Passage Based Questions)
21 Videos
- BIONOMIAL THEOREMBOOK - ARIHANT MATHS ENGLISHCHAPTER - BIONOMIAL THEOREMEXERCISE - Exercise For Session 4
14 Videos
- AREA OF BOUNDED REGIONSBOOK - ARIHANT MATHS ENGLISHCHAPTER - AREA OF BOUNDED REGIONSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
23 Videos
- CIRCLE BOOK - ARIHANT MATHS ENGLISHCHAPTER - CIRCLE EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
16 Videos
Similar Questions
In △ABC, D, E and F are, respectively, the mid-points of BC, CA and AB . If the lengths of side AB, BC and CA are 7cm, 8cm, and 9cm, respectively, find the perimeter of △DEF.
View SolutionIf a,b,c,d,e, f are A. M's between 2 and 12 , then find a+b+c+d+e+f.
View SolutionLet A =(a,b,c),B=(b,d,e)andC=(e,f,g) , verify that :
A∩B=B∩A
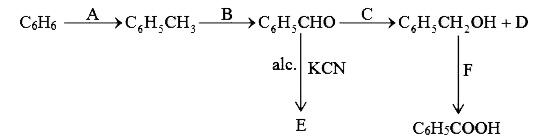
View SolutionIdentify the compounds A, B, C, D, E and F.
View SolutionLet A =(a,b,c),B=(b,d,e)andC=(e,f,g) , verify that :
A∪B=B∪A
View SolutionIf in two triangles ABC and DEF , ABDE=BCFE=CAFD , then FDECAB (b) FDEABC (c) CBAFDE (d) BCAFDE
View SolutionD, E, F are the mid-point of the sides BC, CA and AB respectively of △ABC. Then DEF is congruent to triangle.
(a)ABC
(b) AEF
(c) BFD, CDE
(d) AFE, BFD, CDE
View SolutionABCD is a parallelogram and E and F are the centroids of triangles ABD and BCD respectively, then EF=
(a)AE
(b) BE
(c) CE
(d) DE
View SolutionIn Figure, CD and GH are respectively the medians of ABC and EFG . If ABC~FEG , prove that (i)ADC~FHG (ii)CDGH=ABFE (iii)CDB~GHE
View SolutionO is any point inside a triangle ABC . The bisector of ∠AOB,∠BOC and ∠COA meet the sides AB,BC and CA in point D,EandF respectively. Show that AD⋅BE⋅CF=DB⋅EC⋅FA
View Solution
