Write down 2×2 matrix A which corresponds to a counterclockwise rotation of 60∘ about tha origin. In the diagram OB of 2√2 units in lenth. The square is rotated counterclockwise about O through 60∘ find the coordiates of the vertices of the square after rotating.
To solve the problem of finding the coordinates of the vertices of a square after a counterclockwise rotation of 60∘ about the origin, we will follow these steps:
Step 1: Define the rotation matrix
The rotation matrix A for a counterclockwise rotation by an angle θ is given by:
A=(cosθ−sinθsinθcosθ)
For θ=60∘:
cos60∘=12,sin60∘=√32
Thus, the rotation matrix A becomes:
A=⎛⎜⎝12−√32√3212⎞⎟⎠
Step 2: Identify the coordinates of the square vertices
Given that the length of each side of the square is 2 units, the coordinates of the vertices of the square before rotation are:
- A(2,0)
- B(2,2)
- C(0,2)
- O(0,0) (the origin)
Step 3: Rotate each vertex using the rotation matrix
We will apply the rotation matrix A to each vertex.
For vertex A (2, 0):
(x′y′)=A⋅(20)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(20)=⎛⎜⎝12⋅2+(−√32)⋅0√32⋅2+12⋅0⎞⎟⎠=(1√3)
Thus, A′=(1,√3).
For vertex B (2, 2):
(x′y′)=A⋅(22)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(22)=⎛⎜⎝12⋅2+(−√32)⋅2√32⋅2+12⋅2⎞⎟⎠=(1−√3√3+1)
Thus, B′=(1−√3,1+√3).
For vertex C (0, 2):
(x′y′)=A⋅(02)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(02)=(−√31)
Thus, C′=(−√3,1).
Step 4: Summarize the new coordinates
After the rotation, the new coordinates of the vertices of the square are:
- A′=(1,√3)
- B′=(1−√3,1+√3)
- C′=(−√3,1)
- O=(0,0)
The rotation matrix A for a counterclockwise rotation by an angle θ is given by:
A=(cosθ−sinθsinθcosθ)
For θ=60∘:
cos60∘=12,sin60∘=√32
Thus, the rotation matrix A becomes:
A=⎛⎜⎝12−√32√3212⎞⎟⎠
Given that the length of each side of the square is 2 units, the coordinates of the vertices of the square before rotation are:
- A(2,0)
- B(2,2)
- C(0,2)
- O(0,0) (the origin)
We will apply the rotation matrix A to each vertex.
(x′y′)=A⋅(20)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(20)=⎛⎜⎝12⋅2+(−√32)⋅0√32⋅2+12⋅0⎞⎟⎠=(1√3)
Thus, A′=(1,√3).
(x′y′)=A⋅(22)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(22)=⎛⎜⎝12⋅2+(−√32)⋅2√32⋅2+12⋅2⎞⎟⎠=(1−√3√3+1)
Thus, B′=(1−√3,1+√3).
(x′y′)=A⋅(02)=⎛⎜⎝12−√32√3212⎞⎟⎠⋅(02)=(−√31)
Thus, C′=(−√3,1).
After the rotation, the new coordinates of the vertices of the square are:
- A′=(1,√3)
- B′=(1−√3,1+√3)
- C′=(−√3,1)
- O=(0,0)
Topper's Solved these Questions
- MATRICES BOOK - ARIHANT MATHS ENGLISHCHAPTER - MATRICES EXERCISE - Exercise For Session 1
9 Videos
- MATRICES BOOK - ARIHANT MATHS ENGLISHCHAPTER - MATRICES EXERCISE - Exercise For Session 2
19 Videos
- MATHEMATICAL INDUCTION BOOK - ARIHANT MATHS ENGLISHCHAPTER - MATHEMATICAL INDUCTION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHS ENGLISHCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
29 Videos
Similar Questions
Knowledge Check
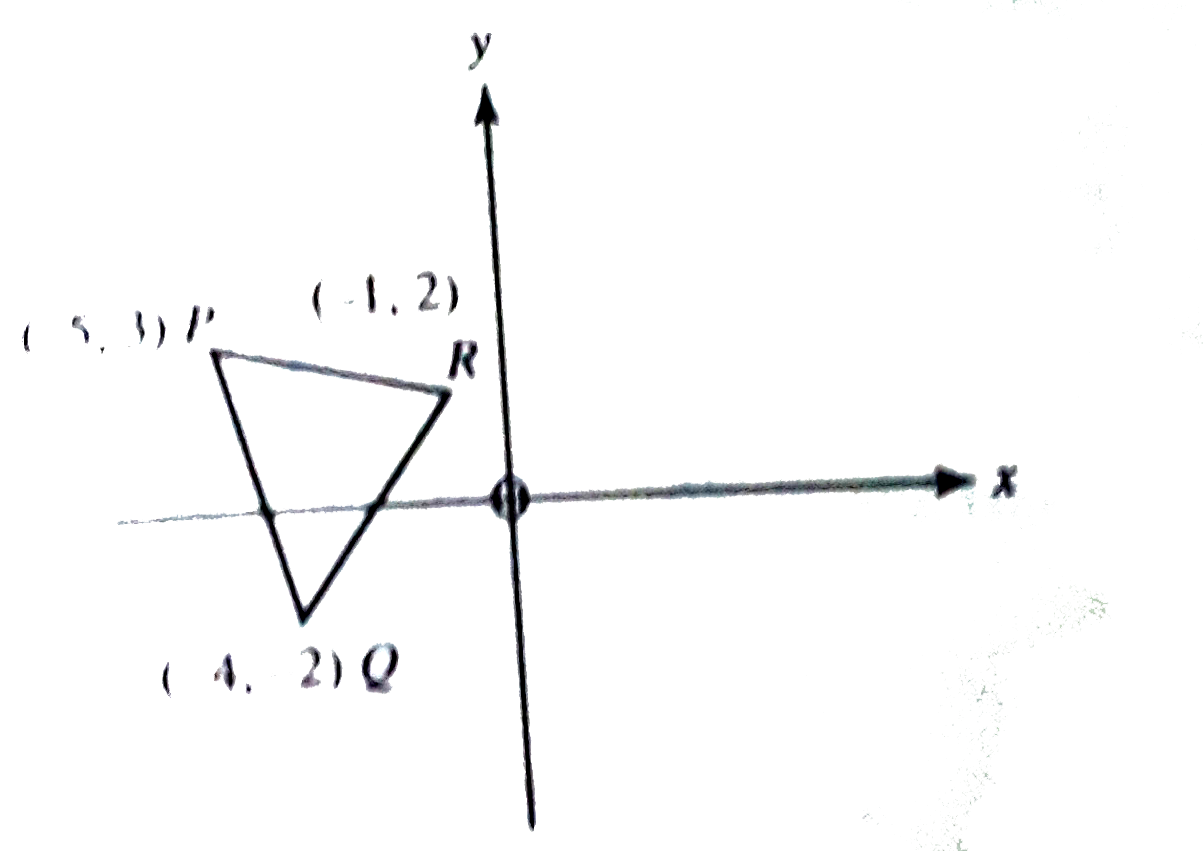
Triangle PQR , shown in the diagram above , is translated 4 units to the right and 5 units down. The resulting triangle is then rotated 180∘ counterclockwise about the origin. What is the final image of point P ?A(-1,-2)B(1,2)C(2,1)D(-2,1)If point P(3, 2) is rotated 90 degree counterclockwise with respect to the origin, what will be its new coordinates ?
A(−2,3)B(−2,−3)C(−3,3)D(−3,2)
If the pair of straight lines ax2+2hxy+by2=0 is rotated about the origin through 900 , then find the equations in the new position.
View SolutionA line through the point P(1,2) makes an angle of 600 with the positive direction of x-axis and is rotated about P in the clockwise direction through an angle 150. Find the equation of the straight line in the new position.
View Solutionif the axes are rotated through 60 in the anticlockwise sense,find the transformed form of the equation x2−y2=a2,
View SolutionIf the transferred equation of a curve is x2+2√3xy−y2=2a2 when the axes are rotated through an angle 60∘, then the original equation of the curve is
View SolutionA line through the point A(2,0) which makes an angle of 300 with the positive direction of x-axis is rotated about A in clockwise direction through an angle 150. Find the equation of the straight line in the new position.
View SolutionLet A(1,2) and B(5,6). If the axes are rotated about origin such that the new x-axis is parallel to the line though A and B. The find the co-ordinates of the mid point of segment ¯¯¯¯¯¯AB with respect to new system.
View Solution
