In the ΔABC,AB=a,AC=candBC=b, then
a+b+c=0
a+b-c=0
a-b+c=0
−a+b+c=0
The correct Answer is:B
To solve the problem, we need to analyze the vectors in triangle ABC with the given sides: AB = a, AC = c, and BC = b. We will express the sides as vectors and use the properties of a triangle to find the correct equation among the provided options.
1. Identify the Vectors:
- Let →A represent the vector from point A to point B (i.e., →AB=→A).
- Let →B represent the vector from point B to point C (i.e., →BC=→B).
- Let →C represent the vector from point C to point A (i.e., →CA=→C).
2. Set Up the Equation:
- In a triangle, the sum of the vectors around the triangle must equal zero. Thus, we have:
→AB+→BC+→CA=0
- Substituting the vectors we defined:
→A+→B+→C=0
3. Express CA in Terms of Vectors:
- Since →CA=−→C, we can rewrite the equation:
→A+→B−→C=0
4. Rearranging the Equation:
- Rearranging gives us:
→A+→B−→C=0
- This implies:
→A+→B=→C
5. Identify the Correct Option:
- From the equation →A+→B−→C=0, we see that the correct option among the given choices is:
→A+→B−→C=0
- Therefore, the answer is option 2: →A+→B−→C=0.
- Let →A represent the vector from point A to point B (i.e., →AB=→A).
- Let →B represent the vector from point B to point C (i.e., →BC=→B).
- Let →C represent the vector from point C to point A (i.e., →CA=→C).
- In a triangle, the sum of the vectors around the triangle must equal zero. Thus, we have:
→AB+→BC+→CA=0
- Substituting the vectors we defined:
→A+→B+→C=0
- Since →CA=−→C, we can rewrite the equation:
→A+→B−→C=0
- Rearranging gives us:
→A+→B−→C=0
- This implies:
→A+→B=→C
- From the equation →A+→B−→C=0, we see that the correct option among the given choices is:
→A+→B−→C=0
- Therefore, the answer is option 2: →A+→B−→C=0.
Topper's Solved these Questions
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:Exercise (More Than One Correct Option Type Questions)
Explore 7 Videos
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:Exercise (Statement I And Ii Type Questions)
Explore 3 Videos
- VECTOR ALGEBRABook:ARIHANT MATHS ENGLISHChapter:VECTOR ALGEBRAExercise:VECTOR ALGEBRA EXERCISES 1: Single Option Correct Type Questions
Explore 1 Video
- TRIGONOMETRIC FUNCTIONS AND IDENTITIESBook:ARIHANT MATHS ENGLISHChapter:TRIGONOMETRIC FUNCTIONS AND IDENTITIESExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 19 Videos
Similar Questions
In a ΔABC,B=90∘,AC=h and the length of perpendicular from B to AC is p such that h=4p. If AB<BC, then measure ∠C.
View SolutionIn ΔABC,AB=AC=x,BC=10cm and the area of triangle is 60 cm2. Find x.
View SolutionIn ΔABC,AB=AC and the bisectors of angles B and C intersect at point O. Prove that
BO=OC
View SolutionIn ΔABC,AB=AC and D is a point in BC so that BD=CD. Prove that AD bisects ∠BAC.
View SolutionIn a ΔABC, if(c+a+b) (a+b-c) =ab, then the measure of angle C is
View SolutionIn ΔABC, if BC>AC>AB then :
(i) What is the relation ∠A and ∠C ?
(ii) What is the relation between the values of (AB+AC) and BC ?
(iii) What is the relation between the values of (AC−BC) and AB ?
(iv) What is the smallest angle in ΔABC ?
View SolutionA(3,4),B(0,0)andc(3,0) are vertices of ΔABC. If 'P' is the point inside the ΔABC, such that d(P,BC)≤ min. {d(P,AB),d(P,AC)}. Then the maximum of d(P,BC) is.(where d(P,BC) represent distance between PandBC).
View SolutionIn the adjoining figure, ΔABC is right angled at C and M is the mid-point of hypotenuse AB, If AC = 32 cm and BC = 60 cm, then find the length of CM.

View SolutionWhich of the following pairs of triangles are congruent ? Also state the condition of congruency in each case :
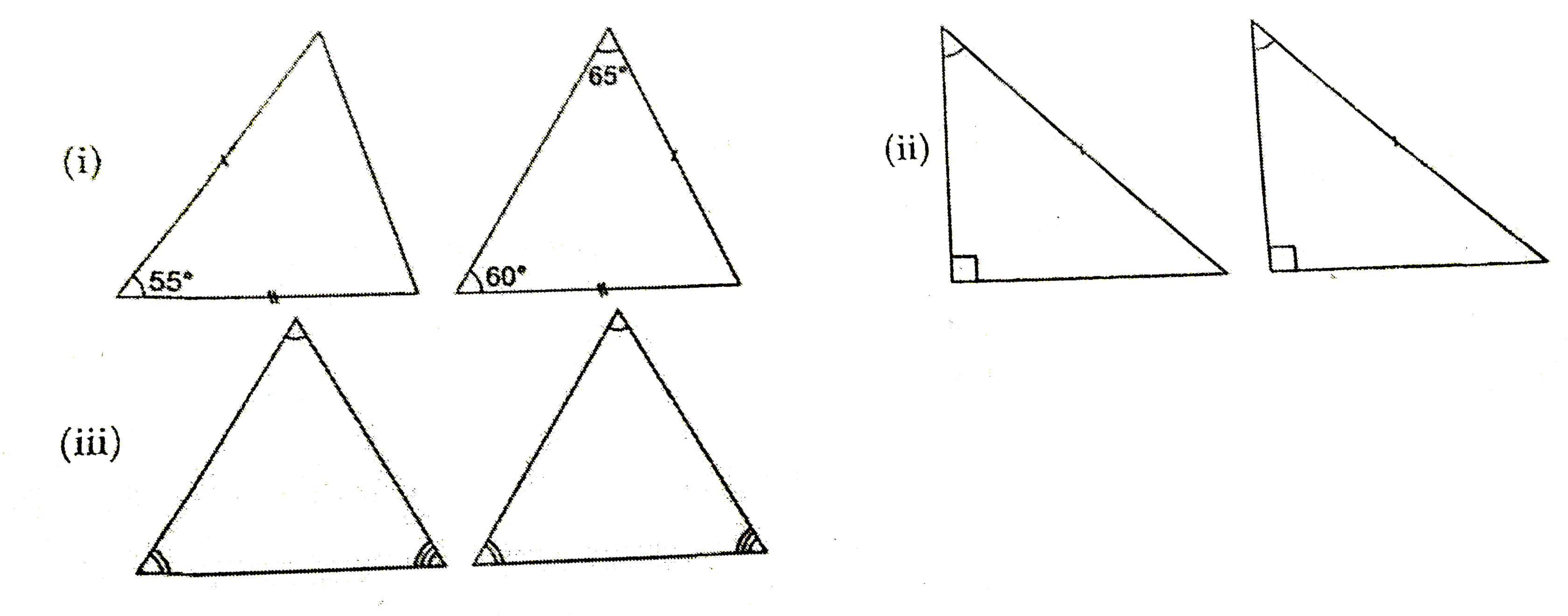
(iv) In ΔABC and ΔDEF,AB=EF,BC=DF and ∠B=∠F
(v) In ΔABC and ΔPQR,AB=QR,AC=PR and ∠B=∠R
(vi) In ΔABC and ΔPQR,∠A=∠P,AC=PR and AB=PQ
(vi) In ΔABC and ΔPQR,AB=QR,∠A=∠Q and AC=QP.
View SolutionΔABC is isosceles with AB=AC=7.5cmandBC=9cm. The height AD from A to BC is 6cm. Find the area of ΔABC. What will be the height from C to AB,CE
View Solution