The mass of a lift is 2000 kg. When the tension in the supporting cable is 28000 N, then its acceleration is
14ms−2 upwards
30ms−2 downwards
4ms−2 upwards
4ms−2 downwards
The correct Answer is:C
∑F=ma
⇒T−mg=ma
a=T−mgm=4m/s2
Similar Questions
The mass of a lift is 2000kg . When the tensioon in the supporting cable is 28000N , then its acceleration is.
View SolutionWhen an empty lift is moving down with an acceleration of g4ms−2 the tension in the cable is 9000N. When the lift is moving up with an acceleration of g3ms−2 the tension in the cable is
View Solution
Knowledge Check
Two masses 8 kg and 12 kg are connected at the two ends of a light in extensible string that passes over a friction less pulley Find the acceleration of the masses and tension in the string , when the masses are released
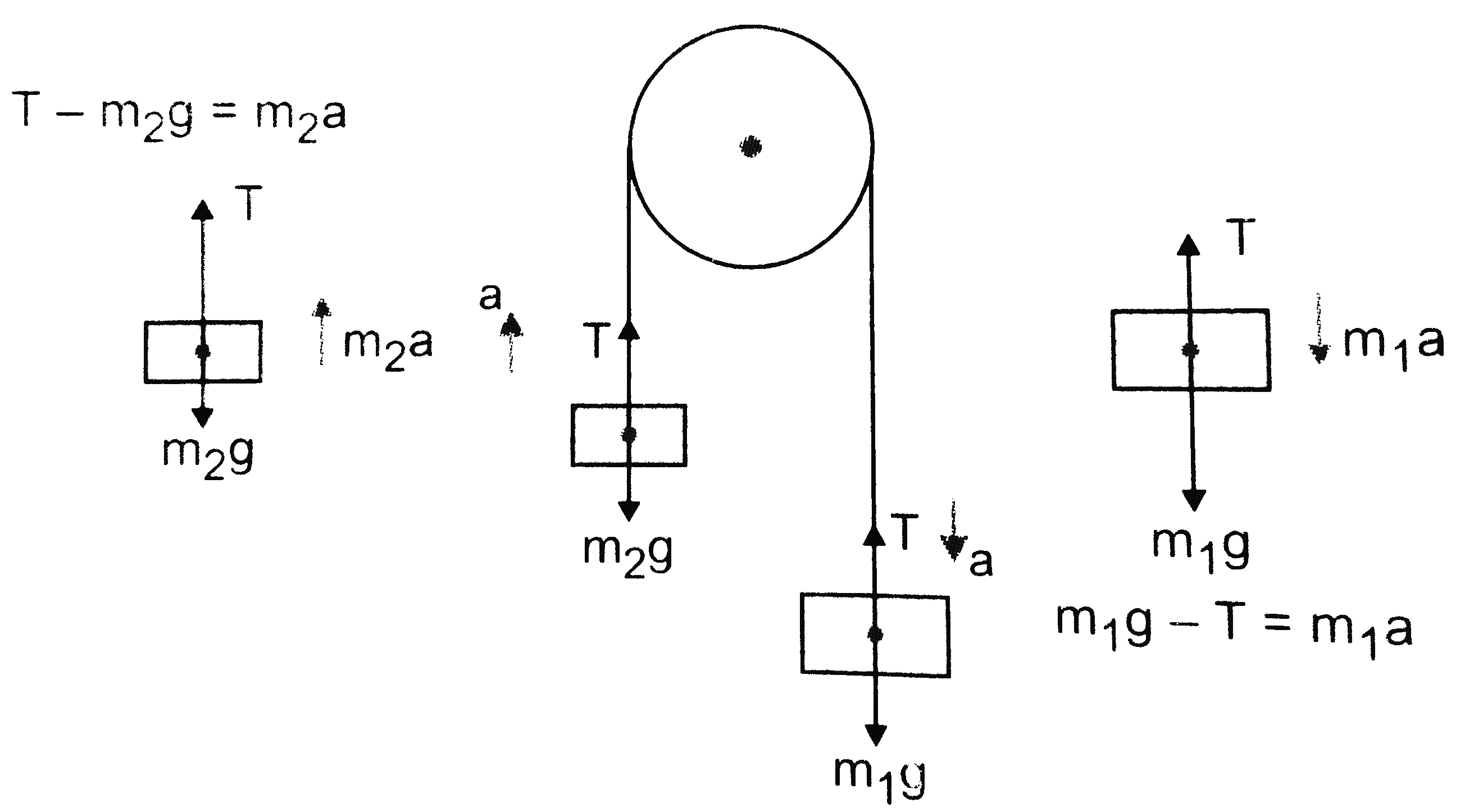. Ag4Bg5Cg8Dg6
The apparent weight of a person inside a lift is w1 when lift moves up with a certain accelration and is w2 when lift moves down with same acceleration. The weight of the person when lift moves up with constant speed is :
View SolutionA lift is going up with variable velocity as shown in figure if mass of the lift is 2000 kg Then tension in the rope of lift at t = 5 second is
View SolutionThe mass of man when standing on the lift is 60 kg. The weight when the lift is moving upwards with acceration 4.9ms−2 is
View SolutionA lift is going up. The total mass of the lift and the passenger is 1500 kg. The variation in the speed of the lift is as shown in the figure. Find the tension in the rope pulling the lift at t = 11 th second.
View SolutionThe apparent weight of a man in a lift is W1 when lift moves upwards with some acceleration and is W2 when it is accerating down with same acceleration. Find the true weight of the man and acceleration of lift .
View SolutionThe mass of a lift if 600kg and it is moving upwards with a uniform acceleration of 2m/s2. Then the tension in the cable of the lift is :
View SolutionConsider two objects of different masses connected by a cable passing over a massless and frictionless Pulley. Derive expressions for the tension in the cable and acceleration of the objects.
View Solution