A block of mass m is placed on a smooth wedge of inclination θ. The whole system is accelerated horizontally, so that the block does not slip on the wedge. The force exerted by the wedge on the block (g is acceleration due to gravity) will be
mg sin θ
mg
mg/cos θ
mg cos θ
The correct Answer is:C
To solve the problem of finding the force exerted by the wedge on the block, we can follow these steps:
Step 1: Understand the Forces Acting on the Block
The block of mass m is placed on a wedge inclined at an angle θ. The forces acting on the block are:
- The gravitational force mg acting downwards.
- The normal force N exerted by the wedge acting perpendicular to the surface of the wedge.
- A pseudo force Fp acting horizontally due to the acceleration a of the wedge.
Step 2: Resolve the Gravitational Force
The weight of the block can be resolved into two components:
- Perpendicular to the inclined surface: mgcosθ
- Parallel to the inclined surface: mgsinθ
Step 3: Resolve the Pseudo Force
The pseudo force due to the horizontal acceleration a can also be resolved:
- The component of the pseudo force acting perpendicular to the inclined surface: acosθ
- The component acting parallel to the inclined surface: asinθ
Step 4: Apply the Condition for No Slipping
Since the block does not slip on the wedge, the forces acting perpendicular to the surface must balance:
N=mgcosθ+masinθ
Step 5: Substitute the Acceleration
From the problem, we know that the wedge is accelerated such that:
a=gtanθ
This implies:
a=gsinθcosθ
Step 6: Substitute a into the Normal Force Equation
Substituting a into the normal force equation gives:
N=mgcosθ+m(gtanθ)sinθ
Substituting tanθ=sinθcosθ:
N=mgcosθ+mgsin2θcosθ
Step 7: Combine Terms
Now, factor out mg:
N=mg(cosθ+sin2θcosθ)
Combining the terms gives:
N=mg(cos2θ+sin2θcosθ)
Using the Pythagorean identity sin2θ+cos2θ=1:
N=mgcosθ
Final Result
Thus, the force exerted by the wedge on the block is:
N=mgcosθ
The block of mass m is placed on a wedge inclined at an angle θ. The forces acting on the block are:
- The gravitational force mg acting downwards.
- The normal force N exerted by the wedge acting perpendicular to the surface of the wedge.
- A pseudo force Fp acting horizontally due to the acceleration a of the wedge.
The weight of the block can be resolved into two components:
- Perpendicular to the inclined surface: mgcosθ
- Parallel to the inclined surface: mgsinθ
The pseudo force due to the horizontal acceleration a can also be resolved:
- The component of the pseudo force acting perpendicular to the inclined surface: acosθ
- The component acting parallel to the inclined surface: asinθ
Since the block does not slip on the wedge, the forces acting perpendicular to the surface must balance:
N=mgcosθ+masinθ
From the problem, we know that the wedge is accelerated such that:
a=gtanθ
This implies:
a=gsinθcosθ
Substituting a into the normal force equation gives:
N=mgcosθ+m(gtanθ)sinθ
Substituting tanθ=sinθcosθ:
N=mgcosθ+mgsin2θcosθ
Now, factor out mg:
N=mg(cosθ+sin2θcosθ)
Combining the terms gives:
N=mg(cos2θ+sin2θcosθ)
Using the Pythagorean identity sin2θ+cos2θ=1:
N=mgcosθ
Thus, the force exerted by the wedge on the block is:
N=mgcosθ
Similar Questions
A block of mass m is placed on a smooth wedge of incination θ. The whole system s acelerated horizontally so tht the block does not slip on the wedge. The force exerted by the wedge on the block has a magnitude
View SolutionA block of mass m is placed on a smooth wedge of inclination Q. The whole system is accelerated horizontally so that the block does not slip on the wedge. Find the
i) Acceleration of the wedge
ii) Force to be applied on the wedge
iii) Force exerted by the wedge on the block.
View SolutionA block of mass 'm' is kept on a smooth moving wedge. If the acceleration of the wedge is 'a'
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA block of mass m is placed on a smooth inclined plane of inclination θ with the horizontal. The force exerted by the plane on the block has a magnitude
View SolutionA small block of mass m is kept on a rough inclined surface of inclination θ fixed in a car. The car moves with a uniform velocity v and the block does not slide on the wedge. The work done by the force of friction on the block in time t will be
_PHY_E05_250_Q01.png)
View SolutionAs shown in the figure a block of mass 'm' is placed on a smooth wedge moving with constant acceleration such that block does not move with respect to the wedge. Find work done by the net force on the block in time t. (Initial speed is 0)
View SolutionA smooth block of mass m is held stationary on a smooth wedge of mass M and inclination θ as shown in figure .If the system is released from rest , then the normal reaction between the block and the wedge is

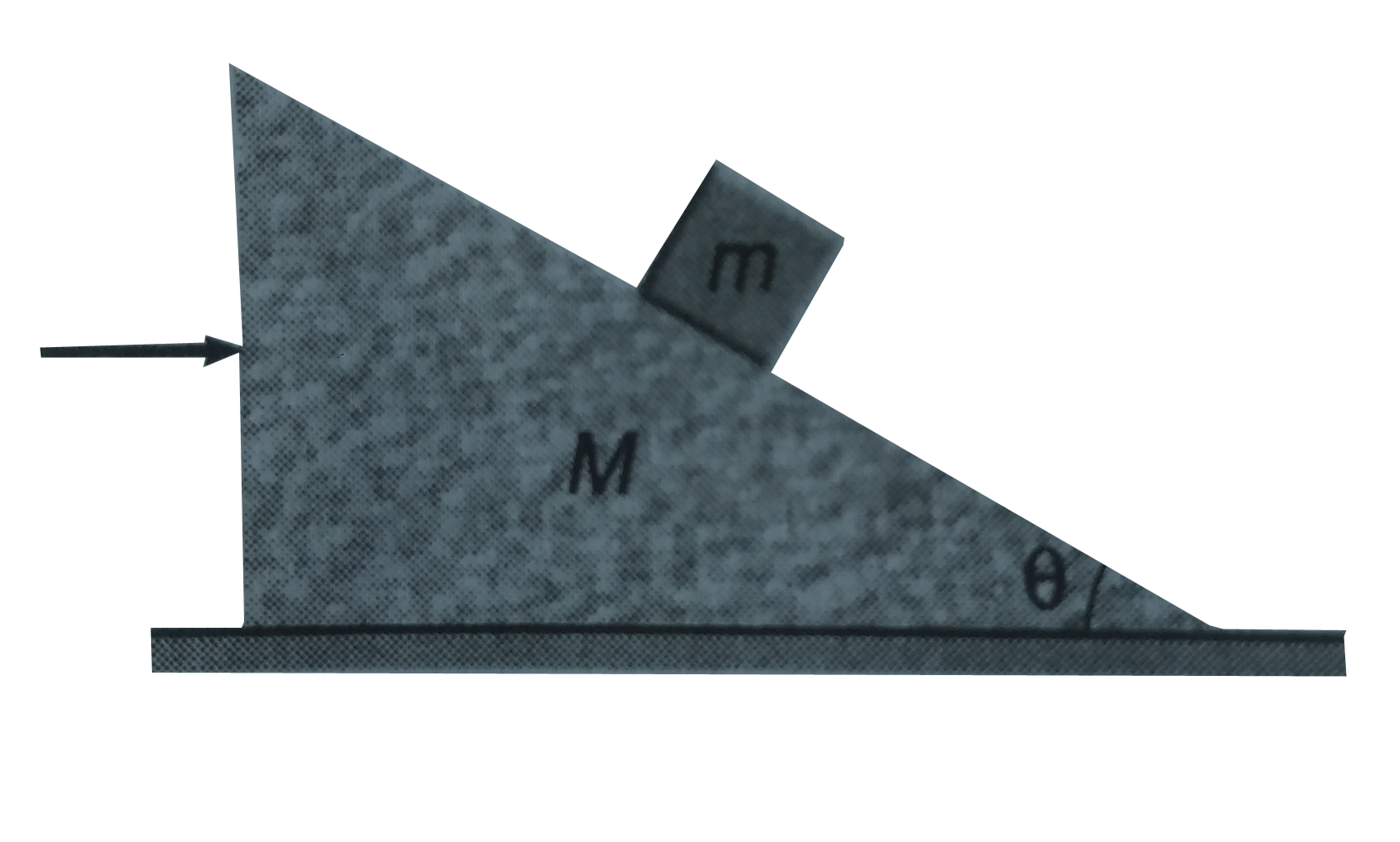
View SolutionAll surfaces are smooth in following figure. Find F, such that block remain stationary with respect to wedge.
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

View Solution
