Specific volume of cylindrical virus particle is 6.02×10−2cc/g , whose radius and length are 7Åand10Å respectively . If NA=6.023×1023 , find molecular weight of virus.
1.54 kg/mol.
1.54×104 kg/mol.
3.08×104 kg/mol.
3.08×103 kg/mol.
The correct Answer is:A
Sp. Vol (vol. of 1gm ) cylindrical virus particle =6.02×10−2 cc/gm
radius of virus r=7Å= 7×10−8 cm
length of virus =πr2l
=227×(7×10−8)2×10×10−8=154×10−23 cc
wt. of one virus particle =Vol.Sp. vol.
⇒154×10−236.02×10−2 gm
∴ mol. wt. of virus =wt. of NA particles
=154×10−236.02×10−2×6.02×10+23 gm/mol
=15400 gm/mol =15.4 kg/mol
Similar Questions
Specific volume of cylindrical virus particle is 6.02×10−2cc/g whose radius and length 7Å and 10Å respectively. If NA=6.02×1023, find molecular weight of virus:
View SolutionA sample of CxOy having 3×1019 molecules is weighing 3.4mg. Then the value of x and y is respectively …………… (TakeNA=6×1023)
View Solution
Knowledge Check
The number of atoms in 0.1 mole of a triatomic gas is (NA=6.02×1023mol−1)
A6.026×1022B1.806×1023C3.600×1023D1.800×1022The specific charge of a proton is 9.6×107C kg−1.
The specific charge of an alpha particle will beA9.6×107C kg−1B19.2×107C kg−1C4.8×107C kg−1D2.4×107C kg−1
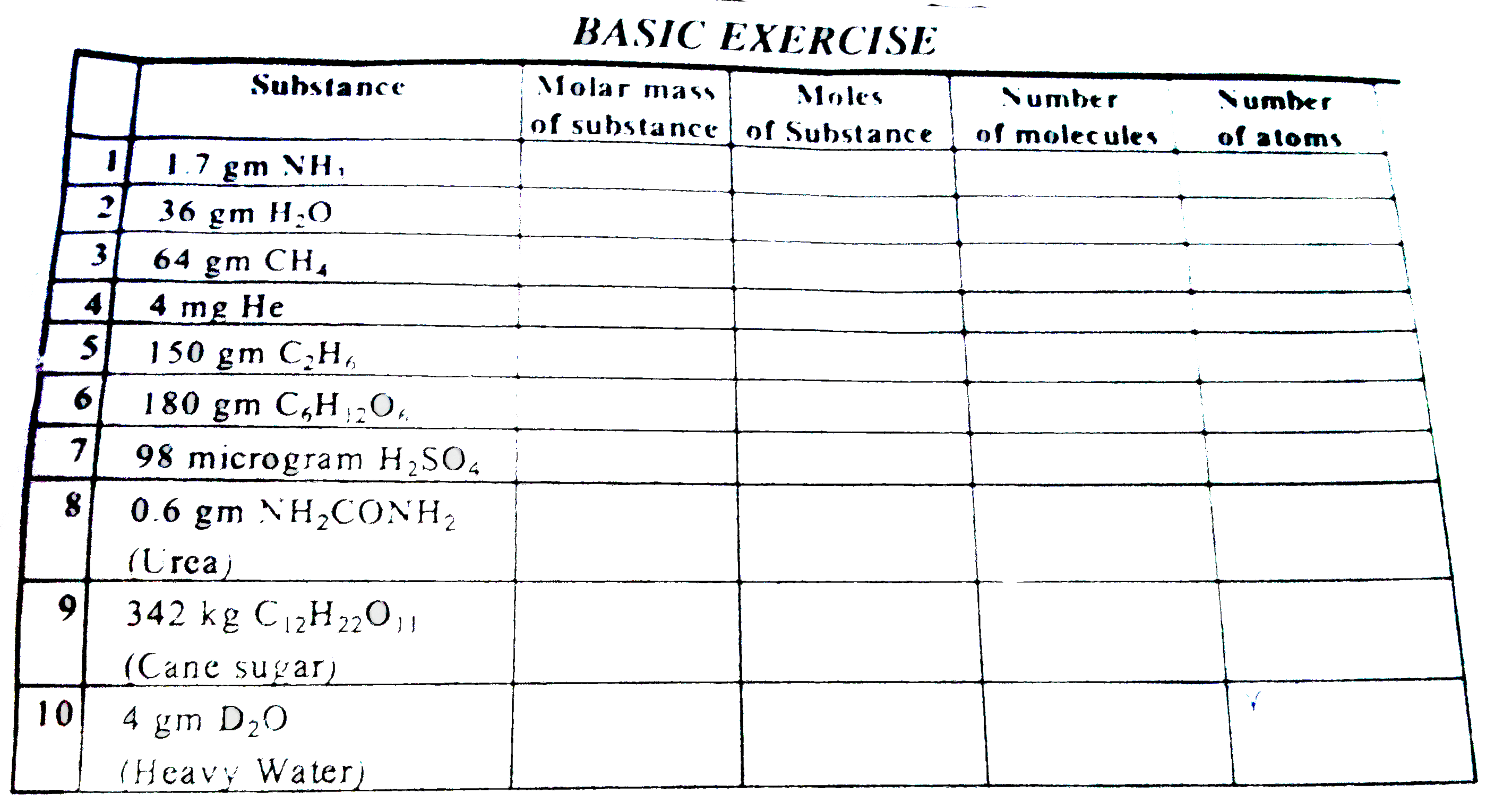
How many moles of O-atoms are in 2.7×1025 molecules of CO2(NA=6×1023)
View SolutionSilver metal crystallises with a face centred cubic lattice. The length of the unit cell is found to be 3.0×10−8cm. Calculate atomic radius and density of silver.
Molar mass of Ag=108gmol−1,NA=6.02×1023mol−1).
View SolutionThe specific charge of a proton is 9.6×107C kg−1.
The specific charge of an alpha particle will be
View SolutionThe average kinetic energy of one molecule of an ideal gas at 27∘C and 1 atm pressure is [Avogadro number NA=6.023×1023 ]
View SolutionThe number of atoms in 0.1 mol of a tetraatomic gas is (NA=6.02×1023mol−1)
View Solution8 g of O2− ion has amount of charge equal to : (NA=6.02×1023,e=−1.6×10−19C)
View Solution
