Correct gas equation is :
V1T2p1=V2T1p2
p1T1V1=p2V2T2
p1V1p2V2=T1T2
V1V2T1T2=p1p2
The correct Answer is:C
To solve the question regarding the correct gas equation, we will derive the relationship using the ideal gas law and compare the given options.
1. Understand the Ideal Gas Law:
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature.
2. Set Up the Equations for Two Gases:
For gas 1, we can write:
P1V1=nRT1
For gas 2, we can write:
P2V2=nRT2
3. Assume the Number of Moles is the Same:
We assume that the number of moles n is the same for both gases. This means we can cancel n and R when we divide the two equations.
4. Divide the Two Equations:
Dividing the equation for gas 1 by the equation for gas 2, we get:
P1V1P2V2=T1T2
5. Rearranging the Equation:
Rearranging the above equation gives us:
P1V1T2=P2V2T1
6. Identifying the Correct Expression:
Now, we need to compare this derived equation with the options provided in the question. The derived equation can be rearranged to show the relationship between the variables.
7. Conclusion:
The correct expression derived from the ideal gas law is:
P1V1P2V2=T1T2
Thus, the correct option from the given choices is Option A.
The ideal gas law is given by the equation:
PV=nRT
where P is pressure, V is volume, n is the number of moles, R is the gas constant, and T is temperature.
For gas 1, we can write:
P1V1=nRT1
For gas 2, we can write:
P2V2=nRT2
We assume that the number of moles n is the same for both gases. This means we can cancel n and R when we divide the two equations.
Dividing the equation for gas 1 by the equation for gas 2, we get:
P1V1P2V2=T1T2
Rearranging the above equation gives us:
P1V1T2=P2V2T1
Now, we need to compare this derived equation with the options provided in the question. The derived equation can be rearranged to show the relationship between the variables.
The correct expression derived from the ideal gas law is:
P1V1P2V2=T1T2
Thus, the correct option from the given choices is Option A.
Similar Questions
The perfect gas equation for 4 gm of Hydrogen gas will be
View SolutionDerive the perfect gas equation.
View SolutionWith the help of gas laws, deduce an expression for the gas equation. What is the utility of the gas equation ?
View SolutionFor two mole of an ideal gas, the correct relation is :
View SolutionCorrect equation of Freundlich isotherm is
View SolutionCorrect equation of Freundrich iostherm is
View SolutionCorrect equation for exponential growth is :
View SolutionThe correct thermochemical equation is :
View SolutionCorrect equation for photosynthesis is
View SolutionSee the electrical circuit shown in this figure. Which of the following equations is the correct equation for it ?
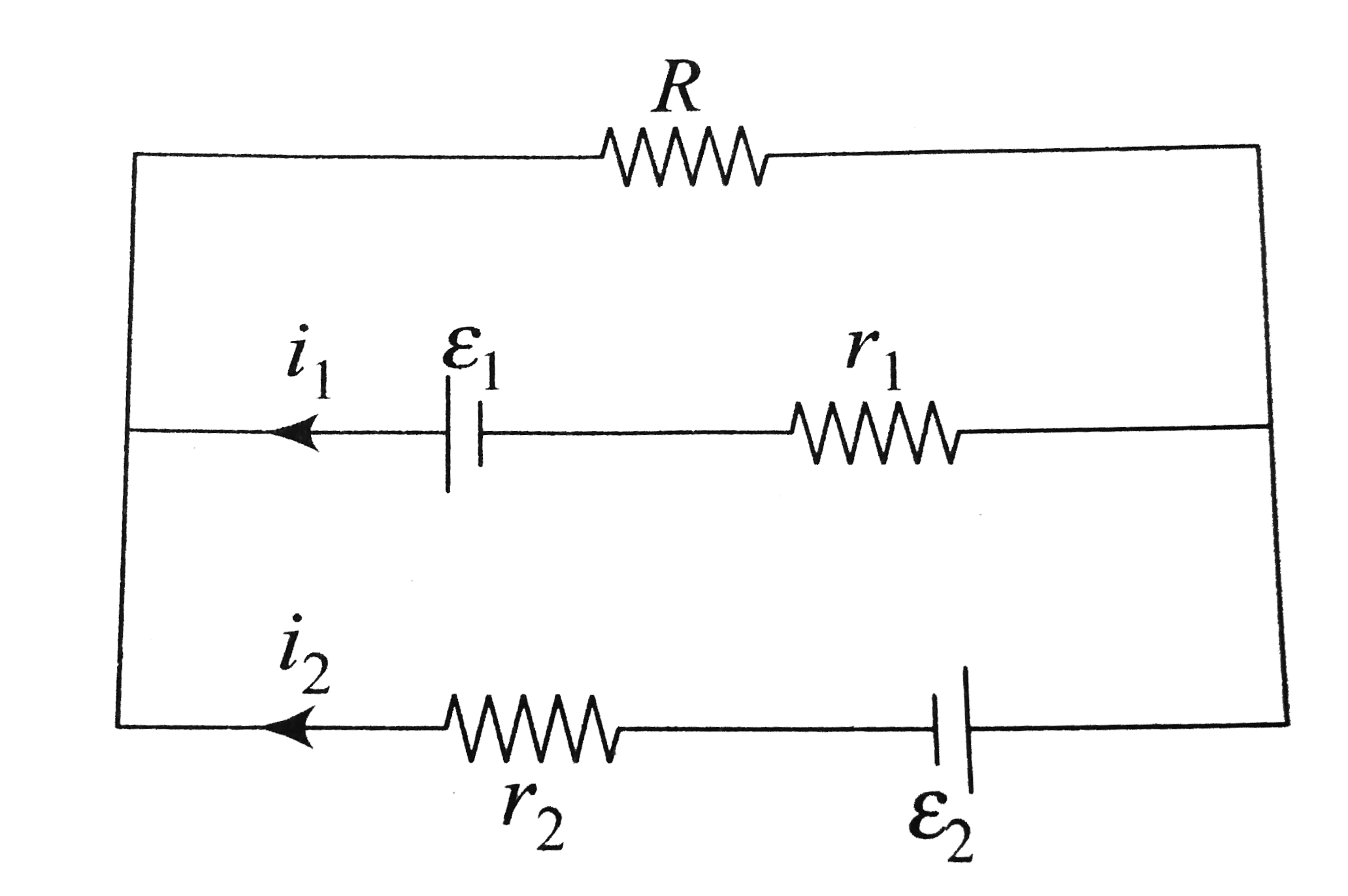
View Solution
