If ΔH is the enthalpy change and ΔU the change in internal energy accompanying a gaseous reaction, then
ΔH is always greater than ΔE
ΔH<ΔE only if the number of moles of products is greater than the number of moles of the reactants
ΔH is always less than ΔE
ΔH<ΔE only if the number of moles of products is less than the number of moles of the reactants
The correct Answer is:D
To solve the question regarding the relationship between the enthalpy change (ΔH) and the change in internal energy (ΔU) for a gaseous reaction, we can follow these steps:
Step 1: Understand the relationship between ΔH and ΔU
The relationship between the change in enthalpy (ΔH) and the change in internal energy (ΔU) for a gaseous reaction is given by the equation:
ΔH=ΔU+ΔngRT
where:
- Δn_g = change in the number of moles of gas (moles of gaseous products - moles of gaseous reactants)
- R = universal gas constant
- T = temperature in Kelvin
Step 2: Analyze the significance of Δn_g
The term Δn_g indicates whether the number of gaseous products is greater than, less than, or equal to the number of gaseous reactants:
- If Δn_g > 0: There are more moles of gas in the products than in the reactants.
- If Δn_g < 0: There are fewer moles of gas in the products than in the reactants.
- If Δn_g = 0: The number of gaseous moles remains the same.
Step 3: Determine the implications of Δn_g
1. If Δn_g > 0:
- The equation becomes:
ΔH=ΔU+(positive term)
- This implies that ΔH > ΔU.
2. If Δn_g < 0:
- The equation becomes:
ΔH=ΔU+(negative term)
- This implies that ΔH < ΔU.
Step 4: Conclusion based on the analysis
From the analysis:
- ΔH can be greater than or less than ΔU depending on the value of Δn_g.
- Thus, we cannot say that ΔH is always greater than or always less than ΔU; it depends on the reaction.
Step 5: Identify the correct statement
Based on the above analysis, the correct statement is:
- If Δn_g > 0, then ΔH > ΔU.
- If Δn_g < 0, then ΔH < ΔU.
Thus, the correct answer is Option B: ΔH is less than ΔU when the number of gaseous products is greater than the number of gaseous reactants.
The relationship between the change in enthalpy (ΔH) and the change in internal energy (ΔU) for a gaseous reaction is given by the equation:
ΔH=ΔU+ΔngRT
where:
- Δn_g = change in the number of moles of gas (moles of gaseous products - moles of gaseous reactants)
- R = universal gas constant
- T = temperature in Kelvin
The term Δn_g indicates whether the number of gaseous products is greater than, less than, or equal to the number of gaseous reactants:
- If Δn_g > 0: There are more moles of gas in the products than in the reactants.
- If Δn_g < 0: There are fewer moles of gas in the products than in the reactants.
- If Δn_g = 0: The number of gaseous moles remains the same.
1. If Δn_g > 0:
- The equation becomes:
ΔH=ΔU+(positive term)
- This implies that ΔH > ΔU.
- The equation becomes:
ΔH=ΔU+(negative term)
- This implies that ΔH < ΔU.
From the analysis:
- ΔH can be greater than or less than ΔU depending on the value of Δn_g.
- Thus, we cannot say that ΔH is always greater than or always less than ΔU; it depends on the reaction.
Based on the above analysis, the correct statement is:
- If Δn_g > 0, then ΔH > ΔU.
- If Δn_g < 0, then ΔH < ΔU.
Similar Questions
If ΔH is the change in enthylpy and ΔU, the change in internal energy accompanying a gaseous reactant then
View SolutionThe change in the internal energy of a substance
View SolutionA mixture of 2 moles of CO and 1 mole of O2, in a closed vessele is ignited to convert the CO to CO2 if ΔH is the enthalpy change and ΔU is the change in internal energy then:
View SolutionEnthalpy change equal internal energy change when
View SolutionWhy is the enthalpy considered more useful than internal energy in chemical reactions ?
View SolutionThe enthalpy change of a reaction does not depend on
View SolutionIn a reversible isothermal process, the change in internal energy is :
View SolutionChange in internal energy in an isothermal process for ideal gas is
View SolutionThe magnitude of ethalphy changes for reversible adiabatic expansion of a gas from volume V1 to V2(in L)is ΔH1 and for irreversible adiabatic expansion for the same expansion is ΔH2. Then
when ΔU1 and ΔU2 are the changes in magnitudes for the internal energy of gas in the two expansions.
View SolutionA system goes from A and B via two processes. I and II as shown in figure. If ΔU1andΔU2 are the changes in internal energies in the processes I and II respectively, then
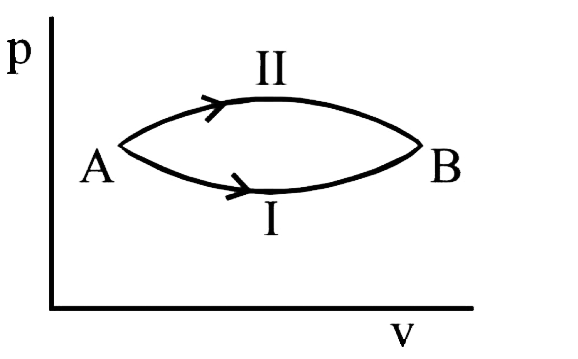
View Solution
