U235+n1→ fission product + neutron + 3.2×10−11J. The energy released , when 1g of U235 finally undergoes fission , is
(a) 12.75×108kj
(b) 18.60×109kj
(c) 8.21×107kj
(d) 6.55×106kj
The correct Answer is:C
To solve the problem of calculating the energy released when 1 gram of Uranium-235 undergoes fission, we can follow these steps:
Step 1: Understand the reaction
The fission of Uranium-235 (U-235) when it absorbs a neutron (n) releases energy. The energy released per fission event is given as 3.2×10−11J.
Step 2: Calculate the number of atoms in 1 gram of U-235
The molar mass of U-235 is approximately 235 g/mol. Using Avogadro's number (6.022×1023 atoms/mol), we can find the number of atoms in 1 gram of U-235.
Number of atoms in 1 g of U-235=6.022×1023atoms/mol235g/mol≈2.56×1021atoms
Step 3: Calculate the total energy released for 1 gram of U-235
Now, we can find the total energy released when 1 gram of U-235 undergoes fission by multiplying the energy released per fission event by the number of atoms in 1 gram.
Total energy released=Number of atoms×Energy per fission
Total energy released=2.56×1021atoms×3.2×10−11J/atom
Total energy released≈8.19×1010J
Step 4: Convert the energy from Joules to kilojoules
To convert the energy from joules to kilojoules, we divide by 1000.
Total energy released in kJ=8.19×1010J1000≈8.19×107kJ
Step 5: Conclusion
The energy released when 1 gram of U-235 undergoes fission is approximately 8.21×107kJ, which corresponds to option C.
Final Answer
The energy released when 1 gram of U-235 undergoes fission is 8.21×107kJ.
---
The fission of Uranium-235 (U-235) when it absorbs a neutron (n) releases energy. The energy released per fission event is given as 3.2×10−11J.
The molar mass of U-235 is approximately 235 g/mol. Using Avogadro's number (6.022×1023 atoms/mol), we can find the number of atoms in 1 gram of U-235.
Now, we can find the total energy released when 1 gram of U-235 undergoes fission by multiplying the energy released per fission event by the number of atoms in 1 gram.
Total energy released=2.56×1021atoms×3.2×10−11J/atom
Total energy released≈8.19×1010J
To convert the energy from joules to kilojoules, we divide by 1000.
The energy released when 1 gram of U-235 undergoes fission is approximately 8.21×107kJ, which corresponds to option C.
The energy released when 1 gram of U-235 undergoes fission is 8.21×107kJ.
Similar Questions
The .92U235 absorbs a slow neturon (thermal neutron) & undergoes a fission represented by .92U235+.0n1→.92U236→.56Ba141+36Kr92+30n1+E. Calculate:
The energy released when 1g of .92U235 undergoes complete fission in N if m=[N] then find m−25. [N] greatest integer
Given
.92U235=235.1175amu (atom),
.56Ba141=140.9577amu (atom),
.36r92=91.9263amu(atom),.0n1=1.00898amu,1amu=931MeV/C2
View SolutionIn a nuclear reactor, the number of U235 nuclei undergoing fissions per second is 4×1020. If the energy releases per fission is 250 MeV, then the total energy released in 10 h is (1eV=1.6×10−19J)
View SolutionThe energy released by fission of one U235 atom is 200 MeV. Calculate the energy released in kWh, when one gram of uranium undergoes fission.
View SolutionIn the given arrangement the block is released from the position where the spring is unstretched. The speed of the block when it has descended through 2cm is x10. Find the value of x.
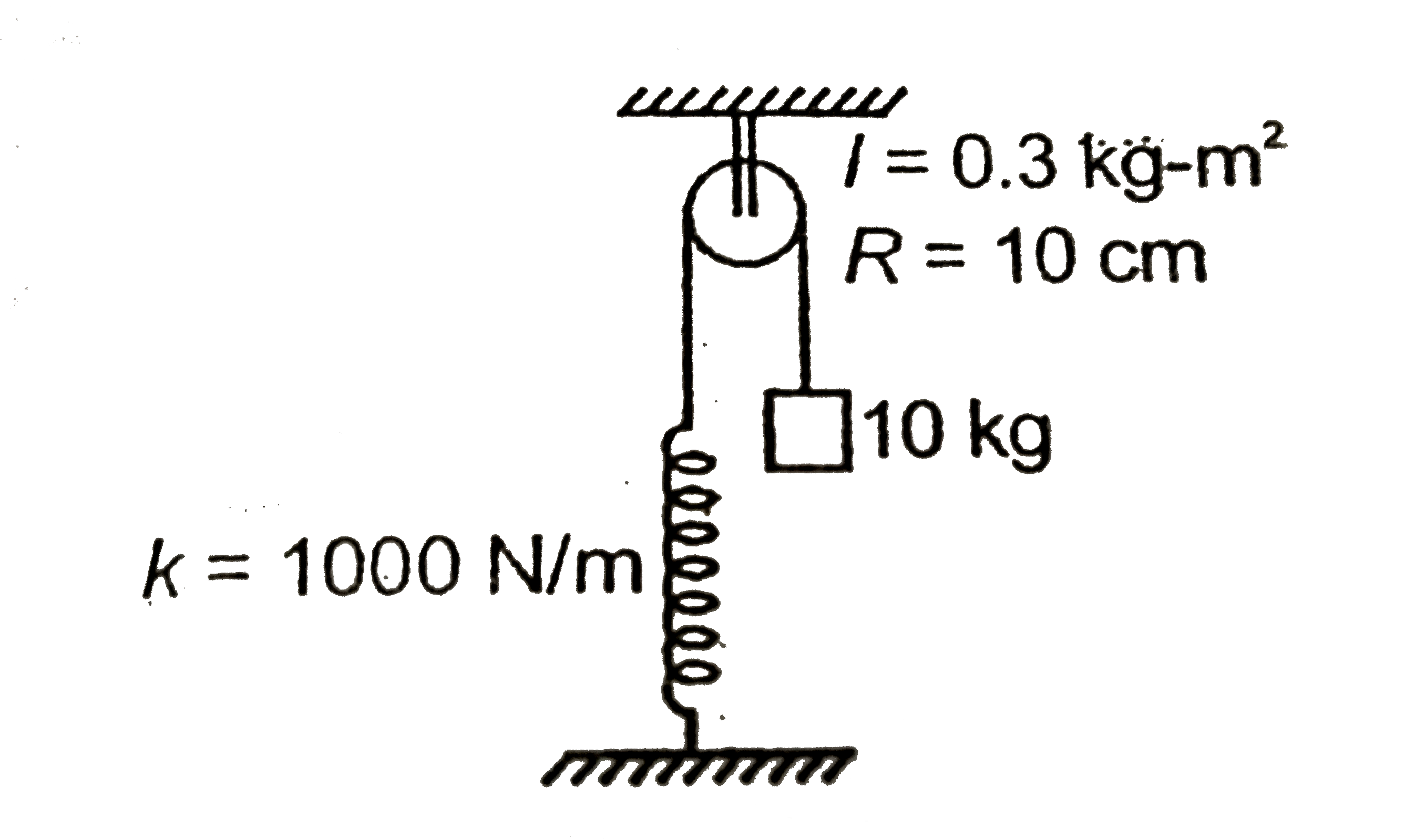
View SolutionWhat is the energy released by fassion of 1 g of U235? (Assume 200 Me V energy is liberated on fission of 1 nucleus)
View SolutionConsider the case of bombardment of U235 nucleus with a thermal neutron. The fission products are Mo95 & La139 and two neutrons. Calculate the energy released by one U235 nucleus. (Rest masses of the nuclides are U235=235.0439u,.10n=1.0087u,Mo95=94.9058u,La139.9061u).
View SolutionTwo other possible ways by which .235U can undergo fission when bombarded with a neutron are (1) by the release of .140Xe and .94Sr as fission fragments and (2) by the release of .132Sn and .101Mo as fission fragments. In each case, neutrons are also released. Find the number of neutrons released in each of these events.
View SolutionThe fission properties of .84Pu239 are very similar to those of .92U235. The average energy released per fission is 180MeV. How much energy in MeV is released if all the atoms in 1kg of pure .94Pu239 undergo fission.
View SolutionAU235 reactor generated power at a rate of P producting 2×1018 fussion per second. The energy released per fission is 185 MeV. The value of P is
View SolutionThe products of combustion of an aliphatic thiol (RSH) at 298K are
View Solution