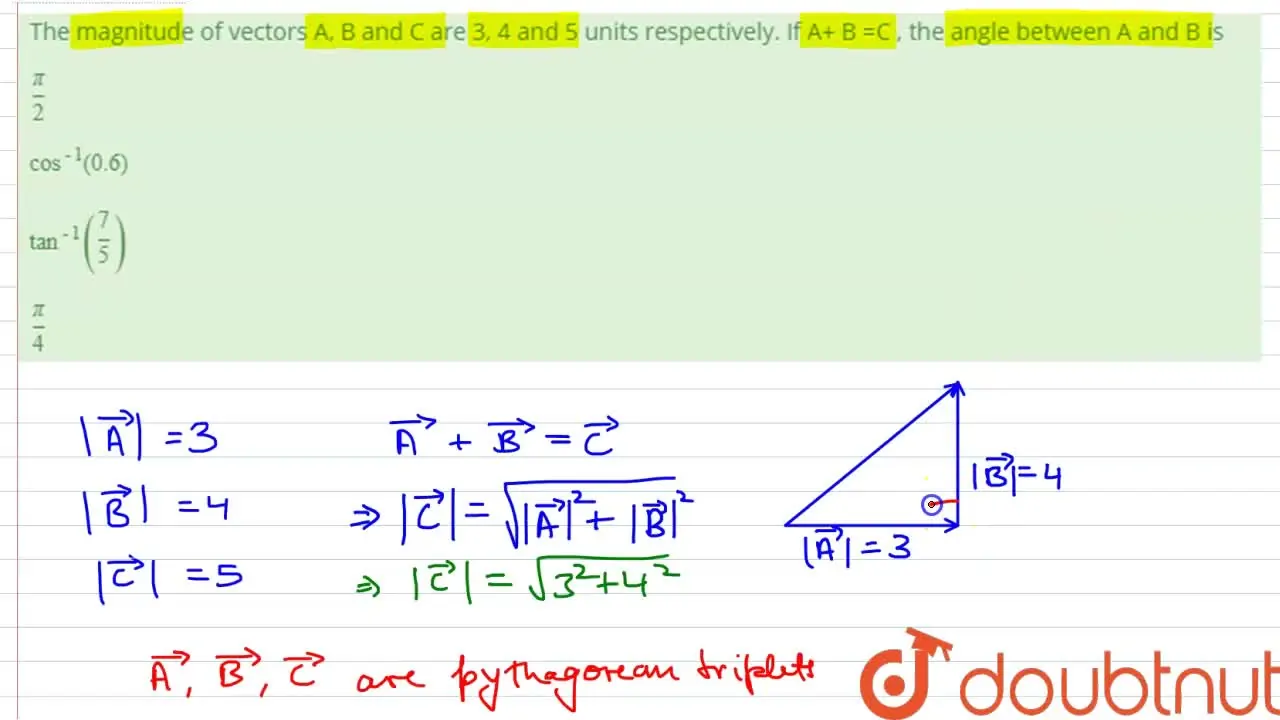
The magnitude of vectors A, B and C are 3, 4 and 5 units respectively. If A+ B =C , the angle between A and B is
The correct Answer is:a
Step by step video & image solution for The magnitude of vectors A, B and C are 3, 4 and 5 units respectively. If A+ B =C , the angle between A and B is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
The magnitudes of vectors →A,→Band→C are 3,4 and 5 units respectively. If →A+→B=→C, the angle between →A and vecB is
View SolutionThe magnitudes of vectors →A,→Band→C are 3,4 and 5 unit respectively. If →A+→B=→C, the angle between →Aand→B is
View SolutionIf →A=→B+→C, and the magnitudes of →A,→B,→C are 5,4, and 3 units, then the angle between →A and →C is
View SolutionIf →A=→B+→C and the magnitude of →A,→B and →C are 5,4 and 3 units respectively the angle between →A and →C is
View SolutionIf →A=→B+→C, and the magnitudes of →A,→B,→C are 5,4, and 3 units, then the angle between →A and →C is
View SolutionTwo vectors A and B have magnitudes 6 units and 8 units respectively. Find |A-B|, if the angle between two vectors is .(a) 0∘ (b) 180∘ (c) 180∘ (d) 120∘ .
View SolutionThe magnitude of two vectors are 16 and 12 units respectively and the magnitude of their scalar product is 98√2 units. The angle between the vectors would be
View SolutionIf A=B+C have scalar magnitudes of 5,4,3 units respectively , then find the angle between A and C.
View SolutionTwo vectors A and B have magnitudes 2 units and 4 units respectively. Find A. B is angle between these two vectors is (a) 0∘ (b) 60∘ (c) 90∘ (d) 120∘ .
View SolutionThe resultant of two vector A and B is at right angles to A and its magnitude is half of B. Find the angle between A and B.
View Solution