Two satellites of earth S1 and S2 are moving in the same orbit. The mass of S1 is four times the mass of S2. Which one of the following statements is true?
The time period of S1 is four times that of S2
The potential energies of the earth and satellite in the two cases are equal
S1 and S2 are moving with the same speed
The kinetic energies of the two satellites are equal
The correct Answer is:C
To solve the problem, we need to analyze the characteristics of two satellites S1 and S2 that are moving in the same orbit around the Earth, where the mass of S1 is four times the mass of S2.
1. Define the Masses of the Satellites:
- Let the mass of satellite S2 be m.
- Therefore, the mass of satellite S1 will be 4m.
2. Understand the Orbit:
- Both satellites are in the same orbit, which means they are at the same distance r from the center of the Earth.
3. Time Period of Satellites:
- The time period T of a satellite in a circular orbit is given by the formula:
T=2π√r3GM
where G is the gravitational constant and M is the mass of the Earth.
- Since both satellites are at the same distance r from the center of the Earth, their time periods T1 and T2 will be the same:
T1=T2
4. Speed of the Satellites:
- The speed v of a satellite in orbit is given by:
v=2πrT
- Since T1=T2 and both satellites are at the same distance r, it follows that:
v1=v2
- Thus, both satellites are moving with the same speed.
5. Kinetic Energy of the Satellites:
- The kinetic energy KE of a satellite is given by:
KE=12mv2
- For satellite S1:
KE1=12(4m)v21=2mv21
- For satellite S2:
KE2=12mv22=12mv21
- Since v1=v2, we can see that:
KE1≠KE2
- Therefore, the kinetic energies of the two satellites are not equal.
6. Potential Energy of the Satellites:
- The gravitational potential energy U between the Earth and a satellite is given by:
U=−GMmr
- For satellite S1:
U1=−GM(4m)r
- For satellite S2:
U2=−GMmr
- Clearly, U1≠U2.
Conclusion:
From the analysis, we find that:
- The time periods of both satellites are the same.
- The speeds of both satellites are the same.
- The kinetic energies of both satellites are not equal.
- The potential energies of both satellites are not equal.
The correct statement is that both satellites are moving with the same speed.
- Let the mass of satellite S2 be m.
- Therefore, the mass of satellite S1 will be 4m.
- Both satellites are in the same orbit, which means they are at the same distance r from the center of the Earth.
- The time period T of a satellite in a circular orbit is given by the formula:
T=2π√r3GM
where G is the gravitational constant and M is the mass of the Earth.
- Since both satellites are at the same distance r from the center of the Earth, their time periods T1 and T2 will be the same:
T1=T2
- The speed v of a satellite in orbit is given by:
v=2πrT
- Since T1=T2 and both satellites are at the same distance r, it follows that:
v1=v2
- Thus, both satellites are moving with the same speed.
- The kinetic energy KE of a satellite is given by:
KE=12mv2
- For satellite S1:
KE1=12(4m)v21=2mv21
- For satellite S2:
KE2=12mv22=12mv21
- Since v1=v2, we can see that:
KE1≠KE2
- Therefore, the kinetic energies of the two satellites are not equal.
- The gravitational potential energy U between the Earth and a satellite is given by:
U=−GMmr
- For satellite S1:
U1=−GM(4m)r
- For satellite S2:
U2=−GMmr
- Clearly, U1≠U2.
From the analysis, we find that:
- The time periods of both satellites are the same.
- The speeds of both satellites are the same.
- The kinetic energies of both satellites are not equal.
- The potential energies of both satellites are not equal.
Similar Questions
For an SN2 reaction which of the following statements are true:-
View SolutionA satellite is orbiting the Earth in a circular orbit of radius R. Which one of the following statement it is true?
View SolutionTwo satellites A and B move round the earth in the same orbit. The mass of B is twice the mass of A.
View SolutionFigure shows the velocity-time graph for two masses R and S that collided elastically. Which of the following statements is true?
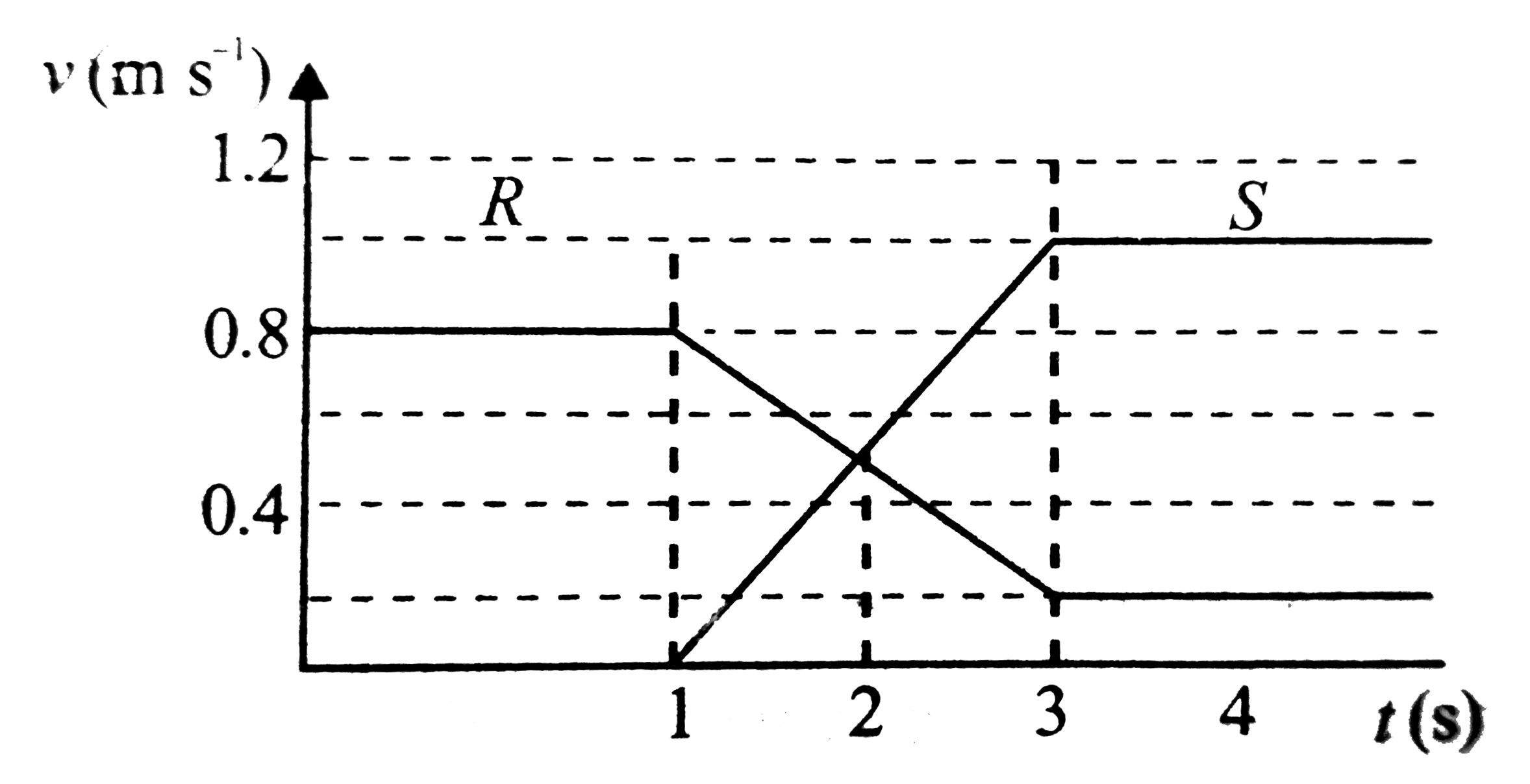
i. R and S moved in the same direction after the collision.
ii. The velocities of R and S were equal at the mid time of the collision.
iii. The mass of R was greater than mass of S.
Which of the following is true?
View SolutionThe rate of disapperance of Q in the reaction 2P+Q→2R+3S is 2×10−2 moles l−1s−1. Which of the following statement is not true?
View SolutionTwo satellites of masses of m1 and m2(m1>m2) are revolving round the earth in circular orbits of radius r1 and r2(r1>r2) respectively. Which of the following statements is true regarding their speeds v1 and v2?
View SolutionTwo billiard balls A and B each of mass 50 g and moving in opposite directions with speed of 5m/s each, collide and rebound with the same speed if the collision lasts for 10−3 s which of the following statement(s) is (are) true?
View SolutionWhich of the following is not true for SN1 reaction ?
View SolutionWhich of the following is not true for SN1 reaction ?
View SolutionFor the reaction Ag2O(s)→2Ag(s)+1/2O2(g), which one of the following is true :
View Solution
