A wind with speed 40m/s blows parallel to the roof of a house. The area of the roof is 250m2. Assuming that the pressure inside the house is atmospheric pressure, the force exerted by the wind on the roof and the direction of the force will be (Pair=1.2kg/m3)
4.8×105N, downwards
4.8×105N, upwards
2.4×105N, upwards
2.4×105N, downwards
The correct Answer is:c
To solve the problem, we need to calculate the force exerted by the wind on the roof of the house using Bernoulli's principle. Here’s a step-by-step breakdown of the solution:
Step 1: Calculate the dynamic pressure exerted by the wind.
According to Bernoulli's principle, the dynamic pressure (P) exerted by the wind can be calculated using the formula:
P=12ρv2
Where:
- ρ is the density of air (given as 1.2kg/m3)
- v is the speed of the wind (given as 40m/s)
Substituting the values:
P=12×1.2kg/m3×(40m/s)2
Calculating this:
P=12×1.2×1600=1.2×16002=960N/m2
Step 2: Calculate the force exerted on the roof.
The force (F) exerted by the wind on the roof can be calculated using the formula:
F=P×A
Where:
- A is the area of the roof (given as 250m2)
Substituting the values:
F=960N/m2×250m2
Calculating this:
F=240000N=2.4×105N
Step 3: Determine the direction of the force.
The wind creates a pressure difference. The pressure inside the house is atmospheric pressure, while the pressure exerted by the wind on the roof is lower due to the wind speed. Therefore, the force exerted by the wind on the roof will act upwards, as the wind moves from an area of higher pressure (outside) to an area of lower pressure (inside the house).
Final Answer:
The force exerted by the wind on the roof is 2.4×105N upwards.
---
According to Bernoulli's principle, the dynamic pressure (P) exerted by the wind can be calculated using the formula:
- ρ is the density of air (given as 1.2kg/m3)
- v is the speed of the wind (given as 40m/s)
The force (F) exerted by the wind on the roof can be calculated using the formula:
- A is the area of the roof (given as 250m2)
The wind creates a pressure difference. The pressure inside the house is atmospheric pressure, while the pressure exerted by the wind on the roof is lower due to the wind speed. Therefore, the force exerted by the wind on the roof will act upwards, as the wind moves from an area of higher pressure (outside) to an area of lower pressure (inside the house).
The force exerted by the wind on the roof is 2.4×105N upwards.
Similar Questions
High speed wind blows over a house. The force on the roof is
View SolutionA force of 1000 N acts on an area of 0.050m2. Find the pressure in pascals.
View SolutionA force of 200 N acts on an area of 0.16 m2 What is the magnitude of pressure in pascal?
View SolutionA heavy particle of mass 0.50 kg is hanging from a string fixed with the roof. Find the force exerted by the string on the particle. Take g=9.8ms2
 .
.
View SolutionWind with a velocity 100kmh−1 blows normally against one of the walls of a house with an area of 108m2. Calculate the force exerted on the wall if the air moves parallel to the wall after striking it and has a density of 1.2kgm−3.
View SolutionIf the force exerted by an electric dipole on a charge q at distance of 1 m is F, the force at a point 2 m away in the same direction will be
View SolutionCalculate the pressure produced by a force of 800 N acting on an area of 2m2
View SolutionAtmospheric pressure is nearly 100kPa. How large the force does the air in a room exert on the inside of a window pan that is 40cm×80cm?
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
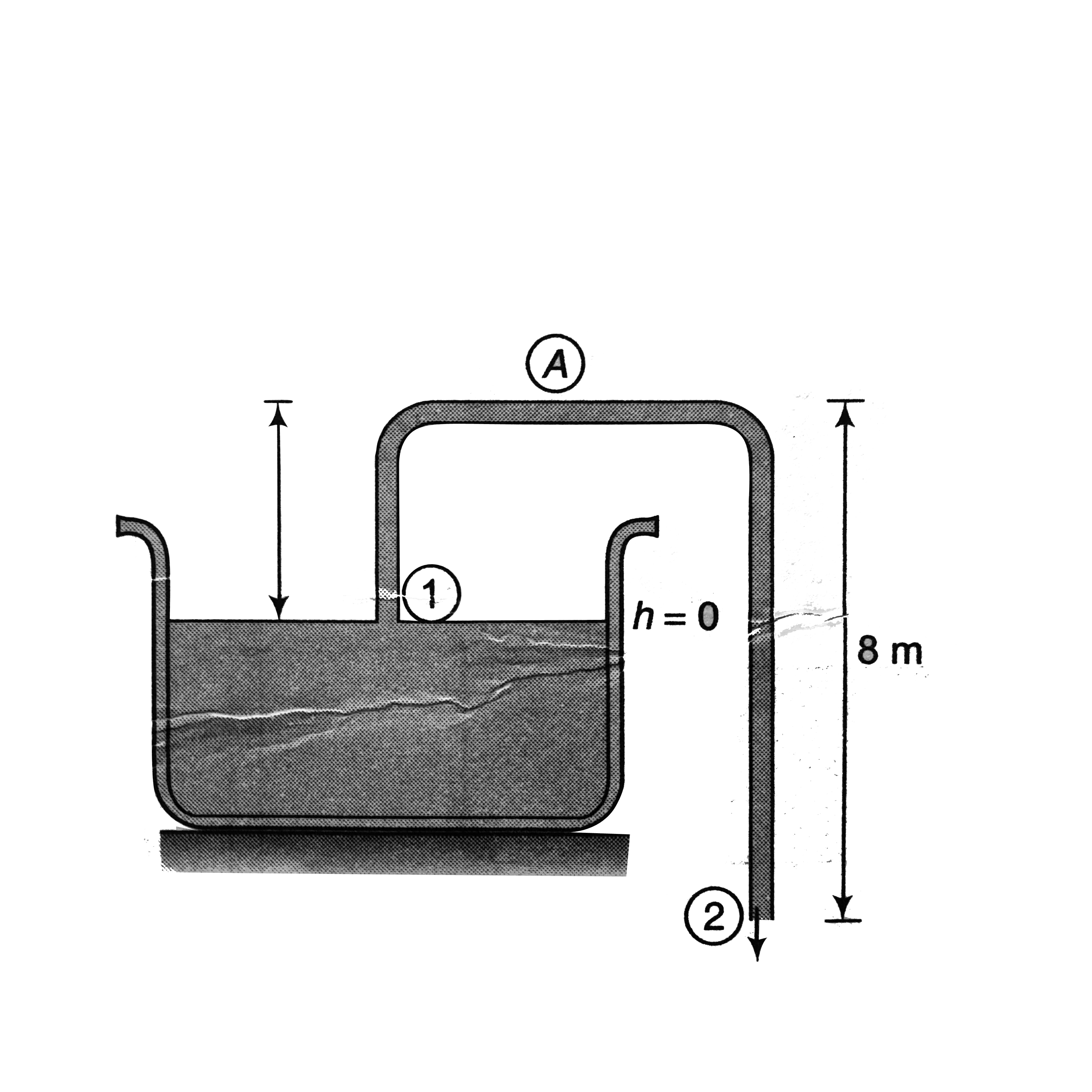 .
.
View SolutionA fighter plane is pulling out for a dive at a speed of 900km/h. Assuming its path to be a vertical circle of radius 2000m and its mass to be 16000kg, find the force exerted by the air on it at the lowest point. Take g=9.8m/s2
View Solution
