Dimension of resistance in an elecatrical circuit, in terms of dimension of mass M, of length L, of time T, and of current I, would be
[ML2T−3l−1]
[ML2T−2]
[ML2T−1l−1]
[ML2T−3l−2]
The correct Answer is:D
To find the dimension of resistance in an electrical circuit in terms of mass (M), length (L), time (T), and current (I), we can follow these steps:
Step 1: Understand the formula for resistance
Resistance (R) is defined as the ratio of voltage (V) to current (I):
R=VI
Step 2: Express voltage in terms of work done and charge
Voltage (V) can be defined as work done (W) per unit charge (Q):
V=WQ
Step 3: Substitute the expression for voltage into the resistance formula
Substituting the expression for voltage into the resistance formula gives:
R=W/QI
This can be rearranged to:
R=WQ⋅I
Step 4: Write the dimensions of work and charge
1. Work (W) has the dimension of energy, which is given by:
W=Force×Distance=(M⋅L⋅T−2)⋅L=ML2T−2
So, the dimension of work is:
(W)=M1L2T−2
2. Charge (Q) can be expressed in terms of current (I) and time (T):
Q=I⋅T
Thus, the dimension of charge is:
(Q)=I1T1
Step 5: Substitute the dimensions into the resistance formula
Now we can substitute the dimensions of work and charge into the resistance formula:
R=M1L2T−2I1T1⋅I1
This simplifies to:
R=M1L2T−2I2T1
Step 6: Simplify the expression
Now, we can simplify the expression:
R=M1L2T−2⋅T−1⋅I−2
R=M1L2T−3I−2
Final Result
Thus, the dimension of resistance (R) in terms of mass (M), length (L), time (T), and current (I) is:
(R)=M1L2T−3I−2
Resistance (R) is defined as the ratio of voltage (V) to current (I):
R=VI
Voltage (V) can be defined as work done (W) per unit charge (Q):
V=WQ
Substituting the expression for voltage into the resistance formula gives:
R=W/QI
This can be rearranged to:
R=WQ⋅I
1. Work (W) has the dimension of energy, which is given by:
W=Force×Distance=(M⋅L⋅T−2)⋅L=ML2T−2
So, the dimension of work is:
(W)=M1L2T−2
Q=I⋅T
Thus, the dimension of charge is:
(Q)=I1T1
Now we can substitute the dimensions of work and charge into the resistance formula:
R=M1L2T−2I1T1⋅I1
This simplifies to:
R=M1L2T−2I2T1
Now, we can simplify the expression:
R=M1L2T−2⋅T−1⋅I−2
R=M1L2T−3I−2
Thus, the dimension of resistance (R) in terms of mass (M), length (L), time (T), and current (I) is:
(R)=M1L2T−3I−2
Similar Questions
Dimension of resistance R in terms of μ0&ε0 are
View SolutionDimension of resistance R in terms of μ0&ε0 are
View SolutionIf the velocity (V) , acceleration (A) , and force (F) are taken as fundamental quantities instead of mass (M) , length (L),andtime(T) , the dimensions of young's modulus (Y) would be
View SolutionIf force, length and time are taken as fundamental units, then the dimensions of mass will be
View SolutionIf velocity,time and force were chosen as basic quantities, find the dimensions of mass.
View SolutionIf LandR denote inductance and resistance , respectively , then the dimensions of L/R are
View SolutionThe dimensions of resistivity in terms of M, L, T and Q, where Q stands for the dimensions of charge is
View SolutionMatch the physical quantities given in column I with dimensions expressed interms of mass (M), length (L), time (T) and charge (Q) given in column II and wire the correct answer against the match quantity in labourar form.
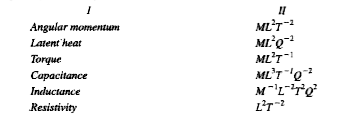
View SolutionIf force, acceleration and time are taken as fundamental quantities, then the dimensions of length will be:
View SolutionThe dimension of magnetic field in M, L, T and C (Coulomb) is given as :
View Solution
