A piece of wood of uniform cross section and height 15 cm floats vertically with its height 10 cm in water and 12 cm in spirit. Find the density of (i) wood and (ii) spirit
Cross section of the piece of wood= A cm2
Volume of the wood = 15 A cm3
Density of wood = ρWOODg cm−3
Mass of wood = 15AρWOODg
Weight of wood = 15AρWOODgf
Volume of wood under water = 10A cm3
Density of water = ρWATERg cm−3
Mass of displaced water = 10 A ρWATERg (where ρWATER = Density of water)
Weight of displaced water = 10AρWATERgf
So, upthrust = 10AρWATERgf
According to the law of flotation,
Weight of the wood = Upthrust
∴15AρWOOD=10AρWATER
Or, 15AρWOOD=10A (putting , ρWATER=1g cm−3)
Or, 15ρWOOD=10
Or, ρWOOD = 10/15
∴ρWOOD=0.667g cm−3
Volume of wood under spirit = 12Acm3
Mass of displaced spirit = 12AρWATERg (where ρSPIRIT = Density of spirit)
Weight of displaced spirit = 12AρSPIRITgf
So, upthrust = 12AρSPIRITgf
According to the law of flotation,
Weight of the wood = Upthrust
∴15AρWOOD=12AρSPIRIT
Or, 15 x 0.667 = 12ρSPIRIT (Putting , ρWOOD = 0.667 g cm−3)
Or , ρSPIRIT = 15 x 0.667/12
∴ρSPIRIT=0.83g cm−3
Topper's Solved these Questions
- FLUIDSBOOK - ICSECHAPTER - FLUIDSEXERCISE - TOPIC 2 (3 Marks Questions )
23 Videos
- ELECTRICITY AND MAGNETISM BOOK - ICSECHAPTER - ELECTRICITY AND MAGNETISM EXERCISE - TOPIC 2 Magnetism (4 Marks Questions )
6 Videos
- HEAT AND ENERGYBOOK - ICSECHAPTER - HEAT AND ENERGYEXERCISE - EXERCISE -6(D) Multiple Choice Question)
4 Videos
Similar Questions
Water rises to a height of 16.3 cm in a capillary of height 18 cm above the water leve. If the tube is cut at a height of 12 cm -
View SolutionA piece of wood floats in water with two-thirds of its volume submerged in water. In a certain oil it has 0.95 of its volume submerged. What is the density of wood and the oll?
View Solution
Knowledge Check
A U tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are at the same level with 10 cm of water in one arm and 12.5 cm of spirit in the other as shown in figure. The relative density of the spirit is
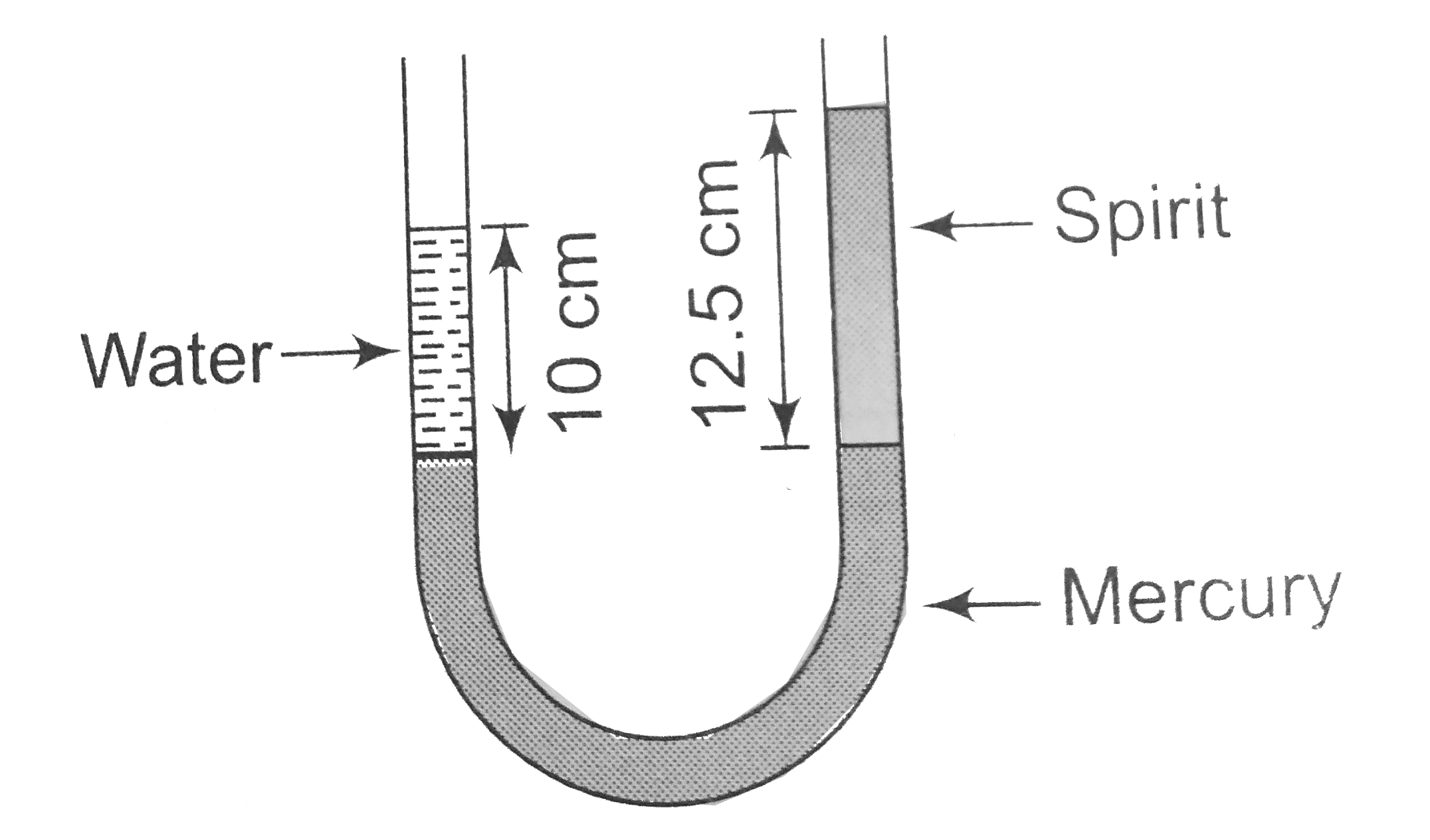 A0.6B0.8C1D1.25
A0.6B0.8C1D1.25
A body of uniform cross-sectional area floats in a liquid of density thrice its value. The portion of exposed height will be :
View SolutionThe radius of a cone is 5cm and vertical height is 12cm. Find the area of the curved surface.
View SolutionFind the area of a triangle with base 15 cm and height 8 cm.
View SolutionFind the area of a triangle :
whose height is 6 cm and base is 10 cm.
View SolutionA U tube contains water and methylated spirts separated by mercury columns in the two arms are in level with 10.0cm of water in one arm and 12.5 cm of spirit in the other. What is the relative density of spirit?
View SolutionA u tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are at the same levels with 10 cm of water in one arm and 12.5 cm of spirit on the other arm as shown. If 15 cm of water and spirit each are further poured into the respective arms of the tube. Difference in the level of mercury in the two arms is (Take, relative density of mercury = 13.6, relative density of spirit=0.8)
View SolutionA U tube contains water and methylated spirit separated by mercury. The mercury columns in the two arms are at the same level with 10 cm of water in one arm and 12.5 cm of spirit in the other as shown in figure. The relative density of the spirit is

View Solution
