The incident ray and the reflected ray from a mirror are mutually perpendicular to each other.
Find the angle of incidence.
To solve the problem where the incident ray and the reflected ray from a mirror are mutually perpendicular to each other, we will follow these steps:
1. Understand the Problem: We know that the incident ray and the reflected ray are mutually perpendicular. This means that the angle between these two rays is 90 degrees.
2. Define Angles:
- Let the angle of incidence be denoted as I.
- The angle of reflection, denoted as R, is equal to the angle of incidence according to the law of reflection, which states that I=R.
3. Set Up the Equation: Since the incident ray and the reflected ray are perpendicular, we can express this relationship mathematically:
I+R=90∘
4. Substitute the Angle of Reflection: Since R=I, we can substitute R in the equation:
I+I=90∘
This simplifies to:
2I=90∘
5. Solve for the Angle of Incidence: To find the angle of incidence I, divide both sides of the equation by 2:
I=90∘2=45∘
6. Conclusion: The angle of incidence is 45∘.
Final Answer:
The angle of incidence is 45∘.
---
- Let the angle of incidence be denoted as I.
- The angle of reflection, denoted as R, is equal to the angle of incidence according to the law of reflection, which states that I=R.
I+R=90∘
I+I=90∘
This simplifies to:
2I=90∘
I=90∘2=45∘
The angle of incidence is 45∘.
Topper's Solved these Questions
- LIGHTBOOK - ICSECHAPTER - LIGHTEXERCISE - TOPIC 1 Reflections of Light (3 Marks Questions )
19 Videos
- LIGHTBOOK - ICSECHAPTER - LIGHTEXERCISE - TOPIC 1 Reflections of Light (4 Marks Questions )
10 Videos
- LAWS OF MOTIONBOOK - ICSECHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE - 3(E) (Numericals)
31 Videos
- MAGNETISM BOOK - ICSECHAPTER - MAGNETISM EXERCISE - EXERCISE-10(B) (Multiple Choice Type)
2 Videos
Similar Questions
A ray of light falls on a transparent glass slab of refractive index √2. If the reflected and refracted rays are mutually perpendicular, then the angle of incidence is
View SolutionA ray of light is incident on a transparent glass slab of refractive index √3. If the reflected and refracted rays are mutually perpendicular, what is the angle of incidence?

View SolutionA light ray is travelling from air to glass. True reflected and refracted rays are perpendicular to each other. If the angle of incidence in air is I the refractive index of glass is
View SolutionA ray is incident on a plane mirro. Its reflected ray is perpendicular to the incident ray. Find the angle of incidence.
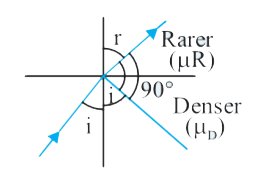
View SolutionA ray of light from a denser medium strikes a rarer medium at an angle of incidence i=tan−1(1/2). If the reflected and the refracted rays are mutually perpendicular to each-other, what is the value of the critical angle in degree?
View SolutionThe incident ray, the reflected ray and the normal lie............
View SolutionStatement I: A light ray is incident on a glass slab. Some portion of it is reflected and some is refracted. Refracted and reflected rays are always perpendicular to each other.
Statement II: Angle of incidence is equal to angle of reflection.
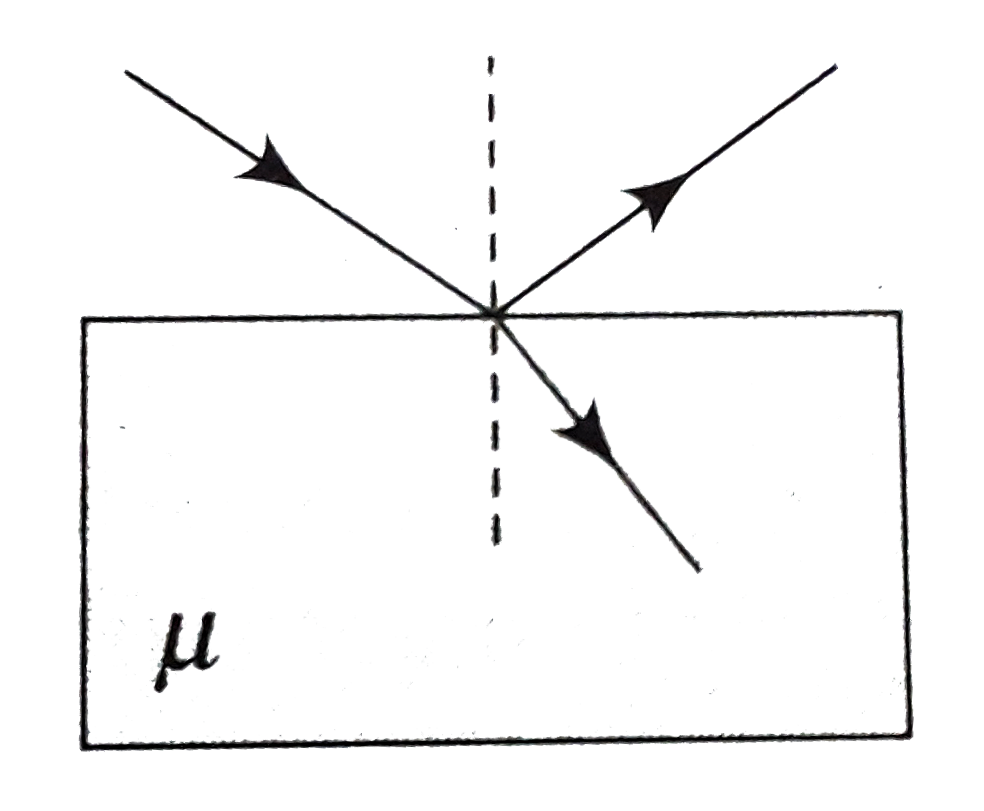
View SolutionDraw the incident ray and the reflected ray. if a light ray is incident on a plane mirror with the angle of incidence 30∘.
View SolutionUnder what condition in an arrangement of two plane mirrors, incident ray and reflected ray will always be parallel to each other, whatever may be angle of incidence. Show the same with the help of diagram.
View SolutionA ray of light is incident at an angle i from denser to rare medium. The reflected and the refracted rays are mutually perpendicular. The angle of reflection and the angle of refraction are respectively r and r'. Then the critical angle will be:
View Solution
